
 | Computer Modeling in Engineering & Sciences |  |
DOI: 10.32604/cmes.2021.016882
REVIEW
Damage Assessment of Reinforced Concrete Structures through Damage Indices: A State-of-the-Art Review
College of Civil Engineering, Tongji University, Shanghai, 200092, China
*Corresponding Author: Xiaodan Ren. Email: rxdtj@tongji.edu.cn
Received: 06 April 2021; Accepted: 11 May 2021
Abstract: Due to the developments of computer science and technology in recent years, computer models and numerical simulations for large and complicated structures can be done. Among the vast information and results obtained from the analysis and simulations, the damage performance is of great importance since this damage might cause enormous losses for society and humanity, notably in cases of severe damage occurring. One of the most effective tools to handle the results about the damage performance of the structure is the damage index (DI) together with the damage states, which are used to correlate the damage indices with the damage that occurred in the actual structures. Numbers of damage indices proposed and developed rely on the fact that the damage causes noticeable changes in the structural and dynamic properties of the structural components or the whole structure. Therefore, this study presents a comprehensive review of the damage assessment of Reinforced Concrete (RC) structures. It presents step by step the development of the damage indices that are most widely used to estimate the performance of structural components in the structure and subsequently assess the damage degree of such these structures either based on the structural properties or dynamic properties of the structure. Also, several damage states have been introduced to estimate the performance level of the structure. Finally, case studies, methodologies, and applications on the damage assessment of RC structures are reviewed and presented.
Keywords: Structure properties-based damage indices; dynamic properties-based damage indices; damage states
Microcracks are initiated in the reinforced concrete elements due to shrinkage, hydration, etc., even if they have not been subjected to an external load. Subsequently, these microcracks propagated and led to macrocracks formulation during the structure life cycle due to various types of external loads and various types of structural shortcomings such as cracking, buckling, yielding of steel reinforcement, crushing of concrete. Structural damage and collapse might occur due to various types of external loads and, according to different structural faults, which might cause enormous losses for society and humanity, see Fig. 1. Therefore, structures must be accurately evaluated for structural safety [1]. Damage assessment of the structures is significantly needed in performance-based structural analysis.
The structural damage can be defined as the degradation degree, which represents the structure capacity resisting and withstand further loadings since the failure of the structures might cause considerable losses. Therefore, performing the damage assessment and determining the structural damage degree became the main challenge for structural analysts. As a subsequent step for the damage assessment, the structural analysts can estimate the maximum loading capacity and the structure’s remaining capacity before reaching the failure limit. Accordingly, the structure safety can be assessed. Many researchers have studied the damage and safety performance of different RC structures from various perspectives [2–5].

Figure 1: Structural damage and collapse during Wenchuan Earthquake, Sichuan Province, China, 2008 [6] (a) Collapsed four-story Xuankou Middle School in Yinxiu (b) The total collapse of buildings at Xiaoyudong due to Surface rupture
The damage progression index has been widely used to investigate the performance of structural elements and assess the degree of damage for the structure. In seismic regions, damage indices have a fundamental role in decision-making about retrofit and maintenance [7]. Elenas et al. [8] mentioned that parameters that can reflect and represent the structural damage had attracted many researchers’ attention among several parameters of the structural response. Consequently, their studies have mainly focused on these parameters. It is also worthy to note that damage indices have many other applications, such as they can contribute to selecting earthquake records for structural design [9] and mapping the spatial distribution of the damage potential of recorded ground motions [10].
Damage index at the structural level has been defined according to the main characteristic associated with the structure. The classification of the damage index has been done in four groups based on resistance demands (in the linear and nonlinear stages), ductility requirements, energy dissipation, and finally, the reduction of stiffness. It has been suggested by Krawinkler et al. [11] to estimate the structural behavior against a seismic hazard by evaluating the damage indices of the nonlinear models. The need for such damage indices as an effective estimation tool has been appeared and developed since the 1970s, mainly in earthquake engineering, in whose several formulae for estimating the damage degree of beam-column structures have been proposed and developed [12,13].
The damage index based on mathematical functions, which depend accordingly on many structural parameters that determine the structural damage, has ranged from 0 to 1. Zero indicates that there is no damage in the structure and the structural behavior remains in the elastic stage. In contrast, the unit value of the damage index represents the failure state or collapse of the structure [14]. The force-based approach and the deformation-based approach [15] have been adopted in the current seismic design codes to indicate the damage performance. However, this method does not take into consideration the accumulative damage.
Significant and considerable efforts have been presented to describe the damage level of specific structures through damage indices which can assess and quantify the damage degree. For instance, Powell et al. [16] mentioned that damage assessment and evaluation of a structure could be investigated with several damage indices (DIs), accurately reflecting the amount and the degree of the damage. Also, the structural damage index could be calculated and determined through several ways, such as demand and capacity of the structure, balancing, or degradation of some structural property. Reinhorn et al. [17] mentioned that demand of ground motion and structure capacity are the main factors in evaluating the structural damage index. This evaluation contains two sources of damage, including cyclic loading and permanent deformation under the earthquake effect.
Two different methodologies have been proposed and introduced to provide a reliable prediction for the damaged structure state. The first approach is based on the structural response and the change in the structural properties when the structure is subjected to a particular loading condition. In the case of reinforced concrete, several structural response parameters can numerically represent the degree of structural damage, including structure’s drift, displacement, strain, plastic dissipated energy, or a combination of these [18]. The second category of damage indices has been developed according to the concept, the structural element’s degradation changes the dynamic response of the structure, including fundamental frequencies, damping ratios, and mode of shape. Hence, Modal analysis also reveals information about the damage of the structures according to the variation in dynamic characteristics.
Kappos [19] has presented a detailed classification for the damage indices according to response criteria and parameters affected, formulation, and the use or capability of these damage indices. According to this classification, the damage indices have been divided into local damage indices and global damage indices used to assess the damage that occurred in individual elements or the whole structure. The local damage indices can also be divided into two categories: cumulative and non-cumulative [20].
The following sections introduce the alternative methods for implementing these approaches and the formulae of different damage indices. Section 2 presents the damage assessment based on structural properties, where Section 3 presents the damage assessment based on modal analysis, Appendix 1.
2 Structure Properties-Based Damage Indices
The classification of damage indices based on structure properties has been introduced by several researchers [21–23]. In general, damage indices have been divided into two kinds of damage indices, including local damage indices and global damage indices.
The local damage indices have been divided and classified as follows:
• Cumulative damage index in case of cyclic loading.
• Non-cumulative damage index if no-cyclic loading exists.
2.1.1 Cumulative Damage Index Accounting for Cyclic Load
These sets of damage indices depend on the number of loading and unloading cycles. It has been generally concluded that the degree of the damage for a structural element is not only dependent on the maximum displacement recorded under the earthquake effect but also on the number of load cycles and hysteretic energy absorbed [18,24–26].
(1) Displacement-based cumulative indices
Banon et al. [18] developed the normalized cumulative rotation (NCR) damage index based on the ductility-based methodology to consider the cyclic loading effect if it exists. This damage index has been developed based on the low-cycle fatigue law, reflecting the damage accumulated due to cyclic loading, such as the damage that occurred due to seismic hazard. The low-cycle fatigue index (
Here
Miner [27] has proposed the low-cycle fatigue index DF, and it has been expressed as follows:
Here
(2) Hysteretic energy-based cumulative indices
Plastic dissipated energy is considered one of several structural response parameters, which can numerically reflect the damage degree of the structure. Therefore, it has been used to define energy-based damage indices. The energy dissipated by the structure is supposed to be less than or equal to a threshold value before the structure reaches the collapse limit. Gosain et al. [28] have proposed a formula for an energy-based damage index based on the maximum loading capacity of the structure (the failure load), the yielding force, the maximum and yield displacement of the structure, and this damage index has been expressed as:
where
Hwang et al. [29] also proposed another formula based on the dissipated energy to assess the structural damage degree through the damage index ID, and this damage index has been presented as follows:
Here
(3) Combined cumulative indices
The stated damage indices in the previous sections have been developed and expressed based on the deformation or the energy dissipated individually depending on the structure response. However, the displacement index or displacement ductility index cannot give a reliable description for damage performance and the dynamic response of structures [30,31]. In this section, the damage indices have been developed based on the change in the displacement and the energy dissipated together, and they have been expressed as the summation of the two quantities, called combined cumulative damage indices. In this regard, Park et al. [32,33] proposed the well-known and most widely used Park–Ang damage index
Here
where:
Cosenza et al. [34] stated that the value of

Figure 2: Modeling of building [35] (a) Structural modeling (b) Force-displacement relation
Kunnath et al. [44,45] developed the Park–Ang damage index by replacing the deformation with curvature in the first term using the moment-curvature concept instead of load-displacement. The developed damage index has been represented as follows:
Here
According to the basic concept and original definition of the damage index, the damage index values range from 0 to 1.0. Therefore, the value of the damage index is supposed to be equal to 1.0 at the maximum deformation limit
Here
Another modification has been presented for the Park–Ang damage index regarding the elastic response. However, the value of the damage index is supposed to be zero in the elastic response indicating no damage occurred; the value of the original formula of the Park–Ang damage index or its modifications seems to be more than zero. To overcome this drawback, Bozorgnia et al.{[46,47]} have presented two modified formulae for the damage index, in which the sum of the weight factors of deformation and energy dissipation is equal to 1.0; the two modified damage indices have been presented as follows:
Here μ refers to the displacement ductility ratio
Kunnath and the National Institute of Standards and Technology (US) have investigated the Park–Ang damage index and its association with the different values of displacement and the energy dissipated. This investigation resulted in some discoveries; one of these findings has been stated that at different deformation values, the same energy dissipated leads to different values of damage index and damage level. Based on these findings, Wang et al. [48] have provided another contribution to modify for the Park–Ang damage index, and it has been expressed as follows:
Here
However, the structural members most likely would be subjected to three-dimensional loading during the seismic events, i.e., the damage probably could be in the three dimensions. The original formula of the Park–Ang damage index has been proposed and used considering axial load and uniaxial bending only. Based on this fact, the structural elements most likely would be subjected to three-dimensional loading. Consequently, it would be subjected to three-dimensional damage. Guo et al. [51] have developed the Park–Ang damage index and proposed a modified formula for the Park–Ang damage index called a three-dimensional damage index to account for the biaxial effect due to earthquake events. Therefore, his study has been based on the Park–Ang damage index and its improvements to modify the Park–Ang damage index with a biaxial effect to get a relation between moment and rotation for a more effective and more precise determination of the damage index of the reinforced concrete pier. Lastly, a three-dimensional damage index has been proposed and presented as follows:
Here
Here
2.1.2 Non-Cumulative Damage Index if No Cyclic Loading Exists
(1) Drift damage index
Damage indices, mainly dependent on degradation in structure stiffness, have been widely explored [52,53]. The drift damage index or the maximum deformation damage index is considered one of the most detailed damage indices in which the lateral displacement or the floor drift can indicate the structural damage if no cyclic loading exists. This index can be simply calculated by evaluating the inter-story drift based on the maximum lateral displacement between floors [52]. According to the lateral deformation between floors, the inter-story drift damage index (
Here
The damage in the structures is mainly occurred due to the plastic deformation, not the total deformation. Consequently, the inter-story drift damage index has been modified and presented in another formulation by subtracting the part related to elastic displacement from the maximum displacement and the plastic drift damage index
Here
(2) Displacement ductility-based damage index
In the set of the non-cumulative damage indices, another formula for the damage index called displacement ductility-based damage index based on the ductility of the member had been developed and introduced. Since the ductility tends to the structure ability towards plastic deformation without complete failure and degradation of structural strength, therefore it has been used as an indication of the structural damage, and ductility damage index has been widely used in seismic analysis to evaluate the seismic damage and the capacity of structures [54]. The ductility-based index (
Here
(3) Flexural damage ratio (FDR)
It has been generally concluded that the structural element’s damage level depends on the maximum deformation and mainly depends on the number of load cycles and the energy dissipated [24,25]. Hence, a new formula for damage index developed as a measure of the local stiffness degradation and called the flexural damage ratio (
Here
In the nonlinear analysis of structures subjected to static horizontal load, it is necessary to consider damage index, which incorporates stiffness degradation. Relying on this fact, Skærbæk et al. [55] proposed a new formula of the damage index for the individual column or beam and the damage index for beam or column
Here
According to the stiffness degradation, a new formula for the damage index has been proposed and presented [56]. The formulation of this damage index was associated with the relationship between ultimate and yielding deformation at the ultimate and yielding stiffness. This index has included a design ductility value and has been presented as follows:
Here
This section introduces the global damage indices used to assess the damage for the whole damaged structure. The global damage indices are most likely quantified by weighting the local indices of the different elements of a specific structure. The global damage indices have been classified as follows:
• Strength-based global damage indices.
• Weighted average global damage indices.
2.2.1 Strength-Based Global Damage Indices
Powell et al. [16] have mentioned that the deformation-based damage index
where
By adopting the same previous concept, Roufaiel et al. [57] have introduced a formula for the damage index, and the formula presented as follows:
where
2.2.2 Weighted Average Global Damage Indices
The most prevalent global damage indices that use the energy absorbed at different locations are the weighting function developed and presented by weighting and summing the local damage indices of the individual elements [33,44]. The overall damage index
Here N represents the number of structural components,
where
However, severely damaged members might limit its overall stability; this has not been reflected in the averaging effect of the previous equation. Therefore, Bracci et al. [58] proposed a global damage index that accounts for the severity of damage in the structure, and it has been presented as follows:
Higher values of parameter b are used when more emphasis on the most severely damaged member is required.
Moreover, Amziane et al. [59] presented a methodology to evaluate the global damage indices taking into account pseudo plastic hinges. Hanganu et al. [60] presented a procedure to assess RC structural local and global damage based on a concrete damage model.
3 Dynamic Properties-Based Damage Indices
Natural frequencies, damping ratios, modal participation factor, and mode shapes represent the most common modal properties obtained from the analytical solution of time histories, which can indicate the damage performance of the structures and have been used to calculate story damage index [61,62]. Pandey et al. [63] have presented a procedure to assess the damage by flexibility matrices based on the changes that occurred in the mentioned dynamic parameters.
3.1 Damage Indices Based on Natural Frequencies (Fundamental Periods)
Based on the fact that modal damage assessment can be performed based on changes in these dynamic properties, Dipasquale et al. [62] have proposed the ultimate stiffness degradation using the fundamental period of the undamaged structure as a reference, and the damage index has been introduced as follows:
where
Since the structures suffering softening when damage increases and after a specific step, structures suffering severe softening and become irreparable. Therefore, several damage indices have been proposed to account for this softening and accounting for the fundamental period variation [62,64–66]; thus, the following damage indices have been developed and presented:
(1) The maximum softening damage index
(2) The plastic softening damage index
(3) The final softening damage index
Here

Figure 3: Fundamental period time history [66]
The period degradation might be considered as an indication of the stiffness degradation. According to this concept, Hori et al. [67] have developed a formula to quantify period degradation based on design ductility, and it has been presented as follows:
Here
3.2 Damage Indices Based on Damping Ratio
3.2.1 Normalized Damping Ratio
As common knowledge, structure deterioration creates an increase in damping, especially in nonlinear material such as concrete, where damping enhancement is related to concrete cracking and yielding steel reinforcement. Therefore, the normalized damping ratio changes and then serves as a damage indicator [68]. Hence, damage evaluated and presented as follows:
where
Moreover, Wang et al. [61] used different modal parameters such as damping ratio, natural frequency, participation factor, and mode shape to estimate the story damage index.
The damage states are usually used to estimate the structural damage level, and this has been done according to the values of the damage indices determined for the structure, as mentioned in published literature. Also, these damage states are used to correlate the damage indices with the damage that occurred in the actual structures. Therefore, the damage states have been classified according to the damage indices values. The damage index is a normalized quantity where the value of this quantity ranges from zero and 1.0. Zero value of the damage index represents the undamaged structure and mean that the structural behavior still in the elastic stage and did not suffer any damage, while the unit value of the damage index refers to the failure of the structure, and this means a part of the whole of the structure is collapsed. The damage states also can be classified according to the cost required for repairing the structure due to the occurred damage. Priestley [69] mentioned that the damage state of the structures is significantly associated with their member’s deformation and their maximum strain.
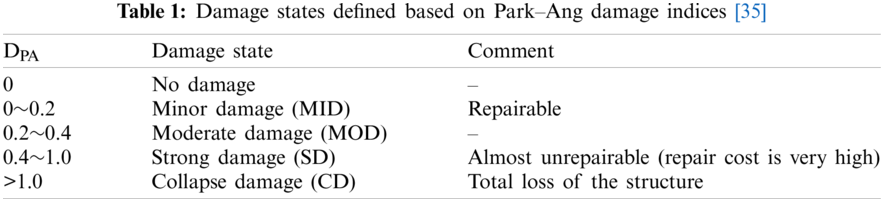
Generally, the available damage states have been defined based on damage factors, engineering judgment, or experimental calibration. One of the limitations of the available damage states that most of these damage states have not been defined or classified according to the structural response parameters and have not considered the differences in the structure lateral load resisting system and nonstructural elements damage. Here are some of the damage states mentioned in the literature. One of the damage state definitions was related to Park–Ang damage indices [35], where the degree of the damage of the structure has been determined according to the Park–Ang damage index calculated for the structure as provided in Tab. 1.
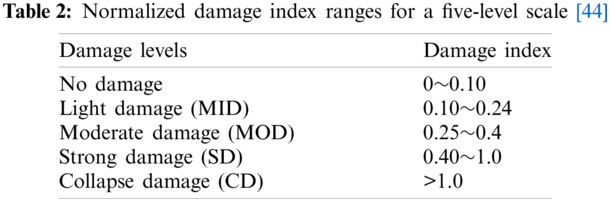
Also, Kunnath [23] introduced ranges for five degrees of the damage states according to the values of the normalized damage index, and it has been presented in Tab. 2.
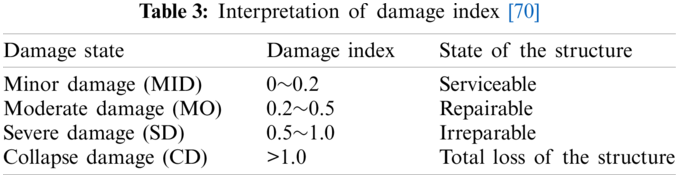
The degree of the damage can also be evaluated through the damage index by comparing the specific structural response parameters induced by the seismic event with the structural deformation capacity. However, the ductility demand and amount of dissipated hysteretic energy are effective parameters of the nonlinear response; they do not provide information on the degree of damage by themselves. Therefore, the structural available deformation capacity must be known to get a reliable estimation of the damage level of the structure. Ladjinovic et al. [70] defined the relationship between the damage index and the damage degree according to the data recorded on damage in RC buildings that subjected to moderate or severe damage during several seismic hazards in the USA and Japan, and the classification of the damage states has been presented as in Tab. 3.
Since the story drift is considered one of the most influential and earliest tools to evaluate and assess structural damage, in many building codes [71–73], floor drift has been used as a damage indicator of the structure. The FEMA 356 guidelines [73] described the performance levels of the structure based on the floor drift as presented in Tab. 4.

5 Case Studies and Applications on Damage Assessment of RC Structures
Cho et al. [74] presented an approach for evaluating seismic damage of concrete containment structures using nonlinear finite element analysis. In this study, two types of damage indices have been introduced and quantified at the finite element and structural levels. Nonlinear time-history analysis for the studied containment has been performed using a layered shell approach, and the damage indices at the finite element and structural levels have been estimated, which was also then used to evaluate the damage of the containment structure. Thirty as-recorded seismic waves have been considered in this study. For each wave, values range from 0.1 to 1.6 g for the peak ground acceleration (PGA) have been considered. The damage index at the finite element level and the structural level has been investigated. The damage index proposed by Roufaiel et al. [57] has been adopted to determine the damage index at the structural level as expressed in Eq. (21). This index uses

Figure 4: Damage index at the structural level [74]
Zhang et al. [75] have presented a study to investigate the effects of aftershocks on the damage of concrete gravity dams by considering 30 as-recorded main shock–aftershock seismic wave, taking Konya dam as a case study. In this study, a two-dimensional gravity dam was analyzed by applying the chosen seismic sequences to investigate the nonlinear behavior and the seismic damage for the Koyna dam under mainshock–aftershock seismic sequences. The local and global damage indices have been used in this study to investigate the effect of strong aftershocks seismic waves on the cumulative damage of concrete gravity dams. The results showed that the as-recorded sequences of ground motions significantly affected the accumulated damage and consequently the structural design of concrete gravity dams, see Fig. 5. In this study, the global damage has been calculated by weighting the local damage index at the ends of each element, with the dissipated energy where the local damage index
Here

Figure 5: Seismic wave effect on the global damage index calculated for the dam [75]
Zhai et al. [20] presented a comprehensive study to explore the influence and effect of aftershocks on the accumulative damage of containment structures by estimating the after-main shock damage levels. In this study, material stiffness degradation in the concrete damage plasticity (CDP) model has been used as an indication of the damage process and used to describe the damage that occurred in the concrete. The accumulative tensile and compressive damage have been reflected through the damage parameters
where
Massumi et al. [76] have modified the Park–Ang damage index to consider the structural fundamental period in damage index estimation and introduced a new formula for the damage index for RC structures according to the variations of the nonlinear fundamental period, which could be estimated experimentally through field tests. In this study, a correlation between the Park–Ang damage index and the fundamental period elongation has been presented. This new damage assessment has great importance since it accounts for the fundamental period elongation, which reflects the structure softening and encompasses one of the most effective, significant, and widely used damage indices (Park–Ang damage index). Also, this criterion is considered more reliable since it has associated with the fundamental period, which can reflect the actual seismic behavior of the structures that are induced due to their configuration, their construction quality, dissipated energy, and deformation of different elements. For this purpose, six flexural RC frames have been analyzed. Pushover analyses have been used to identify and reflect seismic behavior and performance of the RC frames step by step. In each step, the fundamental period and damage of analyzed frames have been investigated. Finally, a new damage index based on this significant correlation has been proposed and introduced. During the analysis process, the assessment indicates an increase in the fundamental period with the increase of the base shear parameter in each step according to the Park–Ang damage index, and this approach continued till the fundamental period suddenly shift, as shown in Figs. 6 and 7 for a 10-story frame (δ is the fundamental period elongation).
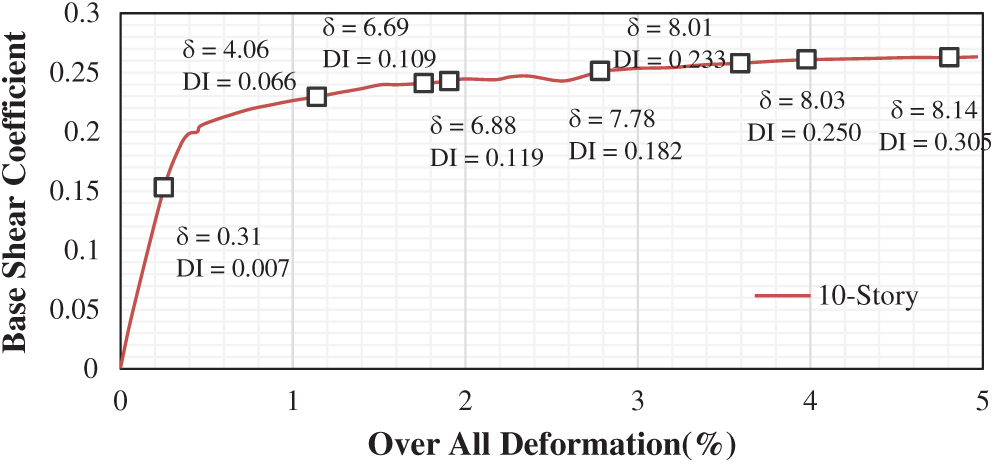
Figure 6: Fundamental period elongation corresponding to the damage rate [76]

Figure 7: Period elongation pursuit corresponding to the damage rate [76]
Based on this study, the developed damage index has been presented as follows:
where
Moreover, the deformation has been expressed as follows:
Here H refers to the height of the reinforced concrete frames presented in meters,

Figure 8: Damage coefficient value of α and β [76] (a) Damage coefficient value of α (b) Damage coefficient value of β
The proposed damage pattern in this study also represented in a new format as follows:
The value of δcritical indicates the initial elastic period; based on some references; the experimental elastic period can be replaced by the analytical elastic period in this newly developed damage formula since it slightly affected the results. Therefore, this new approach has great importance for structures whose initial elastic periods not available or cannot be determined experimentally.
Carrillo et al. [77] investigated and assessed the damage of squat, thin, and lightly-RC walls by modifying the Park–Ang damage index. In this study, the damage performance of the squat, thin, and lightly-RC walls has been experimentally investigated and tested under monotonic and cyclic loading until the failure occurred. During these tests, the damage evolution and the cracking pattern on the tested RC walls have been observed at different damage states. The experimental program contained 25 RC walls, including walls with and without openings with different height-to-length ratios equal to 0.5, 1.0, and 2.0. Three different concrete types, including (normal-weight, light-weight, and self-consolidating) concrete, have been used in the tested specimens using compressive strength of 15 MPa. In this study, the Park–Ang damage index has been adopted to evaluate the damage on these specimens. For most of the tested specimens, parameter β was quantified using Eq. (6), which was presented by Park et al. [32,33] in the original formula of Park–Ang damage index calculations resulting in negative values. Consequently, the damage index could not be calculated. Therefore, it has been concluded from this study that for squat, thin, and lightly-RC walls where shear deformation is dominated instead of flexural deformations, the formula initially proposed by Park et al. [33,35] to calculate parameter β in slender elements was not applicable for such these walls. In this study, parameter β has been experimentally determined from the hysteretic response of 25 wall specimens to overcome Park–Ang damage index shortcoming, and a new formula for the parameter β has been provided. The new formula for β has been developed by considering that all wall specimens reached the failure limit and at the failure limit, the damage index is supposed to equal 1.0, accordingly the experimental-based formula for parameter β has been presented as follows:
The values of β calculated using the previous formula of
where
where
Moreover, much work has been performed to investigate and identify the seismic response of several RC structures subjected to near-fault ground motions [44,79]. For the same intensity and duration of ground motions, near-fault ground motions lead to severe damage and higher seismic response on the structures compared with far-fault ground motions [80]. Rodriguez et al. [81] have done a further study to estimate the DI for RC columns. In this study, DI depends on hysteretic energy has been investigated by a structural member and the drift ratio of 76 RC columns.
Guo et al. [39] developed a new formula to account for the mainshock-aftershock seismic wave based on the modified Park–Ang formula, which has been introduced previously by Kunnath et al. [44,45]. According to this study, the accumulative damage of the structures due to a mainshock-aftershock
The first term in the previous equations refers to the damage that occurred due to mainshock
Here
where
In this study, an overview of available damage assessment methods through damage indices is presented. Their formulation, features, limitations, and progressive development of these indices have also been introduced. According to this review, it can be concluded that:
• These damage indices are considered an effective tool to quantify the degree of structural components’ damage or the overall structural damage.
• Damage indices can practically be used in the evaluation of damage induced due to seismic events.
• In this context, it should be mentioned that researchers widely use the well-known Park–Ang damage index to assess the damage because of its high accuracy and simplicity in application.
• Park–Ang damage index is initially proposed for slender sections (slender beam and columns) where the flexural deformations dominated. However, it is rarely calibrated for shell structures or for the element in which shear deformation dominated instead of flexural deformations such as RC walls.
Funding Statement: This work was supported by the National Natural Science Foundation of China (Grant No. 52078361) and the Innovation Program of the Shanghai Municipal Education Commission (Grant No. 2017-01-07-00-07-E00006).
Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.
1. Paal, S., Jeon, J., Brilakis, I., DesRoches, R. (2015). Automated damage index estimation of reinforced concrete columns for post-earthquake evaluations. Journal of Structural Engineering, 141(9), 1–13. DOI 10.1061/(ASCE)ST.1943-541X.0001200. [Google Scholar] [CrossRef]
2. Huang, C., El Hami, A., Radi, B. (2017). Overview of structural reliability analysis methods—Part I : Local reliability methods. Incertitudes et fiabilité des systèmes multiphysiques, 1. DOI 10.21494/ISTE.OP.2017.0115. [Google Scholar] [CrossRef]
3. Huang, T., Ren, X., Li, J. (2017). Incremental dynamic analysis of seismic collapse of super-tall building structures. Structural Design of Tall and Special Buildings, 26, 1–11. DOI 10.1002/tal.1370. [Google Scholar] [CrossRef]
4. Li, Z., Gao, X., Tang, Z. (2020). Safety performance of a precast concrete barrier: Numerical study. Computer Modeling in Engineering and Sciences, 123(3), 1105–1129. DOI 10.32604/cmes.2020.09047. [Google Scholar] [CrossRef]
5. Zhang, T., Deng, J., Wang, J. (2020). Progressive damage analysis (PDA) of carbon fiber plates with out-of-plane fold under pressure. Computer Modeling in Engineering and Sciences, 124(2), 545–559. DOI 10.32604/cmes.2020.09536. [Google Scholar] [CrossRef]
6. https://www.eeri.org/site/images/eeri_newsletter/2008_pdf/Wenchuan_China_Recon_Rpt.pdf. [Google Scholar]
7. Cao, V. V., Ronagh, H. R. (2014). Correlation between seismic parameters of far-fault motions and damage indices of low-rise reinforced concrete frames. Soil Dynamics and Earthquake Engineering, 66(1), 102–112. DOI 10.1016/j.soildyn.2014.06.020. [Google Scholar] [CrossRef]
8. Elenas, A., Meskouris, K. (2004). Correlation study between seismic acceleration parameters and damage indices of structures. Engineering Structures 200, 23(6), 698–704. DOI 10.1016/S0141-0296(00)00074-2. [Google Scholar] [CrossRef]
9. Rodriguez, M. (2008). Selecting earthquake ground motion records for nonlinear analysis of structures. Proceedings MERCEA Conference. Regio-Calabria, Italy. [Google Scholar]
10. Bozorgnia, Y., Bertero, V. (2003). Damage spectra: Characteristics and applications to seismic risk reduction. Journal of Structural Engineering, 129(10), 1330–1340. DOI 10.1061/(ASCE)0733-9445(2003)129:10(1330). [Google Scholar] [CrossRef]
11. Krawinkler, H., Zohrei, M. (1983). Cumulative damage in steel structures subjected to earthquake ground motions. Computers & Structures, 16(1–4), 531–541. DOI 10.1016/0045-7949(83)90193-1. [Google Scholar] [CrossRef]
12. Lybas, J., Sozen, M. (1977). Effect of beam strength and stiffness on the dynamic behavior of reinforced concrete coupled walls. Civil Engineering Studies, Structural Research Series No. 444. Illinois: University of Urbana. [Google Scholar]
13. Park, Y., Ang, A., Wen, Y. (1985). Seismic damage analysis of reinforced concrete buildings. Journal of Structural Engineering, 111(4), 740–757. DOI 10.1061/(ASCE)0733-9445(1985)111:4(740). [Google Scholar] [CrossRef]
14. Bertero, V., Bresler, B. (1971). Developing methodologies for evaluating the earthquake safety of existing buildings. Report No. UCB/EERC-77-06. Berkeley: University of California. [Google Scholar]
15. Calvi, G. M., Kingsley, G. R. (1995). Displacement-based seismic design of multi-degree-of-freedom bridges structures. Earthquake Engineering and Structural Dynamics, 24(9), 1247–1266. DOI 10.1002/eqe.4290240906. [Google Scholar] [CrossRef]
16. Powell, G. H., Allahabadi, R. (1988). Seismic damage prediction by the deterministic method: Concepts and procedures. Earthquake Engineering and Structural Dynamics, 16(5), 719–734. DOI 10.1002/eqe.4290160507. [Google Scholar] [CrossRef]
17. Reinhorn, A., Kunnath, S., Bracci, J., Mander, J. (1989). Normalized damage index for evaluation of buildings. Proceedings of ASCE Structures Congress, pp. 507–516. San Francisco, New York. [Google Scholar]
18. Banon H., Veneziano D. (1982). Seismic safety of reinforced concrete members and structures. Earthquake Engineering and Structural Dynamics, 10(2), 179–193. DOI 10.1002/eqe.4290100202. [Google Scholar] [CrossRef]
19. Kappos, A. (1977). Seismic damage indices for RC buildings: Evaluation of concepts and procedures. Progress in Structural Engineering and Materials, 1(1), 78–87. DOI 10.1002/pse.2260010113. [Google Scholar] [CrossRef]
20. Zhai, C., Bao, X., Zheng, Z., Wang, X. (2018). Impact of aftershocks on a post-mainshock damaged containment structure considering duration. Soil Dynamics and Earthquake Engineering, 115(13), 129–141. DOI 10.1016/j.soildyn.2018.08.013. [Google Scholar] [CrossRef]
21. Kappos, A. J., Stylianidis, K. C., Michailidis, C. N., Athanassiadou, C. J. (1992). Development of earthquake damage scenarios using a comprehensive analytical method. Proceeding of the 10th World Conference on Earthquake Engineering, pp. 6013–6018. Madrid, Spain. [Google Scholar]
22. Golafshani, A. A., Bakhshi, A., Tabeshpour, M. R. (2005). Vulnerability and damage analysis of existing buildings. Asian Journal of Civil Engineering, 16, 85–100. https://www.sid.ir/en/journal/ViewPaper.aspx?id=48875. [Google Scholar]
23. Mieses, Hernandez, L. A. (2007). Seismic Performance and Fragility Curves for Reinforced Concrete Frames and Shear Wall Residential Buildings in Puerto Rico. San Juan, Puerto Rico: University of Puerto Rico. [Google Scholar]
24. Banon, H., Biggs, J., Irvine, H. (1980). Prediction of seismic damage in reinforced concrete frames seismic behavior and design of buildings. Report No. 3. Cambridge, Mass: MIT. [Google Scholar]
25. Banon, H., Biggs, J., Irvine, H. (1981). Seismic damage in reinforced concrete members. Journal of Structural Engineering, 107(9), 1713–1729. DOI 10.1061/JSDEAG.0005778?. [Google Scholar] [CrossRef]
26. Colombo, A., Negro, P. (2005). A damage index of generalized applicability. Engineering Structures, 27(8), 1164–1174. DOI 10.1016/j.engstruct.2005.02.014. [Google Scholar] [CrossRef]
27. Miner, M. A. (1945). Cumulative damage in fatigue. Journal of Applied Mechanics, 12(3), 159–164. DOI 10.1115/1.4009458. [Google Scholar] [CrossRef]
28. Gosain, N. K., Brown, R. H., Jirsa, J. O. (1977). Shear requirements for load reversals on RC members. Journal of Structural Engineering, 113(7), 1461–1476. DOI 10.1061/JSDEAG.0004677. [Google Scholar] [CrossRef]
29. Hwang, T. H., Scribner, C. F. (1984). R/C member cyclic response during various loadings. Journal of Structural Engineering, 110(3), 477–489. DOI 10.1061/(ASCE)0733-9445(1984)110:3(477). [Google Scholar] [CrossRef]
30. Teran, G. A. (1996). Performance-based earthquake-resistant design of framed buildings using energy concepts. Berkeley: University of California. [Google Scholar]
31. Ye, L. P., Cheng, G. Y. (2012). Study on energy-based seismic design method and application on steel braced frame structure. Journal of Building Structures, 33(11), 36–45 (in Chinese). [Google Scholar]
32. Park, Y., Ang, A. (1985). Mechanistic seismic damage model for reinforced concrete. Journal of Structural Engineering, 111(4), 722–739. DOI 10.1061/(ASCE)0733-9445(1985)111:4(722). [Google Scholar] [CrossRef]
33. Park, Y., Ang, A., Wen, Y. (1985). Seismic damage analysis and damage-limiting design of RC buildings. Civil Engineering Studies SRS-516. Urbana-Champaign: College of Engineering, University of Illinois Engineering Experiment Station. http://hdl.handle.net/2142/14124. [Google Scholar]
34. Cosenza, E., Manfredi, G., Ramasco, R. (1993). The use of damage functional in earthquake-resistant design: A comparison among different procedures. Structural Dynamics and Earthquake Engineering, 22(3), 855–868. DOI 10.1002/eqe.4290221003. [Google Scholar] [CrossRef]
35. Park, Y., Ang, A., Wen, Y. (1987). Damage-limiting aseismic design of buildings. Earthquake Spectra, 3(1), 1–26. DOI 10.1193/1.1585416. [Google Scholar] [CrossRef]
36. Alarcón, E., Recuero, A., Perera, R., López, C., Gutiérrez, J. et al. (2001). A repairability index for reinforced concrete members based on fracture mechanics. Engineering Structures, 23(6), 687–697. DOI 10.1016/S0141-0296(00)00075-4. [Google Scholar] [CrossRef]
37. Chai, Y. H., Romstad, K. M., Bird, S. M. (1995). Energy-based linear damage model for high-intensity seismic loading. Journal of Structural Engineering, 121(5), 857–864. DOI 10.1061/(ASCE)0733-9445(1995)121:5(857). [Google Scholar] [CrossRef]
38. Ang, A. H. S., de Leon, D. (1994). Reliability and response control of R/C buildings. ASCE Structures Congress XII, pp. 1593–1599. Georgia, USA: ASCE. [Google Scholar]
39. Guo, X., He, Z., Xu, J. (2020). Identification of incremental seismic damage development in RC structures excited with sequence-type ground motions. Structures, Elsevier, 24(7), 464–476. DOI 10.1016/j.istruc.2020.01.012. [Google Scholar] [CrossRef]
40. Shen, J., Ren, X., Zhang, Y., Chen, J. (2019). Nonlinear dynamic analysis of frame-core tube building under seismic sequential ground motions by a supercomputer. Soil Dynamics and Earthquake Engineering, 124, 86–97. DOI 10.1016/j.soildyn.2019.05.036. [Google Scholar] [CrossRef]
41. Jia, S., Cao, W., Zhang, Y. (2017). Damage index calibration of frame-supported concealed multi-ribbed wall panels with energy-efficient blocks. Applied Sciences (Switzerland), 7(5), 453. DOI 10.3390/app7050453. [Google Scholar] [CrossRef]
42. Huang, W., Qian, J., Zhou, Z. (2016). Seismic damage assessment of steel-reinforced concrete members by a modified Park–Ang model. Journal of Asian Architecture and Building Engineering, 15(3), 605–611. DOI 10.3130/jaabe.15.605. [Google Scholar] [CrossRef]
43. Wen, Y. K. (1980). Equivalent linearization for hysteretic systems under random excitations. Journal of Applied Mechanics, 47(1), 150–154. DOI 10.1115/1.3153594. [Google Scholar] [CrossRef]
44. Kunnath, S., Reinhorn, A. M., Lobo, R. F. (1992). IDARC version 30: A program for the inelastic damage analysis of reinforced concrete structures. Technical Report NCEER-92-0022iiat. Buffalo, NY: National Center for Earthquake Engineering Research, State University of New York. [Google Scholar]
45. Kunnath, S., Reinhorn, A., Abel, J. (1991). Computational tool for evaluation of seismic performance of reinforced concrete buildings. Computers & Structures, 41(1), 157–173. DOI 10.1016/0045-7949(91)90165-I. [Google Scholar] [CrossRef]
46. Bozorgnia, Y., Bertero, W. (2001). Evaluation of the damage potential of recorded earthquake ground motion. Proceedings of the 96th Annual Meeting of Seismological Society of America. Seismological Research Letters, vol. 72, pp. 233. Tehran: Jahad Daneshgahi University. [Google Scholar]
47. Bozorgnia, Y., Bertero, V. (2001). Improved shaking and damage parameters for post-earthquake applications. Proceedings, SMIP01 Seminar on Utilization of Strong-Motion Data, pp. 1–22. Los Angeles, USA. [Google Scholar]
48. Wang, D., Feng, Q., Wang, G. X. (2004). A modified Park and Ang seismic damage model considering low-cycle fatigue life. China Civil Engineering Journal, 37(11), 41–49 (in Chinese). DOI 10.3321/j.issn:1000-131X.2004.11.007. [Google Scholar] [CrossRef]
49. Kunnath, S. (1997). Cumulative seismic damage of reinforced concrete bridge piers. Gaithersburg, MD: US Department of Commerce, Technology Administration, National Institute of Standards and Technology. [Google Scholar]
50. Jaing, H. J., Chen, L. Z., Chen, Q. (2011). Seismic damage assessment and performance levels of reinforced concrete members. Procedia Engineering, 14(2), 939–945. DOI 10.1016/j.proeng.2011.07.118. [Google Scholar] [CrossRef]
51. Guo, J., Wang, J. J., Li, Y., Zha, W. G., Du, Y. L. (2016). Three-dimensional extension for Park and Ang damage model. Structures, 7(10), 184–194. DOI 10.1016/j.istruc.2016.06.008. [Google Scholar] [CrossRef]
52. Ghobarah, A., Abou-Elfath, H., Biddah, A. (1999). Response-based damage assessment of structures. Earthquake Engineering and Structural Dynamics, 28, 79–104. DOI 10.1002/(SICI)1096-9845(199901)28:1<79::AID-EQE805>3.0.CO;2-J. [Google Scholar] [CrossRef]
53. Kim, T., Lee, K., Chung, Y. S., Shin, H. (2005). Seismic damage assessment of reinforced concrete bridge columns. Engineering Structures, 27(4), 576–592. DOI 10.1016/j.engstruct.2004.11.016. [Google Scholar] [CrossRef]
54. Newmark, N. M., Rosenblueth, E. (1974). Fundamentals of earthquake engineering. Englewood Cliffs, NJ: Prentice-Hall. [Google Scholar]
55. Skærbæk, S., Nielsen, S., Kierkegaard, H. P., Çakmak, A. Ş. (1980). Damage localization and quantification of earthquake excited RC-frames. Earthquake Engineering and Structural Dynamics, 27, 903–916. DOI 10.1002/(SICI)1096-9845(199809)27:9<903::AID-EQE757>3.0.CO;2-C. [Google Scholar] [CrossRef]
56. Gupta, S. P., Nielsen, R., Kierkegaard, H. (2001). A preliminary prediction of seismic damage-based degradation in RC structures. Earthquake Engineering and Structural Dynamics, 30(7), 981–933. DOI 10.1002/eqe.45. [Google Scholar] [CrossRef]
57. Roufaiel, M., Meyer, C. (1987). Analytical modeling of hysteretic behavior of R/C frames. Journal of Structural Engineering, 113(3), 429–444. DOI 10.1061/(ASCE)0733-9445(1987)113:3(429). [Google Scholar] [CrossRef]
58. Bracci, J., Reinhorn, A., Kunnath, S., Mander (1989). A deterministic model for seismic damage evaluation of RC structures. Technical Report NCEER-89-0033. Buffalo, NY: National Center for Earthquake Engineering Research, State University of New York. [Google Scholar]
59. Amziane, S., Dube, J. F. (2008). Global RC structural damage index based on the assessment of local material damage. Journal of Advanced Concrete Technology, 6(3), 459–468. DOI 10.3151/jact.6.459. [Google Scholar] [CrossRef]
60. Hanganu, A. D., Õnate,, E., Barbat, A. H. (2002). A finite element methodology for local/global damage evaluation in civil engineering structures. Computers and Structures, 80, 1667–1687. DOI 10.1016/S0045-7949(02)00012-3. [Google Scholar] [CrossRef]
61. Wang, J. F., Lin, C. C., Yen, S. M. (2007). A story damage index of seismically-excited buildings based on modal frequency and mode shape. Engineering Structures, 29(9), 2143–2157. DOI 10.1016/j.engstruct.2006.10.018. [Google Scholar] [CrossRef]
62. DiPasquale, E., Cakmak, A. (1987). Detection and assessment of seismic structural damage. Technical Report NCEER-87-0015. Buffalo, NY: National Center for Earthquake Engineering Research, State University of New York. [Google Scholar]
63. Pandey, A. K., Biswas, M. (1994). Damage detection in structures using changes in flexibility. Journal of Sound and Vibration, 196(1), 3–17. DOI 10.1006/jsvi.1994.1002. [Google Scholar] [CrossRef]
64. DiPasquale, E., Cakmak, A. (1988). Identification of the serviceability limit state and detection of seismic structural damage. Technical Report NCEER-88-0022. Buffalo, NY: National Center for Earthquake Engineering Research, State University of New York. [Google Scholar]
65. DiPasquale, E., Cakmak, A. S. (1989). On the relation between local and damage indices. Technical Report NCEER-89-0034. Buffalo, NY: National Center for Earthquake Engineering Research, State University of New York. [Google Scholar]
66. DiPasquale, E., Ju, J. W., Cakmak, A. (1990). Relation between damage indices and local stiffness degradation. Journal of Structural Engineering, 116(5), 1440–1456. DOI 10.1061/(ASCE)0733-9445(1990)116:5(1440). [Google Scholar] [CrossRef]
67. Hori, N., Inoue, N. (2002). Damaging properties of ground motions and prediction of maximum response of structures based on momentary energy response. Earthquake Engineering and Structural Dynamics, 31(9), 1657–1679. DOI 10.1002/eqe.183. [Google Scholar] [CrossRef]
68. Richardson, M. H., Mannan, M. A. (1993). Correlating minute structural faults with changes in modal parameters. Proceeding 11th Modal Analysis Conference, pp. 893–898. Kissimmee Florida. [Google Scholar]
69. Priestley, M. J. (2002). Direct displacement-based design of precast/ prestressed concrete buildings. PCI Journal, 47(6), 66–79. DOI 10.15554/pcij. [Google Scholar] [CrossRef]
70. Ladjinovic, D., Folic, R. (2004). Application of improved damage index for designing of earthquake-resistant structures. 13th World Conference on Earthquake Engineering. Vancouver, Canada. [Google Scholar]
71. Poland, C. D., Hill, J., Sharpe, R. L., Soulages, J. (1995). Vision 2000: Performance-based seismic engineering. Structural engineers. Berlin, Germany: Springer. [Google Scholar]
72. Applied Technology Council (1996). ATC 40: Seismic evaluation and retrofit of concrete buildings. Report No. SSC 96-01. Redwood City, CA. [Google Scholar]
73. FEMA 356 (2000). Prestandard and commentary for the seismic rehabilitation of buildings. Prepared by the American Society of Civil Engineers Reston, Virginia, Prepared for the Federal Emergency Management Agency, Washington DC, USA. [Google Scholar]
74. HoHyun, C., Hyun, M., Chang, H., Hyun, M. (2003). Seismic damage assessment of reinforced concrete containment structures. Transactions of the 17th International Conference on Structural Mechanics in Reactor Technology, pp. 17–22. Prague, Czech Republic. [Google Scholar]
75. Zhang, S., Wang, G., Sa, W. (2013). Damage evaluation of concrete gravity dams under mainshock-aftershock seismic sequences. Soil Dynamics and Earthquake Engineering, 50(20), 16–27. DOI 10.1016/j.soildyn.2013.02.021. [Google Scholar] [CrossRef]
76. Massumi, A., Moshtagh, E. (2010). A new damage index for RC buildings based on variations of nonlinear fundamental period. Structural Design of Tall and Special Buildings, 22(1), 50–61. DOI 10.1002/tal.656. [Google Scholar] [CrossRef]
77. Carrillo, J., Oyarzo-Ver, C., Blandon, C. (2019). Damage assessment of squat, thin, and lightly-reinforced concrete walls by the Park & Ang damage index. Journal of Building Engineering, 26(2), 1009–1021. DOI 10.1016/j.jobe.2019.100921. [Google Scholar] [CrossRef]
78. Oyarzo, V. C. (2006). Damage evaluation in R/C shear walls using the damage index of Park & Ang. Proceedings of the First European Conference on Earthquake Engineering and Seismology, Geneva, Switzerland. [Google Scholar]
79. Dicleli, M., Mehta, A. (2007). Effect of near-fault ground motion and damper characteristics on the seismic performance of chevron braced steel frames. Earthquake Engineering and Structural Dynamics, 36(7), 927–948. DOI 10.1002/eqe.662. [Google Scholar] [CrossRef]
80. Loh, C. H., Liao, W., Chai, J. F. (2002). Effect of the near-fault earthquake on bridges: Lessons learned from the Chi-Chi earthquake. Proceedings of the 3rd National Seismic Conference and Workshop on Bridges and Highways, pp. 211–222. FHWA Western Resource Center and MCEER, Portland. [Google Scholar]
81. Rodriguez, M. E., Padilla, D. (2009). A damage index for the seismic analysis of reinforced concrete members. Earthquake Engineering and Structural Dynamics, 13, 364–383. DOI 10.1080/13632460802597893. [Google Scholar] [CrossRef]

 | This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. |