Neutrosophic <i>N</i>-Structures Applied to Sheffer Stroke BL-Algebras

Tugce Katican; Tahsin Oner; Akbar Rezaei; Florentin Smarandache

doi:10.32604/cmes.2021.016996

Loading [MathJax]/jax/element/mml/optable/MathOperators.js

[BACK]

Computer Modeling in Engineering & Sciences

DOI: 10.32604/cmes.2021.016996

ARTICLE

Neutrosophic $N$ -Structures Applied to Sheffer Stroke BL-Algebras

Tugce Katican1, Tahsin Oner1, Akbar Rezaei2,* and Florentin Smarandache3

1Department of Mathematics, Ege University, Izmir, 35100, Turkey
2Department of Mathematics, Payame Noor University, Tehran, 19395-4697, Iran
3Department of Mathematics and Science, University of New Mexico, Gallup, 87301, NM, USA
*Corresponding Author: Akbar Rezaei. Email: rezaei@pnu.ac.ir
Received: 18 April 2021; Accepted: 25 May 2021

Abstract: In this paper, we introduce a neutrosophic $N$ -subalgebra, a (ultra) neutrosophic $N$ -filter, level sets of these neutrosophic $N$ -structures and their properties on a Sheffer stroke BL-algebra. By defining a quasi-subalgebra of a Sheffer stroke BL-algebra, it is proved that the level set of neutrosophic $N$ -subalgebras on the algebraic structure is its quasi-subalgebra and vice versa. Then we show that the family of all neutrosophic $N$ -subalgebras of a Sheffer stroke BL-algebra forms a complete distributive lattice. After that a (ultra) neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is described, we demonstrate that every neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is its neutrosophic $N$ -subalgebra but the inverse is generally not true. Finally, it is presented that a level set of a (ultra) neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is also its (ultra) filter and the inverse is always true. Moreover, some features of neutrosophic $N$ -structures on a Sheffer stroke BL-algebra are investigated.

Keywords: Sheffer stroke BL-algebra; (ultra) filter; neutrosophic 𝒩-subalgebra; (ultra) neutrosophic 𝒩-filter

1 Introduction

Fuzzy set theory, which has the truth (t) (membership) function and state positive meaning of information, is introduced by Zadeh [1] as a generalization the classical set theory. This led scientists to find negative meaning of information. Hence, intuitionistic fuzzy sets [2] which are fuzzy sets with the falsehood (f) (nonmembership) function were introduced by Atanassov. However, there exist uncertainty and vagueness in the language, as well as positive ana negative meaning of information. Thus, Smarandache defined neutrosophic sets which are intuitionistic fuzzy sets with the indeterminacy/neutrality (i) function [3,4]. Thereby, neutrosophic sets are determined on three components: $(t,i,f):(truth, indeterminacy, falsehood)$ [5]. Since neutrosophy enables that information in language can be comprehensively examined at all points, many researchers applied neutrosophy to different theoretical areas such as BCK/BCI-algebras, BE-algebras, semigroups, metric spaces, Sheffer stroke Hilbert algebras and strong Sheffer stroke non-associative MV-algebras [6–15] so as to improve devices imitating human behaviours and thoughts, artificial intelligence and technological tools.

Sheffer stroke (or Sheffer operation) was originally introduced by Sheffer [16]. Since Sheffer stroke can be used by itself without any other logical operators to build a logical system which is easy to control, Sheffer stroke can be applied to many logical algebras such as Boolean algebras [17], ortholattices [18], Sheffer stroke Hilbert algebras [19]. On the other side, BL-algebras were introduced by Hájek as an axiom system of his Basic Logic (BL) for fuzzy propositional logic, and he widely studied many types of filters [20]. Moreover, Oner et al. [21] introduced BL-algebras with Sheffer operation and investigated some types of (fuzzy) filters.

We give fundamental definitions and notions about Sheffer stroke BL-algebras, $N$ -functions and neutrosophic $N$ -structures defined by these functions on a crispy set X. Then a neutrosophic $N$ -subalgebra and a $(τ,γ,ρ)$ -level set of a neutrosophic $N$ -structure are presented on Sheffer stroke BL-algebras. By defining a quasi-subalgebra of a Sheffer stroke BL-algebra, it is proved that every $(τ,γ,ρ)$ -level set of a neutrosophic $N$ -subalgebra of the algebra is the quasi-subalgebra and the inverse is true. Also, we show that the family of all neutrosophic $N$ -subalgebras of this algebraic structure forms a complete distributive lattice. Some properties of neutrosophic $N$ -subalgebras of Sheffer stroke BL-algebras are examined. Indeed, we investigate the case which $N$ -functions defining a neutrosophic $N$ -subalgebra of a Sheffer stroke BL-algebra are constant. Moreover, we define a (ultra) neutrosophic $N$ -filter of a Sheffer stroke BL-algebra by $N$ -functions and analyze many features. It is demonstrated that $(τ,γ,ρ)$ -level set of a neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is its filter but the inverse does not hold in general. In fact, we propound that $(τ,γ,ρ)$ -level set of a (ultra) neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is its (ultra) filter and the inverse is true. Finally, new subsets of a Sheffer stroke BL-algebra are defined by the $N$ -functions and special elements of the algebra. It is illustrated that these subsets are (ultra) filters of a Sheffer stroke BL-algebra for the (ultra) neutrosophic $N$ -filter but the special conditions are necessary to prove the inverse.

2 Preliminaries

In this section, basic definitions and notions on Sheffer stroke BL-algebras and neutrosophic $N$ -structures.

Definition 2.1. [18] Let $H=⟨H,∣⟩$ be a groupoid. The operation $∣$ is said to be a Sheffer stroke (or Sheffer operation) if it satisfies the following conditions:

(S1) $x∣y=y∣x,$

(S2) $(x∣x)∣(x∣y)=x,$

(S3) $x∣((y∣z)∣(y∣z))=((x∣y)∣(x∣y))∣z,$

(S4) $(x∣((x∣x)∣(y∣y)))∣(x∣((x∣x)∣(y∣y)))=x.$

Definition 2.2. [21] A Sheffer stroke BL-algebra is an algebra $(C,∨,∧,∣,0,1)$ of type (2, 2, 2, 0, 0) satisfying the following conditions:

(sBL −1) $(C,∨,∧,0,1)$ is a bounded lattice,

(sBL −2) $(C,∣)$ is a groupoid with the Sheffer stroke,

(sBL −3) $c1∧c2=(c1∣(c1∣(c2∣c2)))∣(c1∣(c1∣(c2∣c2)))$ ,

(sBL −4) $(c1∣(c2∣c2))∨(c2∣(c1∣c1))=1$ ,

for all $c1,c2∈C$ .

$1=0∣0$ is the greatest element and $0=1∣1$ is the least element of C.

Proposition 2.1. [21] In any Sheffer stroke BL-algebra C, the following features hold, for all $c1,c2,c3∈C$ :

(1) $c1∣((c2∣(c3∣c3))∣(c2∣(c3∣c3)))=c2∣((c1∣(c3∣c3))∣(c1∣(c3∣c3))),$

(2) $c1∣(c1∣c1)=1,$

(3) $1∣(c1∣c1)=c1,$

(4) $c1∣(1∣1)=1,$

(5) $(c1∣1)∣(c1∣1)=c1,$

(6) $(c1∣c2)∣(c1∣c2)≤c3⇔c1≤c2∣(c3∣c3)$

(7) $c1≤c2$ iff $c1∣(c2∣c2)=1,$

(8) $c1≤c2∣(c1∣c1),$

(9) $c1≤(c1∣c2)∣c2,$

(10) (a) $(c1∣(c1∣(c2∣c2)))∣(c1∣(c1∣(c2∣c2)))≤c1,$

(b) $(c1∣(c1∣(c2∣c2)))∣(c1∣(c1∣(c2∣c2)))≤c2.$

(11) If $c1≤c2$ , then

(i) $c3∣(c1∣c1)≤c3∣(c2∣c2),$

(ii) $(c1∣c3)∣(c1∣c3)≤(c2∣c3)∣(c2∣c3),$

(iii) $c2∣(c3∣c3)≤c1∣(c3∣c3).$

(12) $c1∣(c2∣c2)≤(c3∣(c1∣c1))∣((c3∣(c2∣c2))∣(c3∣(c2∣c2))),$

(13) $c1∣(c2∣c2)≤(c2∣(c3∣c3))∣((c1∣(c3∣c3))∣(c1∣(c3∣c3))),$

(14) $((c1∨c2)∣c3)∣((c1∨c2)∣c3)=((c1∣c3)∣(c1∣c3))∨((c2∣c3)∣(c2∣c3)),$

(15) $c1∨c2=((c1∣(c2∣c2))∣(c2∣c2))∧((c2∣(c1∣c1))∣(c1∣c1)).$

Lemma 2.1. [21] Let C be a Sheffer stroke BL-algebra. Then

$(c1∣(c2∣c2))∣(c2∣c2)=(c2∣(c1∣c1))∣(c1∣c1),$

for all $c1,c2∈C$ .

Corollary 2.1. [21] Let C be a Sheffer stroke BL-algebra. Then

$c1∨c2=(c1∣(c2∣c2))∣(c2∣c2),$

for all $c1,c2∈C$ .

Lemma 2.2. [21] Let C be a Sheffer stroke BL-algebra. Then

$c1∣((c2∣(c3∣c3))∣(c2∣(c3∣c3)))=(c1∣(c2∣c2))∣((c1∣(c3∣c3))∣(c1∣(c3∣c3))),$

for all $c1,c2,c3∈C$ .

Definition 2.3. [21] A filter of C is a nonempty subset $P⊆C$ satisfying

$(SF−1) ifc1,c2∈P, then (c1∣c2)∣(c1∣c2)∈P,(SF−2) if c1∈P and c1≤c2, then c2∈P.$

Proposition 2.2. [21] Let P be a nonempty subset of C. Then P is a filter of C if and only if the following hold:

$(SF−3)1∈P,(SF−4) c1∈P and c1∣(c2∣c2)∈P imply c2∈P.$

Definition 2.4. [21] Let P be a filter of C. Then P is called an ultra filter of C if it satisfies $c∈P$ or $c∣c∈P$ , for all $c∈C$ .

Lemma 2.3. [21] A filter P of C is an ultra filter of C if and only if $c1∨c2∈P$ implies $c1∈P$ or $c2∈P$ , for all $c1,c2∈C$ .

Definition 2.5. [8] $F(X,[−1,0])$ denotes the collection of functions from a set X to $[−1,0]$ and an element of $F(X,[−1,0])$ is called a negative-valued function from X to $[−1,0]$ (briefly, $N$ -function on X). An $N$ -structure refers to an ordered pair (X, f) of X and $N$ -function f on X.

Definition 2.6. [12] A neutrosophic $N$ -structure over a nonempty universe X is defined by

$XN:=X(TN,IN,FN)={x(TN(x),IN(x),FN(x)):x∈X}$

where TN, IN and FN are $N$ -functions on X, called the negative truth membership function, the negative indeterminacy membership function and the negative falsity membership function, respectively.

Every neutrosophic $N$ -structure XN over X satisfies the condition $(∀x∈X)(−3≤TN(x)+IN(x)+FN(x)≤0).$

Definition 2.7. [13] Let XN be a neutrosophic $N$ -structure on a set X and $τ,γ,ρ$ be any elements of [ −1, 0] such that $−3≤τ+γ+ρ≤0$ . Consider the following sets:

$TNτ:={x∈X:TN(x)≤τ},INγ:={x∈X:IN(x)≥γ}$

and

$FNρ:={x∈X:FN(x)≤ρ}.$

The set

$XN(τ,γ,ρ):={x∈X:TN(x)≤τ,IN(x)≥γ and TN(x)≤ρ}$

is called the $(τ,γ,ρ)$ -level set of XN. Moreover, $XN(τ,γ,ρ)=TNτ∩INγ∩FNρ$ .

Consider sets

$XNct:={x∈X:TN(x)≤TN(ct)},XNci:={x∈X:IN(x)≥IN(ci)}$

and

$XNcf:={x∈X:FN(x)≤FN(cf)},$

for any $ct,ci,cf∈X$ . Obviously, $ct∈XNct,ci∈XNci$ and $cf∈XNcf$ [13].

3 Neutrosophic $N$ -Structures

In this section, neutrosophic $N$ -subalgebras and neutrosophic $N$ -filters on Sheffer stroke BL-algebras. Unless otherwise specified, C denotes a Sheffer stroke BL-algebra.

Definition 3.1. A neutrosophic $N$ -structure CN on a Sheffer stroke BL-algebra C is called a neutrosophic $N$ -subalgebra of C if the following condition is valid:

$min{TN(c1),TN(c2)}≤TN(c1∣(c2∣c2)),max{IN(c1),IN(c2)}≥IN(c1∣(c2∣c2)) andmax{FN(c1),FN(c2)}≥FN(c1∣(c2∣c2)),$ (1)

for all $c1,c2∈C$ .

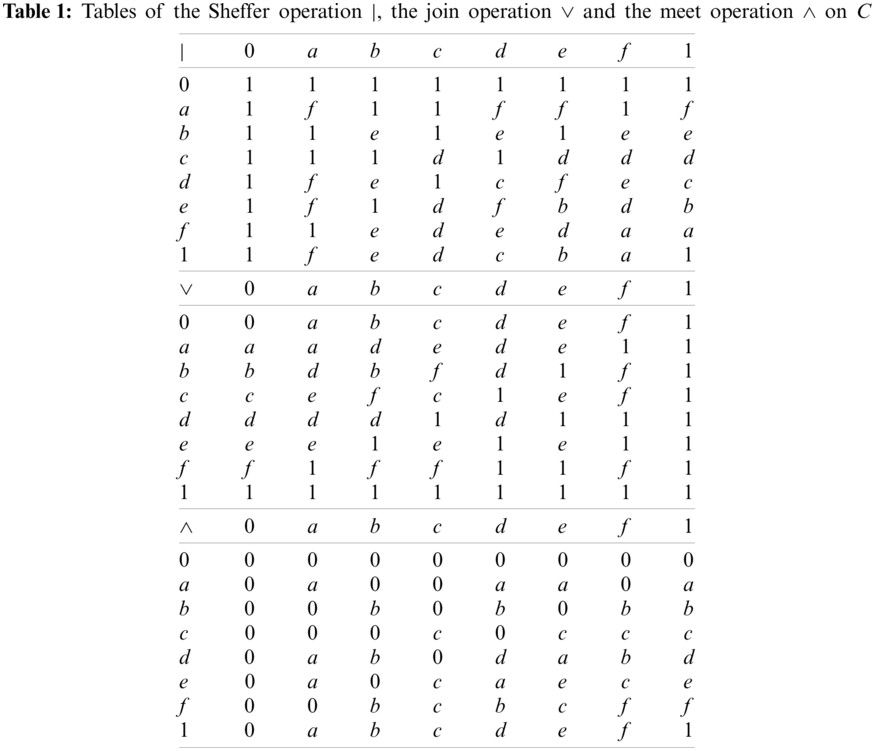
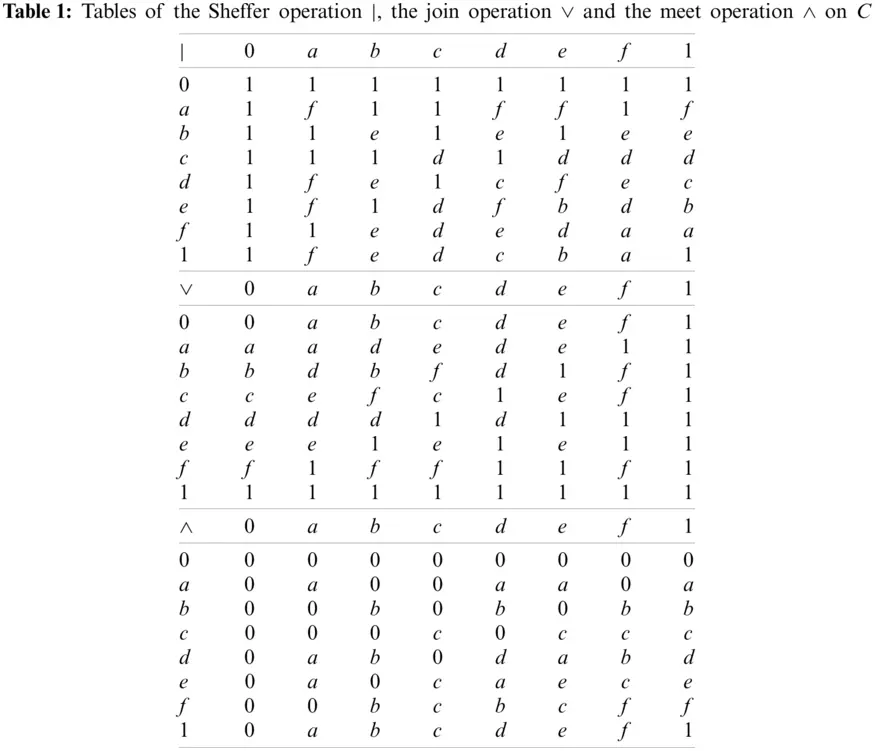
Example 3.1. Consider a Sheffer stroke BL-algebra C where the set $C={0,a,b,c,d,e,f,1}$ and the Sheffer operation $∣$ , the join operation $∨$ and the meet operation $∧$ on C has the Cayley tables in Tab. 1 [21]. Then a neutrosophic $N$ -structure

$CN={x(−0.08,−0.999,−0.26):x=d,1}∪{x(−0.92,−0.52,−0.0012):x∈C−{d,1}}$

on C is a neutrosophic $N$ -subalgebra of C.

images

Definition 3.2. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C and $τ,γ,ρ$ be any elements of [ −1, 0] such that $−3≤τ+γ+ρ≤0$ . For the sets

$TNτ:={c∈C:TN(c)≥τ},INγ:={c∈C:IN(c)≤γ}$

and

$FNρ:={c∈C:FN(c)≤ρ},$

the set

$CN(τ,γ,ρ):={c∈C:TN(c)≥τ,IN(c)≤γ and FN(c)≤ρ}$

is called the $(τ,γ,ρ)$ -level set of CN. Moreover, $CN(τ,γ,ρ)=TNτ∩INγ∩FNρ$ .

Definition 3.3. A subset D of a Sheffer stroke BL-algebra C is called a quasi-subalgebra of C if $c1∣(c2∣c2)∈D$ , for all $c1,c2∈D$ . Obviously, C itself and ${1}$ are quasi-subalgebras of C.

Example 3.2. Consider the Sheffer stroke BL-algebra C in Example 3.1. Then ${0,a,f,1}$ is a quasi-subalgebra of C.

Theorem 3.1. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C and $τ,γ,ρ$ be any elements of [ −1, 0] such that $−3≤τ+γ+ρ≤0$ . If CN is a neutrosophic $N$ -subalgebra of C, then the nonempty level set $CN(τ,γ,ρ)$ of CN is a quasi-subalgebra of C.

Proof. Let CN be a neutrosophic $N$ -subalgebra of C and c1, c2 be any elements of $CN(τ,γ,ρ)$ , for $τ,γ,ρ∈[−1,0]$ with $−3≤τ+γ+ρ≤0$ . Then $TN(c1),TN(c2)≥τ,IN(c1),IN(c2)≤γ$ and $FN(c1),FN(c2)≤ρ$ . Since

$τ≤min{TN(c1),TN(c2)}≤TN(c1∣(c2∣c2)),IN(c1∣(c2∣c2))≤max{IN(c1),IN(c2)}≤γ$

and

$FN(c1∣(c2∣c2))≤max{FN(c1),FN(c2)}≤ρ,$

for all $c1,c2∈C$ , we obtain that $c1∣(c2∣c2)∈TNτ$ , $c1∣(c2∣c2)∈INγ$ and $c1∣(c2∣c2)∈FNρ$ , and so, $c1∣(c2∣c2)∈TNτ∩INγ∩FNρ=CN(τ,γ,ρ)$ . Hence, $CN(τ,γ,ρ)$ is a quasi-subalgebra of C.

Theorem 3.2. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C and $TNτ,INγ$ and $FNρ$ be quasi-subalgebras of C, for all $τ,γ,ρ∈[−1,0]$ with $−3≤τ+γ+ρ≤0$ . Then CN is a neutrosophic $N$ -subalgebra of C.

Proof. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C, and $TNτ,INγ$ and $FNρ$ be quasi-subalgebras of C, for all $τ,γ,ρ∈[−1,0]$ with $−3≤τ+γ+ρ≤0$ . Suppose that c1 and c2 be any elements of C such that $w1=TN(c1∣(c2∣c2))<min{TN(c1),TN(c2)}=w2$ , $c1$ and $c1$ . If $τ1=12(w1+w2)∈[−1,0)$ , $γ1=12(t1+t2)∈[−1,0)$ and $ρ1=12(r1+r2)∈[−1,0)$ , then , and . Thus, $c1,c2∈TNτ1$ , $c1,c2∈INγ1$ and $c1,c2∈FNρ1$ but $c1∣(c2∣c2)∉TNτ1$ , $c1∣(c2∣c2)∉INγ1$ and $c1∣(c2∣c2)∉FNρ1$ , which are contradictions. Hence, $min{TN(c1),TN(c2)}≤TN(c1∣(c2∣c2))$ , $IN(c1∣(c2∣c2))≤max{IN(c1),IN(c2)}$ and $FN(c1∣(c2∣c2))≤max{FN(c1),FN(c2)}$ , for all $c1,c2∈C$ . Thereby, CN is a neutrosophic $N$ -subalgebra of C.

Theorem 3.3. Let ${CNi:i∈N}$ be a family of all neutrosophic $N$ -subalgebras of a Sheffer stroke BL-algebra C. Then ${CNi:i∈N}$ forms a complete distributive lattice.

Proof. Let D be a nonempty subset of ${CNi:i∈N}$ . Since CNi is a neutrosophic $N$ -subalgebra of C, for all $i∈N$ , it satisfies the condition (1). Then $⋂D$ satisfies the condition (1). Thus, $⋂D$ is a neutrosophic $N$ -subalgebra of C. Let E be a family of all neutrosophic $N$ -subalgebras of C containing $⋃{CNi:i∈N}$ . Thus, $⋂E$ is also a neutrosophic $N$ -subalgebra of C. If $⋀i∈NCNi=⋂i∈NCNi$ and $⋁i∈NCNi=⋂E$ , then $({CNi:i∈N},⋁,⋀)$ forms a complete lattice. Also, it is distibutive by the definitions of $⋁$ and $⋀$ .

Lemma 3.1. Let CN be a neutrosophic $N$ -subalgebra of a Sheffer stroke BL-algebra C. Then $TN(c)≤TN(1)$ , $IN(c)≥IN(1)$ and $FN(c)≥FN(1)$ , for all $c∈C$ .

Proof. Let CN be a neutrosophic $N$ -subalgebra of C. Then it follows from Poposition 2.1 (2) that

$TN(c)=min{TN(c),TN(c)}≤TN(c∣(c∣c))=TN(1),IN(1)=IN(c∣(c∣c))≤max{IN(c),IN(c)}=IN(c)$

and

$FN(1)=FN(c∣(c∣c))≤max{FN(c),FN(c)}=FN(c),$

for all $c∈C$ .

The inverse of Lemma 3.1 is not true in general.

Example 3.3. Consider the Sheffer stroke BL-algebra C in Example 3.1. Then a neutrosophic $N$ -structure

$CN={x(−0.01,−0.1,−0.11):x=a,b,1}∪{x(−0.1,−0.01,−0.01):x∈C−{a,b,1}}$

on C is not a neutrosophic $N$ -subalgebra of C since $max{FN(a),FN(b)}=−0.11<−0.01=FN(f)=FN(a∣(b∣b))$ .

Lemma 3.2. A neutrosophic $N$ -subalgebra CN of a Sheffer stroke BL-algebra C satisfies $TN(c1)≤TN(c1∣(c2∣c2)),$ $IN(c1)≥IN(c1∣(c2∣c2))$ and $FN(c1)≥FN(c1∣(c2∣c2)),$ for all $c1,c2∈C$ if and only if TN, IN and FN are constant.

Proof. Let CN be a a neutrosophic $N$ -subalgebra of C such that $TN(c1)≤TN(c1∣(c2∣c2)),$ $IN(c1)≥IN(c1∣(c2∣c2))$ and $FN(c1)≥FN(c1∣(c2∣c2)),$ for all $c1,c2∈C$ . Since $TN(1)≤TN(1∣(c∣c))=TN(c),$ $IN(1)≥IN(1∣(c∣c))=IN(c)$ and $FN(1)≥FN(1∣(c∣c))=FN(c)$ from Proposition 2.1 (3), it is obtained from Lemma 3.1 that TN(c) = TN(1), IN(c) = IN(1) and FN(c) = FN(1), for all $c∈C$ . Hence, TN, IN and FN are constant.

Conversely, it is obvious since TN, IN and FN are constant.

Definition 3.4. A neutrosophic $N$ -structure CN on a Sheffer stroke BL-algebra C is called a neutrosophic $N$ -filter of C if

1. $c1≤c2$ implies $TN(c1)≤TN(c2),$ $IN(c2)≤IN(c1)$ and $FN(c2)≤FN(c1),$

2. $min{TN(c1),TN(c2)}≤TN((c1∣c2)∣(c1∣c2)),$ $IN((c1∣c2)∣(c1∣c2))≤max{IN(c1),IN(c2)}$ and $FN((c1∣c2)∣(c1∣c2))≤max{FN(c1),FN(c2)},$

for all $c1,c2∈C$ .

Example 3.4. Consider the Sheffer stroke BL-algebra C in Example 3.1. Then a neutrosophic $N$ -structure

$CN={x(−0.3,−1,−0.15):x=c,e,f,1}∪{x(−1,−0.7,0):x=0,a,b,d}$

on C is a neutrosophic $N$ -filter of C.

Theorem 3.4. Let CN be a a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C. Then CN is a neutrosophic $N$ -filter of C if and only if

$min{TN(c1),TN(c1∣(c2∣c2))}≤TN(c2)≤TN(1),IN(1)≤IN(c2)≤max{IN(c1),IN(c1∣(c2∣c2))} andFN(1)≤FN(c2)≤max{FN(c1),FN(c1∣(c2∣c2))},$ (2)

for all $c1,c2∈C$ .

Proof. Let CN be a neutrosophic $N$ -filter of C. Then it follows from (sBL-3) and Definition 3.4 that

$min{TN(c1),TN(c1∣(c2∣c2))}≤TN((c1∣(c1∣(c2∣c2)))∣(c1∣(c1∣(c2∣c2))))=TN(c1∧c2)≤TN(c2)≤TN(1),IN(1)≤IN(c2)≤IN(c1∧c2)=IN((c1∣(c1∣(c2∣c2)))∣(c1∣(c1∣(c2∣c2))))≤max{IN(c1),IN(c1∣(c2∣c2))}$

and

$FN(1)≤FN(c2)≤FN(c1∧c2)=FN((c1∣(c1∣(c2∣c2)))∣(c1∣(c1∣(c2∣c2))))≤max{FN(c1),FN(c1∣(c2∣c2))},$

for all $c1,c2∈C$ .

Conversely, let CN be a a neutrosophic $N$ -structure on C satisfying the condition (2). Assume that $c1≤c2$ . Then $c1∣(c2∣c2)=1$ from Proposition 2.1 (7). Thus,

$TN(c1)=min{TN(c1),TN(1)}=min{TN(c1),TN(c1∣(c2∣c2))}≤TN(c2),IN(c2)≤max{IN(c1),IN(c1∣(c2∣c2))}=max{IN(c1),IN(1)}=IN(c1)$

and

$FN(c2)≤max{FN(c1),FN(c1∣(c2∣c2))}=max{FN(c1),FN(1)}=FN(c1),$

for all $c1,c2∈C$ . Also, it follows from Proposition 2.1 (9), (S1) and (S2) that

$min{TN(c1),TN(c2)}≤min{TN(c1),TN(c1∣(c1∣c2))}=min{TN(c1),TN(c1∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2))))}≤TN((c1∣c2)∣(c1∣c2)),$

$IN((c1∣c2)∣(c1∣c2))≤max{IN(c1),IN(c1∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2))))}=max{IN(c1),IN(c1∣(c1∣c2))}≤max{IN(c1),IN(c2)}$

and

$FN((c1∣c2)∣(c1∣c2))≤max{FN(c1),FN(c1∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2))))}=max{FN(c1),FN(c1∣(c1∣c2))}≤max{FN(c1),FN(c2)},$

for all $c1,c2∈C$ . Thus, CN is a neutrosophic $N$ -filter of C.

Corollary 3.1. Let CN be a neutrosophic $N$ -filter of a Sheffer stroke BL-algebra C. Then

1. $min{TN(c3),TN(c3∣(((c2∣(c1∣c1))∣(c1∣c1))∣((c2∣(c1∣c1))∣(c1∣c1))))}≤TN((c1∣(c2∣c2))∣(c2∣c2)),$ $IN((c1∣(c2∣c2))∣(c2∣c2))≤max{IN(c3),IN(c3∣(((c2∣(c1∣c1))∣(c1∣c1))∣((c2∣(c1∣c1))∣(c1∣c1))))}$ and $FN((c1∣(c2∣c2))∣(c2∣c2))≤max{FN(c3),FN(c3∣(((c2∣(c1∣c1))∣(c1∣c1))∣((c2∣(c1∣c1))∣(c1∣c1))))}$ ,

2. $min{TN(c3),TN(c3∣((c1∣(c2∣c2))∣∣(c1∣(c2∣c2))))}≤TN(c1∣(c2∣c2)),$ $IN(c1∣(c2∣c2))≤max{IN(c3),IN(c3∣((c1∣(c2∣c2))∣(c1∣(c2∣c2))))} and$ $FN(c1∣(c2∣c2))≤max{FN(c3),FN(c3∣((c1∣(c2∣c2))∣(c1∣(c2∣c2))))}$ ,

3. $min{TN(c1∣((c2∣(c3∣c3))∣∣(c2∣(c3∣c3)))),TN(c1∣(c2∣c2))}≤TN(c1∣(c3∣c3)),$ $IN(c1∣(c3∣c3))≤max{IN(c1∣((c2∣(c3∣c3))∣(c2∣(c3∣c3)))),IN(c1∣(c2∣c2))} and$ $FN(c1∣(c3∣c3))≤max{FN(c1∣((c2∣(c3∣c3))∣(c2∣(c3∣c3)))),FN(c1∣(c2∣c2))}$ ,

4. $TN(c1∣(c2∣c2))=TN(1)$ , $IN(c1∣(c2∣c2))=IN(1)$ and $FN(c1∣(c2∣c2))=FN(1)$ imply $TN(c1)≤TN(c2)$ , $IN(c2)≤IN(c1)$ and $FN(c2)≤FN(c1)$ ,

for all $c1,c2,c3∈C$ .

Proof. It is proved from Theorem 3.4, Lemma 2.1 and Lemma 2.2.

Lemma 3.3. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C. Then CN is a neutrosophic $N$ -filter of C if and only if

$c1≤c2∣(c3∣c3) implies (min{TN(c1),TN(c2)}≤TN(c3),IN(c3)≤max{IN(c1),IN(c2)} andFN(c3)≤max{FN(c1),FN(c2)},)$ (3)

for all $c1,c2,c3∈C$ .

Proof. Let CN be a neutrosophic $N$ -filter of C and $c1≤c2∣(c3∣c3)$ . Then it is obtained from Definition 3.4 (1) and Theorem 3.4 that

$min{TN(c1),TN(c2)}≤min{TN(c2),TN(c2∣(c3∣c3))}≤TN(c3),IN(c3)≤max{IN(c2),IN(c2∣(c3∣c3))}≤max{IN(c1),IN(c2)}$

and

$FN(c3)≤max{FN(c2),FN(c2∣(c3∣c3))}≤max{FN(c1),FN(c2)},$

for all $c1,c2,c3∈C$ .

Conversely, let CN be a neutrosophic $N$ -structure on C satisfying the condition (3). Since it is known from Proposition 2.1 (4) that $c≤1=c∣(1∣1)$ , for all $c∈C$ , we get that $TN(c)=min{TN(c),TN(c)}≤TN(1),$ ${IN(1)≤max{IN(c),IN(c)}=IN(c)}$ and ${FN(1)≤max{FN(c),FN(c)}=FN(c)}$ , for all $c∈C$ . Suppose that $c1≤c2$ . Since we have $c1≤c2=1∣(c2∣c2)$ from Proposition 2.1 (3), it is obtained that $TN(c1)=min{TN(c1),TN(1)}≤TN(c2),$ $IN(c2)≤max{IN(c1),IN(1)}=IN(c1)$ and $FN(c2)≤max{FN(c1),FN(1)}=FN(c1)$ . Since $c1≤(c1∣c2)∣c2=c2∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2)))$ from Proposition 2.1 (9), (S1) and (S2), it follows that

$min{TN(c1),TN(c2)}≤TN((c1∣c2)∣(c1∣c2)),IN((c1∣c2)∣(c1∣c2))≤max{IN(c1),IN(c2)}$

and

$FN((c1∣c2)∣(c1∣c2))≤max{FN(c1),FN(c2)},$

for all $c1,c2∈C$ . Thus, CN is a neutrosophic $N$ -filter of C.

Lemma 3.4. Every neutrosophic $N$ -filter of a Sheffer stroke BL-algebra C is a neutrosophic $N$ -subalgebra of C.

Proof. Let CN be a neutrosophic $N$ -filter of C. Since

$((c1∣c2)∣(c1∣c2))∣((c1∣(c2∣c2))∣(c1∣(c2∣c2)))=c1∣((((c1∣c2)∣(c1∣c2))∣(c2∣c2))∣(((c1∣c2)∣(c1∣c2))∣(c2∣c2)))=c1∣((c1∣((c2∣(c2∣c2))∣(c2∣(c2∣c2))))∣(c1∣((c2∣(c2∣c2))∣(c2∣(c2∣c2)))))=c1∣((c1∣(1∣1))∣(c1∣(1∣1)))=c1∣(1∣1)=1$

from Proposition 2.1 (1), (2), (4) and (S3), it follows from Proposition 2.1 (7) that $(c1∣c2)∣(c1∣c2)≤c1∣(c2∣c2)$ , for all $c1,c2∈C$ . Then

$min{TN(c1),TN(c2)}≤TN((c1∣c2)∣(c1∣c2))≤TN(c1∣(c2∣c2)),IN(c1∣(c2∣c2))≤IN((c1∣c2)∣(c1∣c2))≤max{IN(c1),IN(c2)}$

and

$FN(c1∣(c2∣c2))≤FN((c1∣c2)∣(c1∣c2))≤max{FN(c1),FN(c2)},$

for all $c1,c2∈C$ . Thereby, CN is a neutrosophic $N$ -subalgebra of C.

The inverse of Lemma 3.4 is usually not true.

Example 3.5. Consider the Sheffer stroke BL-algebra C in Example 3.1. Then a neutrosophic $N$ -structure

$CN={0(−1,0,0),1(0,−1,−1)}∪{x(−0.5,−0.5,−0.5):x∈C−{0,1}}$

on C is a neutrosophic $N$ -subalgebra of C whereas it is not a neutrosophic $N$ -filter of C since $min{TN(a),TN(b)}=−0.5>−1=TN((a∣b)∣(a∣b))$ .

Definition 3.5. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C. Then an ultra neutrosophic $N$ -filter CN of C is a neutrosophic $N$ -filter of C satisfying TN(c) = TN(1), IN(c) = IN(1), FN(c) = FN(1) or $TN(c∣c)=TN(1)$ , $IN(c∣c)=IN(1)$ , $FN(c∣c)=FN(1)$ , for all $c∈C$ .

Example 3.6. Consider the Sheffer stroke BL-algebra C in Example 3.1. Then a neutrosophic $N$ -structure

$CN={x(−0.02,−0.77,−0.6):x=b,d,f,1}∪{x(−0.79,−0.05,−0.41):x=0,a,c,e}$

on C is an ultra neutrosophic $N$ -filter of C.

Remark 3.1. By Definition 3.5, every ultra neutrosophic $N$ -filter of a Sheffer stroke BL-algebra C is a neutrosophic $N$ -filter of C but the inverse does not generally hold.

Example 3.7. Consider the Sheffer stroke BL-algebra C in Example 3.1. Then a neutrosophic $N$ -filter

$CN={x(−0.18,−0.82,−0.57): x=e,1}∪{x(−1,−0.64,−0.43):x∈C−{e,1}}$

of C is not ultra since $TN(a)≠TN(1)≠TN(a∣a)=TN(f)$ , $IN(a)≠IN(1)≠IN(a∣a)=IN(f)$ and $FN(a)≠FN(1)≠TFN(a∣a)=FN(f)$ .

Lemma 3.5. Let CN be a neutrosophic $N$ -filter of a Sheffer stroke BL-algebra C. Then CN is an ultra neutrosophic $N$ -filter of C if and only if $TN(c1)≠TN(1),TN(c2)≠TN(1),$ $IN(c1)≠IN(1),IN(c2)≠IN(1)$ and $FN(c1)≠FN(1),FN(c2)≠FN(1)$ imply $TN(c1∣(c2∣c2))=TN(1)=TN(c2∣(c1∣c1)),$ $IN(c1∣(c2∣c2))=IN(1)=IN(c2∣(c1∣c1))$ and $FN(c1∣(c2∣c2))=FN(1)=FN(c2∣(c1∣c1)),$ for all $c1,c2∈C$ .

Proof. Let CN be an ultra neutrosophic $N$ -filter of C, and $TN(c1)≠TN(1),TN(c2)≠TN(1),$ $IN(c1)≠IN(1),IN(c2)≠IN(1)$ and $FN(c1)≠FN(1),FN(c2)≠FN(1)$ , for any $c1,c2∈C$ . Then $TN(c1∣c1)=TN(1)=TN(c2∣c2),$ $IN(c1∣c1)=IN(1)=IN(c2∣c2)$ and $FN(c1∣c1)=FN(1)=FN(c2∣c2)$ . Since

$(c1∣c1)∣((c1∣(c2∣c2))∣(c1∣(c2∣c2)))=(c2∣c2)∣((c1∣(c1∣c1))∣(c1∣(c1∣c1)))=(c2∣c2)∣(1∣1)=1$

and

$(c2∣c2)∣((c2∣(c1∣c1))∣(c2∣(c1∣c1)))=(c1∣c1)∣((c2∣(c2∣c2))∣(c2∣(c2∣c2)))=(c1∣c1)∣(1∣1)=1$

from (S1), (S3), Proposition 2.1 (2) and (4), it follows from Theorem 3.4 that

$TN(1)=min{TN(1),TN(1)}=min{TN(c1∣c1),TN((c1∣c1)∣((c1∣(c2∣c2))∣(c1∣(c2∣c2))))}≤TN(c1∣(c2∣c2)),IN(c1∣(c2∣c2))≤max{IN(c1∣c1),IN((c1∣c1)∣((c1∣(c2∣c2))∣(c1∣(c2∣c2))))}=max{IN(1),IN(1)}=IN(1),FN(c1∣(c2∣c2))≤max{FN(c1∣c1),FN((c1∣c1)∣((c1∣(c2∣c2))∣(c1∣(c2∣c2))))}=max{FN(1),FN(1)}=FN(1),$

and similarly, $TN(1)≤TN(c2∣(c1∣c1)),$ $IN(c2∣(c1∣c1))≤IN(1),$ $FN(c2∣(c1∣c1))≤FN(1).$ Hence, we obtain from Theorem 3.4 that $TN(c1∣(c2∣c2))=TN(1)=TN(c2∣(c1∣c1)),$ $IN(c1∣(c2∣c2))=IN(1)=IN(c2∣(c1∣c1))$ and $FN(c1∣(c2∣c2))=FN(1)=FN(c2∣(c1∣c1)),$ for all $c1,c2∈C$ .

Conversely, let CN be a neutrosophic $N$ -filter of C such that $TN(c1)≠TN(1),TN(c2)≠TN(1),$ $IN(c1)≠IN(1),IN(c2)≠IN(1)$ and $FN(c1)≠FN(1),FN(c2)≠FN(1)$ imply $TN(c1∣(c2∣c2))=TN(1)=TN(c2∣(c1∣c1)),$ $IN(c1∣(c2∣c2))=IN(1)=IN(c2∣(c1∣c1))$ and $FN(c1∣(c2∣c2))=FN(1)=FN(c2∣(c1∣c1)),$ for all $c1,c2∈C$ . Assume that $TN(c)≠TN(1)≠TN(0)=TN(1∣1),$ $IN(c)≠IN(1)≠IN(0)=IN(1∣1)$ and $FN(c)≠FN(1)≠FN(0)=FN(1∣1)$ . Hence, $TN(c∣c)=TN(1∣((c∣c)∣(c∣c)))=TN(c∣1)=TN(c∣((1∣1)∣(1∣1)))=TN(1),$ $TN((1∣1)∣(c∣c))=TN(1)$ , $IN(c∣c)=IN(1∣((c∣c)∣(c∣c)))=IN(c∣1)=IN(c∣((1∣1)∣(1∣1)))=IN(1),$ $IN((1∣1)∣(c∣c))=IN(1)$ and $FN(c∣c)=FN(1∣((c∣c)∣(c∣c)))=FN(c∣1)=FN(c∣((1∣1)∣(1∣1)))=FN(1),$ $FN((1∣1)∣(c∣c))=FN(1)$ from Proposition 2.1 (3), (4), (S1) and (S2). Suppose that $TN(c∣c)≠TN(1)≠TN(0)=TN(1∣1),$ $IN(c)≠IN(1)≠IN(0)=IN(1∣1)$ and $FN(c)≠FN(1)≠FN(0)=FN(1∣1)$ . Thus, $TN(c)=TN(1∣(c∣c))=TN((c∣c)∣((1∣1)∣(1∣1)))=TN(1),$ $TN((1∣1)∣((c∣c)∣(c∣c)))=TN(1)$ , $IN(c)=IN(1∣(c∣c))=IN((c∣c)∣((1∣1)∣(1∣1)))=IN(1),$ $IN((1∣1)∣((c∣$ $c)∣(c∣c)))=IN(1)$ and $FN(c)=FN(1∣(c∣c))=FN((c∣c)∣((1∣1)∣(1∣1)))=FN(1),$ $FN((1∣1)∣((c∣c)∣(c∣c)))=FN(1)$ from Proposition 2.1 (3), (4), (S1) and (S2). Therefore, CN is an ultra neutrosophic $N$ -filter of C.

Lemma 3.6. Let CN be a neutrosophic $N$ -filter of a Sheffer stroke BL-algebra C. Then CN is an ultra neutrosophic $N$ -filter of C if and only if $TN(c1∨c2)≤TN(c1)∨TN(c2),$ $IN(c1)∨IN(c2)≤IN(c1∨c2)$ and $FN(c1)∨FN(c2)≤FN(c1∨c2)$ , for all $c1,c2∈C$ .

Proof. Let CN be an ultra neutrosophic $N$ -filter of C. If TN(c1) = TN(1), IN(c1) = IN(1), FN(c1) = FN(1) or TN(c2) = TN(1), IN(c2) = IN(1), FN(c2) = FN(1), then the proof is completed from Theorem 3.4. Assume that $TN(c1)≠TN(1)≠TN(c2),IN(c1)≠IN(1)≠IN(c2)$ and $FN(c1)≠FN(1)≠FN(c2)$ . Thus, we have from Lemma 3.5 that $TN(c1∣(c2∣c2))=TN(1)=TN(c2∣(c1∣c1)),$ $IN(c1∣(c2∣c2))=IN(1)=IN(c2∣(c1∣c1))$ and $FN(c1∣(c2∣c2))=FN(1)=FN(c2∣(c1∣c1)),$ for all $c1,c2∈C$ . Since

$TN(c1∨c2)=min{TN(1),TN(c1∨c2)}=min{TN(c1∣(c2∣c2)),TN((c1∣(c2∣c2))∣(c2∣c2))}≤TN(c2),IN(c2)≤max{IN(c1∣(c2∣c2)),IN((c1∣(c2∣c2))∣(c2∣c2))}=max{IN(1),IN(c1∨c2)}=IN(c1∨c2),FN(c2)≤max{FN(c1∣(c2∣c2)),FN((c1∣(c2∣c2))∣(c2∣c2))}=max{FN(1),IN(c1∨c2)}=FN(c1∨c2),$

and similarly, $TN(c1∨c2)=TN(c2∨c1)≤TN(c1),$ $IN(c1)≤IN(c2∨c1)=IN(c1∨c2),$ $FN(c1)≤FN(c2∨c1)=FN(c1∨c2)$ from Corollary 2.1 and Theorem 3.4, it follows that $TN(c1∨c2)≤TN(c1)∨TN(c2),$ $IN(c1)∨IN(c2)≤IN(c1∨c2)$ and $FN(c1)∨FN(c2)≤FN(c1∨c2)$ , for all $c1,c2∈C$ .

Conversely, let CN be a neutrosophic $N$ -filter of C satisfying that $TN(c1∨c2)≤TN(c1)∨TN(c2),$ $IN(c1)∨IN(c2)≤IN(c1∨c2)$ and $FN(c1)∨FN(c2)≤FN(c1∨c2)$ , for any $c1,c2∈C$ . Since

$TN(1)=TN(c∣(c∣c))=TN((c∣((c∣c)∣(c∣c)))∣((c∣c)∣(c∣c)))=TN(c∨(c∣c))≤TN(c)∨TN(c∣c),IN(c)∨IN(c∣c)≤IN(c∨(c∣c))=IN((c∣((c∣c)∣(c∣c)))∣((c∣c)∣(c∣c)))=IN(c∣(c∣c))=IN(1)$

and

$FN(c)∨FN(c∣c)≤FN(c∨(c∣c))=FN((c∣((c∣c)∣(c∣c)))∣((c∣c)∣(c∣c)))=FN(c∣(c∣c))=FN(1)$

from Proposition 2.1 (2), (S1), (S2) and Corollary 2.1, it is obtained from Theorem 3.4 that $TN(c)∨TN(c∣c)=TN(1)$ , $IN(c)∨IN(c∣c)=IN(1)$ and $FN(c)∨FN(c∣c)=FN(1)$ , and so, TN(c) = TN(1), IN(c) = IN(1), FN(c) = FN(1) or $TN(c∣c)=TN(1)$ , $IN(c∣c)=IN(1)$ , $FN(c∣c)=FN(1)$ , for all $c∈C$ . Thus, CN is an ultra neutrosophic $N$ -filter of C.

Theorem 3.5. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C and $τ,γ,ρ$ be any elements of [ −1, 0] with $−3≤τ+γ+ρ≤0$ . If CN is a (ultra) neutrosophic $N$ -filter of C, then the nonempty subset $CN(τ,γ,ρ)$ is a (ultra) filter of C.

Proof. Let CN be a neutrosophic $N$ -filter of C and $CN(τ,γ,ρ)≠∅$ , for $τ,γ,ρ∈[−1,0]$ with $−3≤τ+γ+ρ≤0$ . Asumme that $c1,c2∈CN(τ,γ,ρ)$ . Since $τ≤TN(c1),τ≤TN(c2),$ $IN(c1)≤γ,IN(c2)≤γ$ , $FN(c1)≤ρ$ and $FN(c2)≤ρ$ , it follows that

$τ≤min{TN(c1),TN(c2)}≤TN((c1∣c2)∣(c1∣c2)),IN((c1∣c2)∣(c1∣c2))≤max{IN(c1),IN(c2)}≤γ$

and

$FN((c1∣c2)∣(c1∣c2))≤max{FN(c1),fN(c2)}≤ρ.$

Then $(c1∣c2)∣(c1∣c2)∈TNτ,INγ,FNρ$ , and so, $(c1∣c2)∣(c1∣c2)∈CN(τ,γ,ρ)$ . Suppose that $c1∈CN(τ,γ,ρ)$ and $c1≤c2$ . Since $τ≤TN(c1)≤TN(c2),$ $IN(c2)≤IN(c1)≤γ$ and $FN(c2)≤FN(c1)≤ρ$ , we have that $c2∈TNτ,INγ,FNρ$ , and so, $c2∈CN(τ,γ,ρ)$ . Hence, $CN(τ,γ,ρ)$ is a filter of C. Moreover, let CN be an ultra neutrosophic $N$ -filter of C. Assume that $c1∨c2∈CN(τ,γ,ρ)$ . Since $τ≤TN(c1∨c2)$ , $IN(c1∨c2)≤γ$ and $FN(c1∨c2)≤ρ$ , it is obtained from Lemma 3.6 that $τ≤TN(c1∨c2)≤TN(c1)∨TN(c2)$ , $IN(c1)∨IN(c2)≤IN(c1∨c2)≤γ$ and $FN(c1)∨FN(c2)≤FN(c1∨c2)≤ρ$ , for all $c1,c2∈C$ . Thus, $τ≤TN(c1)$ , $IN(c1)≤γ$ , $FN(c2)≤ρ$ or $τ≤TN(c2)$ , $IN(c2)≤γ$ , $FN(c2)≤ρ$ , and so, $c1∈CN(τ,γ,ρ)$ or $c2∈CN(τ,γ,ρ)$ . By Lemma 2.3, $CN(τ,γ,ρ)$ is an ultra filter of C.

Theorem 3.6. Let CN be a neutrosophic $N$ -structure on a Sheffer stroke BL-algebra C, and $TNτ,INγ$ and $FNρ$ be (ultra) filters of C, for all $τ,γ,ρ∈[−1,0]$ with $−3≤τ+γ+ρ≤0$ . Then CN is a (ultra) neutrosophic $N$ -filter of C.

Proof. Let CN be a neutrosophic $N$ -structure on C, and $TNτ,INγ$ and $FNρ$ be filters of C, for all $τ,γ,ρ∈[−1,0]$ with $−3≤τ+γ+ρ≤0$ . Assume that

$τ1=TN((c1∣c2)∣(c1∣c2))<min{TN(c1),TN(c2)}=τ2,γ1=max{IN(c1),IN(c2)}<IN((c1∣c2)∣(c1∣c2))=γ2$

and

$ρ1=max{FN(c1),fN(c2)}<FN((c1∣c2)∣(c1∣c2))=ρ2,$

for some $c1,c2∈C$ . If $τ0=12(τ1+τ2)$ , $γ0=12(γ1+γ2)$ , $ρ0=12(ρ1+ρ2)∈[−1,0)$ , then $τ1<τ0<τ2$ , $γ1<γ0<γ2$ and $ρ1<ρ0<ρ2$ . So, $(c1∣c2)∣(c1∣c2)∉TNτ0,INγ0,FNρ0$ when $c1,c2∈TNτ0,INγ0,FNρ0$ , which contradict with (SF-1). Thus

$min{TN(c1),TN(c2)}≤TN((c1∣c2)∣(c1∣c2)),IN((c1∣c2)∣(c1∣c2))≤max{IN(c1),IN(c2)}$

and

$FN((c1∣c2)∣(c1∣c2))≤max{FN(c1),fN(c2)},$

for all $c1,c2∈C$ . Let $c1≤c2$ . Suppose that TN(c2) < TN(c1), IN(c1) < IN(c2) and FN(c1) < FN(c2), for some $c1,c2∈C$ . If $τ∗=12(TN(c1)+TN(c2))$ , $γ∗=12(IN(c1)+IN(c2))$ , $ρ∗=12(FN(c1)+FN(c2))∈[−1,0)$ , then $c2$ , $c1$ and $c1$ Hence, $c1∈TNτ∗,INγ∗,FNρ∗$ but $c2∉TNτ∗,INγ∗,FNρ∗$ which is a contradiction with (SF-2). Therefore, $TN(c1)≤TN(c2)$ , $IN(c2)≤IN(c1)$ and $FN(c2)≤FN(c1),$ for all $c1,c2∈C$ . Thereby, CN is a neutrosophic $N$ -filter of C.

Also, let $TNτ,INγ$ and $FNρ$ be ultra filters of C, for all $τ,γ,ρ∈[−1,0]$ with $−3≤τ+γ+ρ≤0$ , and $TN(c1∨c2)=τ$ , $IN(c1∨c2)=γ$ and $FN(c1∨c2)=ρ$ . Since $c1∨c2∈TNτ,INγ,FNρ$ , it follows from Lemma 2.3 that $c1∈TNτ,INγ,FNρ$ or $c2∈TNτ,INγ,FNρ$ . Thus, $TN(c1∨c2)=τ≤TN(c1),TN(c2)$ , $IN(c1),IN(c2)≤γ=IN(c1∨c2)$ and $FN(c1),FN(c2)≤ρ=FN(c1∨c2)$ , and so, $TN(c1∨c2)≤TN(c1)∨TN(c2)$ , $IN(c1)∨IN(c2)≤IN(c1∨c2)$ and $FN(c1)∨FN(c2)≤FN(c1∨c2)$ , for all $c1,c2∈C$ . By Lemma 3.6, CN is an ultra neutrosophic $N$ -filter of C.

Definition 3.6. Let C be a Sheffer stroke BL-algebra. Define

$CNct:={c∈C:TN(ct)≤TN(c)},CNci:={c∈C:IN(c)≤IN(ci)}$

and

$CNcf:={c∈C:FN(c)≤FN(cf)},$

for all $ct,ci,cf∈C$ . It is obvious that $ct∈CNct,ci∈CNci$ and $cf∈CNcf$ .

Example 3.8. Consider the Sheffer stroke BL-algebra C in Example 3.1. Let ct = a, ci = b, $cf=c∈C$ ,

$TN(x)={ −0.18if x=0, a, f, 1−0.29otherwise, IN(x)={ 0if x=d, e, f−1otherwise and FN(x)={ −0.55if x=0, 1−0.56if x=a, b, c−0.57if x=d, e, f.$

Then

$CNa={x∈C:TN(a)≤TN(x)}={x∈C:−0.18≤TN(x)}={0,a,f,1},CNxb={x∈C:IN(x)≤IN(b)}={x∈C:IN(x)≤−1}={0,a,b,c,1}$

and

$CNc={x∈C:FN(x)≤FN(c)}={x∈C:FN(x)≤−0.56}={a,b,c,d,e,f}.$

Theorem 3.7. Let ct, ci and cf be any elements of a Sheffer stroke BL-algebra C. If CN is a (ultra) neutrosophic $N$ -filter of C, then $CNct,CNci$ and $CNcf$ are (ultra) filters of C.

Proof. Let ct, ci and cf be any elements of C and CN be a neutrosophic $N$ -filter of C. Assume that $c1,c2∈CNct,CNci,CNcf$ . Since $TN(ct)≤TN(c1),TN(ct)≤TN(c2)$ , $IN(c1)≤IN(ci),IN(c2)≤IN(ci)$ and $FN(c1)≤FN(cf),FN(c2)≤FN(cf)$ , we get that

$TN(ct)≤min{TN(c1),TN(c2)}≤TN((c1∣c2)∣(c1∣c2)),IN((c1∣c2)∣(c1∣c2))≤max{IN(c1),IN(c2)}≤IN(ci)$

and

$FN((c1∣c2)∣(c1∣c2))≤max{FN(c1),FN(c2)}≤FN(cf).$

Then $(c1∣c2)∣(c1∣c2)∈CNct,CNci,CNcf$ . Suppose that $c1∈CNct,CNci,CNcf$ and $c1≤c2$ . Since $TN(ct)≤TN(c1)≤TN(c2)$ , $IN(c2)≤IN(c1)≤IN(ci)$ and $FN(c2)≤FN(c1)≤FN(cf)$ , it is obtained that $c2∈CNct,CNci,CNcf$ . Thus, $CNct,CNci$ , $CNcf$ are filters of C.

Let CN be an ultra neutrosophic $N$ -filter of C and $c1∨c2∈CNct,CNci$ , $CNcf$ . Since

$TN(ct)≤TN(c1∨c2)≤TN(c1)∨TN(c2),IN(c1)∨IN(c2)≤IN(c1∨c2)≤IN(ci)$

and

$FN(c1)∨FN(c2)≤FN(c1∨c2)≤FN(cf)$

from Lemma 3.6, it follows that $TN(ct)≤TN(c1),$ $IN(c1)≤IN(ci)$ , $FN(c1)≤FN(cf)$ or $TN(ct)≤TN(c2),$ $IN(c2)≤IN(ci)$ , $FN(c2)≤FN(cf)$ . Hence, $c1∈CNct,CNci,CNcf$ or $c2∈CNct,CNci,CNcf$ . Therefore, $CNct,CNci$ and $CNcf$ are ultra filters of C from Lemma 2.3.

Example 3.9. Consider the Sheffer stroke BL-algebra C in Example 3.1. For a neutrosophic $N$ -filter

$CN={x(−0.21,−0.41,−0.61):x=0,a,b,d}∪{x(−0.13,−0.53,−0.93):x=c,e,f,1}$

of C, ct = b, ci = c and $cf=f∈C$ , the subsets

$CNb={x∈C:TN(b)≤TN(x)}={x∈C:−0.21≤TN(x)}=C,CNc={x∈C:IN(x)≤IN(c)}={x∈C:IN(x)≤−0.53}={c,e,f,1}$

and

$CNf={x∈C:FN(x)≤FN(f)}={x∈C:FN(x)≤−0.93}={c,e,f,1}$

of C are filters of C. Also, $CNb,CNc$ and $CNf$ are ultra since CN is ultra.

The inverse of Theorem 3.7 does not hold in general.

Example 3.10. Consider the Sheffer stroke BL-algebra C in Example 3.1. Then

$CNc={x∈C:TN(c)≤TN(x)}={x∈C:−0.11≤TN(x)}=C,CNd={x∈C:IN(x)≤IN(d)}={x∈C:IN(x)≤0}=C$

and

$CNe={x∈C:FN(x)≤FN(e)}={x∈C:FN(x)≤−0.12}=C$

of C are filters of C but a neutrosophic $N$ -structure

$CN={x(−0.11,0,−0.12):x=0,c,d,e}∪{x(0,−1,−0.87):x=a,b,f,1}$

is not a neutrosophic $N$ -filter of C since TN(d) = −0.11 < 0 = TN(a) when $a≤d$ .

Theorem 3.8. Let ct, ci and cf be any elements of a Sheffer stroke BL-algebra C and CN be a neutrosophic $N$ -structure on C.

1. If $CNct,CNci$ and $CNcf$ are filters of C, then

$TN(c1)≤min{TN(c2∣(c3∣c3)),TN(c2)}⇒TN(c1)≤TN(c3),max{IN(c2∣(c3∣c3)),IN(c2)}≤IN(c1)⇒IN(c3)≤IN(c1) andmax{FN(c2∣(c3∣c3)),FN(c2)}≤FN(c1)⇒FN(c3)≤FN(c1),$

for all $c1,c2,c3∈C$ .

2. If CN satisfies the condition (4) and

$c1≤c2 implies TN(c1)≤TN(c2),IN(c2)≤IN(c1) and FN(c2)≤FN(c1),$

for all $c1,c2,c3∈C$ , then $CNct,CNci$ and $CNcf$ are filters of C, for all $ct∈TN−1, ci∈IN−1$ and $cf∈FN−1$ .

Proof. Let CN be a neutrosophic $N$ -structure on C.

1. Assume that $CNct,CNci$ and $CNcf$ are filters of C, for all $ct,ci,cf∈C$ , and c1, c2 and c3 are any elements of C such that $TN(c1)≤min{TN(c2∣(c3∣c3)),TN(c2)}$ , $max{IN(c2∣(c3∣c3)),IN(c2)}≤IN(c1)$ and $max{FN(c2∣(c3∣c3)),FN(c2)}≤FN(c1)$ . Since $c2∣(c3∣c3),c2∈CNct,CNci,CNcf$ where ct = ci = cf = c1, we have from (SF-4) that $c3∈CNct,CNci,CNcf$ where ct = ci = cf = c1. So, $TN(c1)≤TN(c3)$ , $IN(c3)≤IN(c1)$ and $FN(c3)≤FN(c1)$ , for all $c1,c2,c3∈C$ .

2. Suppose that CN be a neutrosophic $N$ -structure on C satisfying the conditions (4) and (5), for any $ct∈TN−1,ci∈IN−1$ and $cf∈FN−1$ . Let $c1,c2∈CNct,CNci,CNcf$ . Since $c2≤(c2∣c1)∣c1=c1∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2)))$ from Proposition 2.1 (9), (S1)–(S2), and $TN(ct)≤TN(c1),TN(ct)≤TN(c2)$ , $IN(c1)≤IN(ci),IN(c2)≤IN(ci)$ , $FN(c1)≤FN(cf)$ and $FN(c2)≤FN(cf)$ , it follows from the condition (5) that

$TN(ct)≤min{TN(c1),TN(c2)}≤min{TN(c1),TN(c1∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2))))},max{IN(c1),IN(c1∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2))))}≤max{IN(c1),IN(c2)}≤IN(ci)$

and $max{FN(c1),FN(c1∣(((c1∣c2)∣(c1∣c2))∣((c1∣c2)∣(c1∣c2))))}≤max{FN(c1),FN(c2)}≤FN(cf)$ .

Thus, $TN(ct)≤TN((c1∣c2)∣(c1∣c2)),$ $IN((c1∣c2)∣(c1∣c2))≤IN(ci)$ and $FN((c1∣c2)∣(c1∣c2))≤FN(cf)$ from the condition (4), and so, $(c1∣c2)∣(c1∣c2)∈CNct,CNci,CNcf$ . Let $c1≤c2$ and $c1∈CNct,CNci,CNcf$ . Since $TN(ct)≤TN(c1)≤TN(c2),$ $IN(c2)≤IN(c1)≤IN(ci)$ and $FN(c2)≤FN(c1)≤FN(cf)$ from condition (5), it is obtained that $c2∈CNct,CNci,CNcf$ . Thereby, $CNct,CNci$ and $CNcf$ are filters of C.

Example 3.11. Consider the Sheffer stroke BL-algebra C in Example 3.1. Let

$TN(x)={ −0.07if x=1−0.77otherwise, IN(x)={ −0.63if x=e, 10otherwise, and FN(x)={ −0.84if x=a, d, e, 1−0.42otherwise.$

Then the filters $CNct=C,CNci={e.1}$ and $CNcf={a,d,e,1}$ of C satisfy the condition (4), for the elements ct = a, ci = e and cf = d of C.

Also, let

$CN={x(−0.91,−0.23,−0.001):x∈C−{1}}∪{1(−0.17,−0.86,−0.79)}$

be a neutrosophic $N$ -structure on C satisfying the conditions (4) and (5). Then the subsets

$CNct={x∈C:TN(f)≤TN(x)}={x∈C:−0.91≤TN(x)}=C,CNci={x∈C:IN(x)≤IN(b)}={x∈A:IN(x)≤−0.23}=C$

and

$CNcf={x∈C:FN(x)≤FN(1)}={x∈C:FN(x)≤−0.79}={1}$

of C are filters of C, where ct = f, ci = b and cf = 1 of C.

4 Conclusion

In the study, neutrosophic $N$ -structures defined by $N$ -functions on Sheffer stroke BL-algebras have been examined. By giving basic definitions and notions of Sheffer stroke BL-algebras and neutrosophic $N$ -structures on a crispy set X, a neutrosophic $N$ -subalgebra and a $(τ,γ,ρ)$ -level set of a neutrosophic $N$ -structure are defined on Sheffer stroke BL-algebras. We determine a quasi-subalgebra of a Sheffer stroke BL-algebra and prove that the $(τ,γ,ρ)$ -level set of a neutrosophic $N$ -subalgebra of a Sheffer stroke BL-algebra is its quasi-subalgebra and vice versa. Besides, it is stated that the family of all neutrosophic $N$ -subalgebras of the algebra forms a complete distributive lattice. It is illustrated that every neutrosophic $N$ -subalgebra of a Sheffer stroke BL-algebra satisfies $TN(x)≤TN(1)$ , $IN(1)≤IN(x)$ and $FN(1)≤FN(x)$ , for all elements x of the algebra but the inverse does not generally hold. We interpret the case which $N$ -functions defining a neutrosophic $N$ -subalgebra of a Sheffer stroke BL-algebra are constant. Also, a (ultra) neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is described and some properties are analysed. Indeed, it is proved that every neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is the neutrosophic $N$ -subalgebra but the inverse is not true in general, and that the $(τ,γ,ρ)$ -level set of a (ultra) neutrosophic $N$ -filter of a Sheffer stroke BL-algebra is its (ultra) filter and the inverse is always true. After that the subsets $CNct,CNci$ and $CNcf$ of a Sheffer stroke BL-algebra are described by means of $N$ -functions and any elements ct, ci and cf of this algebraic structure, it is demonstrated that these subsets are (ultra) filters of a Sheffer stroke BL-algebra if CN is the (ultra) neutrosophic $N$ -filter.

In future works, we wish to study on plithogenic structures and relationships between neutrosophic $N$ -structures on some algebraic structures.

Acknowledgement: The authors are thankful to the referees for a careful reading of the paper and for valuable comments and suggestions.

Funding Statement: The authors received no specific funding for this study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

References

1. Zadeh, L. A. (1965). Fuzzy sets. Information and Control, 8(3), 338–353. DOI http://dx.doi.org/10.1016/S0019-9958(65)90241-X. [Google Scholar] [CrossRef]

2. Atanassov, K. T. (1986). Intuitionistic fuzzy sets. Fuzzy Sets and Systems, 20(1), 87–96. DOI 10.1016/S0165-0114(86)80034-3. [Google Scholar] [CrossRef]

3. Smarandache, F. (1999). A unifying field in logics. Neutrosophy: Neutrosophic probability, set and logic. Ann Arbor, Michigan, USA: ProQuest Co. [Google Scholar]

4. Smarandache, F. (2005). Neutrosophic set–A generalization of the intuitionistic fuzzy set. International Journal of Pure and Applied Mathematics, 24(3), 287–297. [Google Scholar]

5. Webmaster, University of New Mexico, Gallup Campus, USA, Biography. http://fsunm.edu/FlorentinSmarandache.htm. [Google Scholar]

6. Borumand Saeid, A., Jun, Y. B. (2017). Neutrosophic subalgebras of BCK/BCI-algebras based on neutrosophic points. Annals of Fuzzy Mathematics and Informatics, 14, 87–97. DOI 10.30948/afmi. [Google Scholar] [CrossRef]

7. Muhiuddin, G., Smarandache, F., Jun, Y. B. (2019). Neutrosophi quadruple ideals in neutrosophic quadruple BCI-algebras. Neutrosophic Sets and Systems, 25, 161–173. DOI 10.5281/zenodo.2631518. [Google Scholar] [CrossRef]

8. Jun, Y. B., Lee, K. J., Song, S. Z. (2009). N-ideals of BCK/BCI-algebras. Journal of the Chungcheong Mathematical Society, 22(3), 417–437. [Google Scholar]

9. Muhiuddin, G. (2021). P-ideals of BCI-algebras based on neutrosophic N-structures. Journal of Intelligent & Fuzzy Systems, 40(1), 1097–1105. DOI 10.3233/JIFS-201309. [Google Scholar] [CrossRef]

10. Oner, T., Katican, T., Borumand Saeid, A. (2021). Neutrosophic N-structures on sheffer stroke hilbert algebras. Neutrosophic Sets and Systems (in Press). [Google Scholar]

11. Oner, T., Katican, T., Rezaei, A. (2021). Neutrosophic N-structures on strong Sheffer stroke non-associative MV-algebras. Neutrosophic Sets and Systems, 40, 235–252. DOI 10.5281/zenodo.4549403. [Google Scholar] [CrossRef]

12. Khan, M., Anis, S., Smarandache, F., Jun, Y. B. (2017). Neutrosophic N-structures and their applications in semigroups. Annals of Fuzzy Mathematics and Informatics, 14(6), 583–598. [Google Scholar]

13. Jun, Y. B., Smarandache, F., Bordbar, H. (2017). Neutrosophic N-structures applied to BCK/BCI-algebras. Information–An International Interdisciplinary Journal, 8(128), 1–12. DOI 10.3390/info8040128. [Google Scholar] [CrossRef]

14. Sahin, M., Kargn, A., Çoban, M. A. (2018). Fixed point theorem for neutrosophic triplet partial metric space. Symmetry, 10(7), 240. DOI 10.3390/sym10070240. [Google Scholar] [CrossRef]

15. Rezaei, A., Borumand Saeid, A., Smarandache, F. (2015). Neutrosophic filters in BE-algebras. Ratio Mathematica, 29(1), 65–79. DOI 10.23755/rm.v29i1.22. [Google Scholar] [CrossRef]

16. Sheffer, H. M. (1913). A set of five independent postulates for boolean algebras, with application to logical constants. Transactions of the American Mathematical Society, 14(4), 481–488. DOI 10.1090/S0002-9947-1913-1500960-1. [Google Scholar] [CrossRef]

17. McCune, W., Veroff, R., Fitelson, B., Harris, K., Feist, A. et al. (2002). Short single axioms for boolean algebra. Journal of Automated Reasoning, 29(1), 1–16. DOI 10.1023/A:1020542009983. [Google Scholar] [CrossRef]

18. Chajda, I. (2005). Sheffer operation in ortholattices. Acta Universitatis Palackianae Olomucensis, Facultas Rerum Naturalium. Mathematica, 44(1), 19–23. [Google Scholar]

19. Oner, T., Katican, T., Borumand Saeid, A. (2021). Relation between sheffer stroke and hilbert algebras. Categories and General Algebraic Structures with Applications, 14(1), 245–268. DOI 10.29252/cgasa.14.1.245. [Google Scholar] [CrossRef]

20. Hájek, P. (2013). Metamathematics of fuzzy logic, vol. 4. Berlin, Germany: Springer Science & Business Media. [Google Scholar]

21. Oner, T., Katican, T., Borumand Saeid, A. (2023). (Fuzzy) filters of sheffer stroke bl-algebras. Kragujevac Journal of Mathematics, 47(1), 39–55. [Google Scholar]

This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.