

 | Sound & Vibration |  |
DOI: 10.32604/sv.2022.015882
ARTICLE
On Fractional Integro-Differential Equation with Nonlinear Time Varying Delay
1Department of Mathematics, Faculty of Science, Arish University, AL-Arish, Egypt
2Department of Mathematics, Faculty of Science, Al-Azhar University, Nasr-City, Cairo, Egypt
3Basic Science Department, Higher Technological Institute, 10th of Ramadan City, Egypt
*Corresponding Author: A. M. Abdallah. Email: ahmedabdel3l@yahoo.com
Received: 21 January 2021; Accepted: 09 December 2021
Abstract: In this manuscript, we analyze the solution for class of linear and nonlinear Caputo fractional Volterra Fredholm integro-differential equations with nonlinear time varying delay. Also, we demonstrate the stability analysis for these equations. Our paper provides a convergence of semi-analytical approximate method for these equations. It would be desirable to point out approximate results.
Keywords: Convergence; stability; fractional integro-differential equation
Nowadays, the study of differential equations with nonlinear time varying delay has attracted a wide-ranging interest [1–4]. Meanwhile, few research texts have been based on the theoretical aspects. It is worth to construct an accurate strategy that will facilitate the task of many scholars, followers and experts later. In mathematics, stability of the system is a mathematical property which deals with the behavior of the dynamic systems and corresponds to the convergence of solutions of differential, integro differential and fractional equations [5–12].
It considers as a suitable central role in the study of fractional system [13–15]. This work is perhaps the best straightforward technique to generalize the related papers [16–21].
In [21], Soliman et al. carried out a detailed and comprehensive analysis of fractional Volterra Fred-holm integro-differential equation with constant delay. Likewise, our present study motivated, constructed, continuously developed thus far parallels and properly cited (inspired) by the results of the above work [22–26]. It also includes analysis extensively, but differs significantly about the aforementioned work. It is an attempt to generalize different precedent works and in order to achieve a notable contribution with its counterparts. For more details, this context relied on the appropriate analysis of some linear and nonlinear problems described by fractional integro-differential equations with variable delays. More precisely, we receive a noticeable attention to the nonlinear integro-differential equations with nonlinear time varying delay as in [17]. So far, the strategy of the current research contains four beneficial items. At the first, existence and uniqueness of the solution of the proposed problem will be examined. Secondly, we will provide the readers with the stability and the convergence of this solution. At the end, we shall suggest a method and its convergence therein. This literature consists of five sections. Some basic definitions and theorems are introduced in the first section. Indeed, the suggested problem is discussed and analytical explanation of the proposed problem is detailed in section two. Some Important properties of the solution is mentioned in section three. A modified method is explained in section four. Finally, An experimental example is illustrated in section five.
1.1 Mathematical Tools and Theoretical Background
The most commonly notations, definitions and theorems are mentioned. The presented preliminaries are related to our paper.
Definition 1.1. The Banach space U = C([a, b], ℝ4) is the space of all real-valued continuous functions from :[a, b] → ℝ4, let U(t) = {u(t):u(t) ∈ C([a, b], ℝ4 and Dsu ∈ C([a, b], ℝ4, s ∈ (0, 1]} endowed with the norm ‖ . ‖; ‖ u ‖ = max{|u(t)| + |Dsu(t)|:t ∈ [a, b]}, Dsu denotes the Caputo derivative of fractional order s [27,28].
Definition 1.2. Let σ:U → U be a mapping on a Banach space (U, ‖ . ‖). The point u ∈ U is called a fixed point of σ with σu = u.
Definition 1.3. The mapping σ on a Banach space (U, ‖ . ‖) is called contractive if there exists C ∈ (0, 1), such that
Definition 1.4. For w ∈ C([a, b], ℝ4), the q−th Caputo fractional derivative of a function is defined by
where n = [q] + 1, m ∈ ℕ, [q] is Euler Gamma function for q and [q] denotes the integer part of the real number q.
Definition 1.5. The Mittag-Leffler type is defined as
at b = 1, a > 0 the previous equation becomes the classical Mittag-Leffler.
Definition 1.6. [27] The fractional equation
is Ulam-Hyers stable if there exists Cf such that for each ε > 0 and v ∈ C([a, b], ℝ4)
t is the independent variable.
Definition 1.7. [27] The fractional integro-equation
is Ulam-Hyers stable Rassias stable with respect to ν if there exists a positive constant
L > 0 with the following property: For each u(t) satisfying
then
Definition 1.8. The sequence
ε, N > 0 such that
Lemma 1.9. [26] (Gronwall’lemma)
Let u and v be nonnegative continuous functions on some interval t ∈ [a, b]. Also, let the function f(t) be positive, continuous and monotonically non-decreasing on [a, b] and u satisfies the inequality
Then, there holds the inequality
Lemma 1.10. [26] (Pachpatte’inequality) Let u, v and w be nonnegative continuous functions on ℝ+ and f(t) be a positive and non-decreasing continuous function, the inequality
holds, then
Theorem 1.11. [26] (Banach contraction mapping theorem)
Let (U, ‖ . ‖) be a Banach space, σ:U → U is an operator. If σ is contraction (contractive) mapping. Then σ has exactly one fixed point.
Theorem 1.12. [27] (Schauder fixed point theorem)
Let (U, ‖ . ‖) be a closed, convex and nonempty subset of a Banach space C[a, b], suppose that σ:U → U is a continuous mapping such that σ(U) is a relatively compact subset of C[a, b]. Then σ has at least one fixed point in U.
2 Analytic Explanation of the Problem
Throughout this paper, we will consider Caputo fractional integro-differential equation of the form:
through the initial condition:
where
0 < α < 1, 0 < τ < b, f:[a, b] × U × U × U → U is a continuous function, G, H:[a, b]2 × U → U → U are nonlinear Lipschitz continuous functions of u(t) and χ:U → ℝ4 is a continuous function.
Let us assume the following conditions:
(1) There exists a constant Cf > 0, for each u1, u2, v1, v2, w1, w2 ∈ U
(2) There exists a constant CG > 0
(3) There exists a constant CH > 0
(4) There exists a constant
(5) There exists a constant
Theorem 2.1. The Eq. (14) is equivalent to
Proof. Integrating two both sides of Eq. (14), we get
This leads to
Operate with
Then,
Differentiating we obtain
where
Let σ:U → U, for any u ∈ U. Now, we establish the following theorem for the fixed point σ.
Theorem 2.2. The operator σ maps U into itself and it is also continuous on [a, b].
Proof.
That is, σ maps U into itself. Also, A becomes uniformly bounded. Suppose a sufficiently small number n > 0,
In short,
Consequently, we thus conclude that
where t ∈ [a, b],
Then, σu(t) is continuous on [a, b]. Our approach for proving that σ is continuous, we suppose that un converge to u,
If we follow the conditions Eqs. (16)–(20), we reach
This is equivalent to
This shows that σun → σu.
2.1 Existence and uniqueness of the solution for Eq. (14)
In what follows, we will investigate the existence and uniqueness of solution for the fractional integro-differential equation with time-varying delay (variable delay).
Theorem 2.3. Suppose that the conditions Eqs. (16)–(20) hold, then the non-linear fractional integro-differential Eq. (14) has at least a unique solution u ∈ U.
Proof. By analogous proof to the continuity of σ operator.
For brevity,
In consequent, we have ‖ σu(t) − σv(t)‖ ≤ Y‖u(s) − v(t)‖, where t ∈ [a,b],
We conclude that σ is Lipschitz on U with Lipschitz constant Y. It is well known that σ is a fixed point as a consequence of Theorem 1.11., i.e., σ is a contractive mapping. Eq. (14) has immediately at least a unique solution u ∈ U.
Lemma 2.1. Assume that {u(t)} is a continuous function on [a, b], it satisfies
Further, ‖u(t1) − u(t2)‖ ≤ q. Then {u(t)} is equicontinuous on [a, b].
Proof. Without loss of generality, for t1, t2 ∈ [a, b] such that t1 < t2, we get
whenever t1 → t2, q > 0, where
Lemma 2.2. If the conditions Eqs. (16)–(20) satisfied, then the non-linear Eq. (14) has a unique solution provided
Our following attention is focused on checking the stability of the solution u(t) for Eq. (14) in the frame of Ulam-Hyers and Ulam-Hyers-Rassias.
2.2 Stability Analysis of the Solution for Eq. (14)
Theorem 2.4. Assume that the conditions Eqs. (16)–(20) hold. Then the non-linear fractional integro-differential Eq. (14) is Ulam-Hyers stable.
Proof. Let u ∈ U be a solution of Eq. (14), D(s) is a continuous and non negative function such that
Now, we are going to apply the integral operator
Equivalently
for v(t) ∈ U, it can be written as
The difference |u(t) − v(t)| is given as
or equivalent to
From Gronwall’s lemma Eqs. (10), (11) yields
where K > 0, R = Cf(CG + CH) (CG + CH) such that
In consequence, the problem (14) is stable in the sense of Ulam-Hyers. This completes the proof.
Theorem 2.5. Suppose that the conditions Eqs. (16)–(20) satisfied, P(t) ∈ U is an increasing function and
Proof. Let w ∈ U be a solution of the following inequality
Further, for any t ∈ [a, b], ε > 0. Assume that u ∈ U is the solution of (14). Now, integrate (14), that is
It can be easily noticed that
Hence,
It directly follows from Pachpatte’s lemma Eqs. (12)–(13) that
for C > 0 which ends the proof.
Let us extend our results to asymptotically stable solution. For that, we shall perform the absolute value for the solution of (14)
In fact, by means of Cauchy Schwartz inequality, we deduce
where
Now, we observe that |u(t)| → 0 whenever t → ∞. Therefore, the zero solution of (14) is said to be asymptotically stable.
3 Some Important Properties of the Solution
There is no doubt that there are various properties that characterize solutions. So, we will look at two features, namely continuous dependence of solution and estimates on the solution.
Theorem 3.1. For the two solutions u1(t), u2(t) of Eq. (14).
Proof. See proof of Theorem 4.1.
Theorem 3.2. Assume that the function f in Eq. (14) is Lipschitz function. If u(t) is a solution of Eq. (14), then
Proof. Also, the proof is similar to the proof of Theorem 4.1.
4 Modified Variational Iteration Method with Adomain Decomposition Method
The ongoing method is distinct and the results improve quickly. We will create the correct functional in the following form:
where λ is a Lagrange multiplier. The solution is defined by the infinite series
The nonlinear function can be written as [16,24]
Since An, Bn and Cn are the Adomain polynomials of u0, u1, …, un. Substitute (37)–(40) in (36), we have
For λ = −1, with another formula
5 Experimental and Numerical Examples
Here, we give examples (application situations for the applied fractional equation) which clarifying the gained results.
In this subsection, we shall present the numerical results gained by employing iterative methods namely modified variational iteration method with Adomain decomposition method
Example 1
Solution
For n = 0
By Adomain decomposition method, α = 0.5, we get A0 = B0 = C0 = 0. Hence u1 = t2 is also the exact (analytical) solution. Exactly, u0 = u2 = … = 0.
Example 2
Solution
By the same procedures of the previous example, we have u1 = t.
5.2 Graphical Representation of Solution for Eq. (43)
Firstly, let α approach to 0.5 and n = 1, the obtained solution for this case represent graphically in Fig. 1.
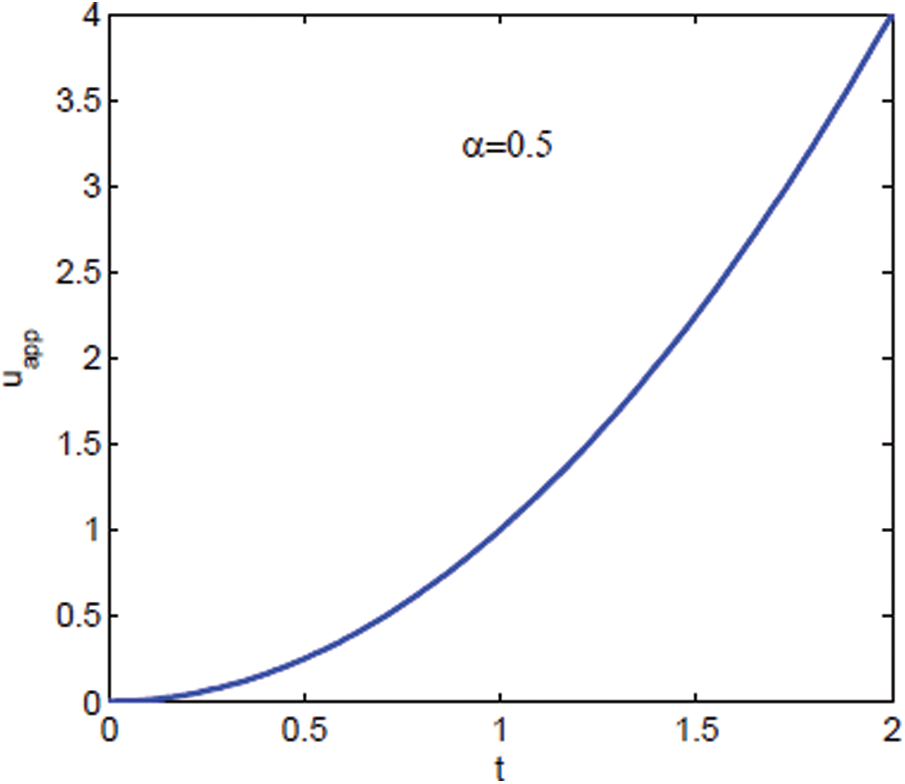
Figure 1: Approximate solution at α = 0.5
Finally, let α approach to 1 and n = 1, 2, 3. Approximate solutions for this case is obtained in Fig. 2.
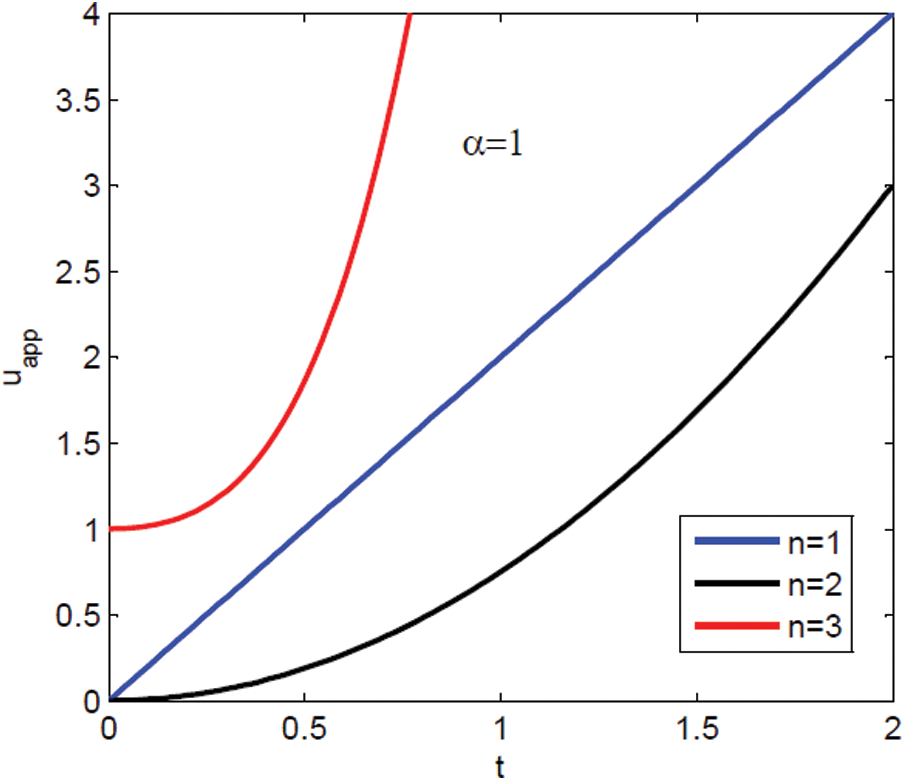
Figure 2: Approximate solutions at α = 1
Table 1 shows the analysis results for Eq. (43).
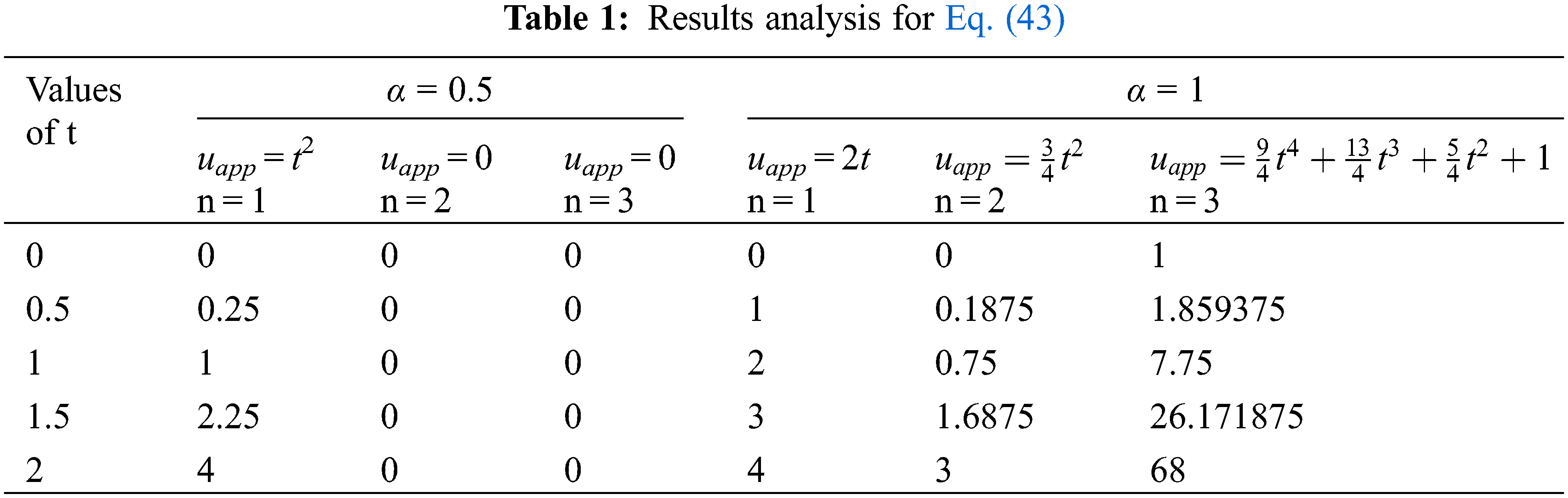
Figs. 1–2 indicate the difference to uapp at different values of n.
Fig. 1 is the relationship between t and the approximate solution uapp at α = 0.5, n = 1 only, otherwise, at other values of n we find that the approximate solution approaches zero.
Fig. 2 is the relationship between t and the approximate solution uapp at α = 1, n = 1, 2, 3.
We emphasize that the analysis of fractional integro-differential equations with delay attracts considerable attention by many scientists [29,30]. The beneficial contribution of this work was the discovery much of the tools in the analysis process for many equations. It should be noted that the choice of α plays a vital role in the results of the suggested problem and this was evident in the examples that were listed in our research. We find that when the approximate solution and the exact solution apply when a value of α is at a certain value of n, and other than this value of n, the approximate solution is 0.
Acknowledgement: The authors are very grateful to the editors and reviewers for carefully reading the paper and for their comments and suggestions which have improved the paper.
Funding Statement: The authors received no specific funding for this study.
Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.
1. Luo, D., Luo, Z. (2019). Existence and finite-time stability of solutions for a class of to nonlinear fractional differential equations with time-varying delays and non-instantaneous impulses. Advances in Difference Equations, 155, 1–21. DOI 10.1186/s13662-019-2101-9. [Google Scholar] [CrossRef]
2. Paulo, J., Mallika, M., Cuevas, C. (2011). Existence results of fractional neutral integrodifferential equations with state-dependent delay. Computers & Mathematics with Applications, 62, 1275–1283. DOI 10.1016/j.camwa.2011.03.048. [Google Scholar] [CrossRef]
3. Tun, C. (2016). New stability and boundedness results to volterra integro-differential equations with delay. Journal of the Egyptian Mathematical Society, 24(2), 210–213. DOI 10.1016/j.joems.2015.08.001. [Google Scholar] [CrossRef]
4. Zhao, J., Meng, F. (2018). Stability analysis of solutions for a kind of integro-differential equations with a delay. Journal of Mathematical Problems in Engineering, 2018(12), 1–7. DOI 10.1155/2018/9519020. [Google Scholar] [CrossRef]
5. Burton, T. A. (1979). Stability theory for volterra equations. Journal of Differential Equations, 32(1), 101–118. DOI 10.1016/0022-0396(79)90054-8. [Google Scholar] [CrossRef]
6. Chalishajar, D., Ravichandran, C., Dhanalakshmi, S., Murugesu, R. (2019). Existence of fractional impulsive functional integro-differential equations in banach spaces. Journal of Applied System Innovation, 2(18), 1–17. DOI 10.3390/asi2020018. [Google Scholar] [CrossRef]
7. Hilfer, R. (2000). Application of fractional calculus in physics. Singapore: World Scientific. [Google Scholar]
8. Kaslik, E., Sivasundaram, S. (2012). Analytical and numerical methods for the stability analysis of linear fractional delay differential equations. Journal of Computational and Applied Mathematics, 236(16), 4027–4041. DOI 10.1016/j.cam.2012.03.010. [Google Scholar] [CrossRef]
9. Khalil, R., Al Horani, M. (2014). A new definition of fractional derivative. Journal of Computational and Applied Mathematics, 264, 65–70. DOI 10.1016/j.cam.2014.01.002. [Google Scholar] [CrossRef]
10. Kumar, P., Haloi, R., Bahuguna, D., Pandey, D. N. (2016). Existence of solutions to a New class of abstract non-instantaneous impulsive fractional integro-differential equations. Journal of Nonlinear Dynamics and Systems Theory, 16(1), 73–85. [Google Scholar]
11. Lazarevic, M. P., Spasic, A. M. (2009). Finite-time stability analysis of fractional order time delay systems. Gronwall’s Approach. Journal of Mathematical and Computer Modelling, 49(3–4), 475481. DOI 10.1016/j.mcm.2008.09.011. [Google Scholar] [CrossRef]
12. Liua, K., Jiang, W. (2016). Stability of nonlinear caputo fractional differential equations. Journal of Applied Mathematical Modelling, 40(5–6), 3919–3924. DOI 10.1016/j.apm.2015.10.048. [Google Scholar] [CrossRef]
13. Agarwal, R. P., Andrade, B. D., Siracusa, G. (2011). On fractional integro-differential equations with state-dependent delay. Computers & Mathematics with Applications, 62(3), 1143–1149. DOI 10.1016/j.camwa.2011.02.033. [Google Scholar] [CrossRef]
14. Behiry, S. H., Abd-Elmonem, R. A., Gomaa, A. M. (2010). Discrete adomian decomposition solution of nonlinear fredholm integral equation. Journal of Ain Shams Engineering, 2010(1), 97–101. DOI 10.1016/j.asej.2010.09.009. [Google Scholar] [CrossRef]
15. Matignon, D. (1996). Stability results on fractional differential equations to control processing. Computational Engineering in Systems and Application, 2, 963–968. [Google Scholar]
16. Manafianheris, J. (2012). Solving the integro-differential equations using the modified laplace adomain decomposition method. Journal of Mathematical Extension, 6(1), 41–55. [Google Scholar]
17. Morgado, M. L., Ford, N. J., Lima, P. M. (2013). Analysis and numerical methods for fractional differential equations with delay. Journal of Computational and Applied Mathematics, 252, 159–168. DOI 10.1016/j.cam.2012.06.034. [Google Scholar] [CrossRef]
18. Zada, A., Waheed, H., Alzabut, J., Wang, X. (2019). Existence and stability of impulsive coupled system of fractional integrodifferential equations. Demonstratio Mathematica, 52(1), 296–335. DOI 10.1515/dema-2019-0035. [Google Scholar] [CrossRef]
19. Zaghrout, A. S. S. (1993). On volterra-fredholm integral equations. Periodic Methmatica Hungarica, 26(1), 55–64. DOI 10.1007/bf01875881. [Google Scholar] [CrossRef]
20. Zhang, P., Hao, X., Liu, L. (2019). Existence and uniqueness of the global solution for a class of nonlinear fractional integro-differential equations in a banach space. Advances in Differential Equations, 135, 1–10. DOI 10.1186/s13662-019-2076-6. [Google Scholar] [CrossRef]
21. Soliman, A. A., Raslan, K. R., Abdallah, A. M. (2021). Analysis for fractional integro-differential equation with time delay. Italian Journal of Pure and Applied Mathematics, 46, 989–1007. [Google Scholar]
22. Muslim, M., Kumar, A., Feckan, M. (2018). Existence, uniqueness and stability of solutions to second order nonlinear differential equations with non-instantaneous impulses. Journal of King Saud University-Science, 30(2), 204–213. DOI 10.1016/j.jksus.2016.11.005. [Google Scholar] [CrossRef]
23. Oldham, K. B. (2010). Fractional differential equations in electrochemistry. Advances in Engineering Software, 41(1), 9–12. DOI 10.1016/j.advengsoft.2008.12.012. [Google Scholar] [CrossRef]
24. Vanualailai, J., Nakagiri, S. (2003). Stability of a system of volterra integro- differential equations. Journal of Mathematical Analysis and Applications, 281(2), 602–619. DOI 10.1016/S0022-247X(03)00171-9. [Google Scholar] [CrossRef]
25. Weia, W., Lia, X., Li, X. (2012). New stability results for fractional integral equation. Computers & Mathematics with Applications, 64(10), 3468–3476. DOI 10.1016/j.camwa.2012.02.057. [Google Scholar] [CrossRef]
26. Magin, R. L. (2006). Fractional calculus in bioengineering. Begell House Publishers, Redding. [Google Scholar]
27. Podlubny, I. (1999). Fractional differential equations. Boston: Academic Press. [Google Scholar]
28. Nouri, K., Nazari, M., Keramati, B. (2017). Existence results of solutions for some fractional neutral functional integro-differential equations with infinite delay. Preprints, 1–11. DOI 10.20944/preprints201706.0090.v1. [Google Scholar] [CrossRef]
29. Paulo, J., Mallika, M., Cuevas, C. (2011). Existence results of fractional neutral integrodifferential equations with state-dependent delay. Computers & Mathematics with Applications, 62, 1275–1283. DOI 10.1016/j.camwa.2011.03.048. [Google Scholar] [CrossRef]
30. Sadati, S. J., Ghaderi, R., Ranjbar, A. N. (2013). Some fractional comparison results and stability theorem for fractional time delay systems. Romanian Reports in Physics, 65(1), 94–102. [Google Scholar]
 | This work is licensed under a Creative Commons Attribution 4.0 International License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. |