New Class of Doubt Bipolar Fuzzy Sub Measure Algebra

1  Introduction

The BCK-algebras class has been identified as an appropriate subclass of the BCI-algebra class. Iséki et al. [1–4] proposed BCK/BCI-algebras as two key classes of Boolean algebra. BCK/BCI-algebra theory has spawned a plethora of literature since then. This gave a useful mathematical tool for modeling systems that were overly complicated or inadequately specified. Bipolar sets have been used in a variety of fields of mathematics since then. What is now known as fuzzy mathematics is the research of fuzzy sets and various implications in mathematics. Zhang et al. [5] was the first to propose bipolar fuzzy groups as a generalization of regular fuzzy groups [0, 1] in 1994. In addition, Lee [6] proposed a bipolar fuzzy group extension for fuzzy groups. Fuzzy dipole groups confer both a positive and negative membership score, while fuzzy groups confer a degree of membership in an element in a particular group. The interval [0, 1] corresponds to positive membership degrees, while the interval [−1, 0] corresponds to negative membership degrees. The range of degrees of membership in bipolar fuzzy groups is raised from period [0, 1] to period [−1, 1]. Many operations and relations have been proposed to dipole fuzzy sets as a basis for investigating the enigmatic pole set theory [−1, 1]. Fuzzy dipole group theory has recently gained traction in a variety of fields, including group theory, half group theory, semifinal theorems, statistics, medicine, and among others. Rosenfeld [7] introduced a fuzzy subgroup of fuzzy algebraic structures.

There have been many contributions to the concepts of fuzzy subgroups and fuzzy ideal of doubt BCK/BCI-algebras by several researchers such as: Biswas [8] later proposed the concept of non-fuzzy subgroups of groups. The related characteristics of BCK-algebras using the fuzzy group notion are examined [9]. Jun et al. have also researched fuzzy features of numerous ideas in BCK/BCI/RHO-algebras [10–21]. Huang [22], on the other hand, are concerned with BCI algebra in other ways. To avoid confusion resulting from, Huang’s definition of perturbation BCI-algebra [23], Jun [24] proposed the definition of the fuzzy sub-algebra of doubt and the fuzzy ideal of doubt BCK/BCI-algebras and offered some conclusions concerning them. Following that, Zhan et al. [25] added the concept of ambiguous skepticism to BCI-algebra ideals, as well as the concept of doubt opaque H ideals in BCK algebra. As a result of what was accomplished by the previous works, new concepts were proposed and circulated to fuzzy measure algebra. The objective of this work is to introduce the concept of BCW and BCM measure algebras in a bipolar fuzzy measure array with discussed properties in this study. Also, we introduce fuzzy bipolar measure algebra and bipolar fuzzy measure proverb, as well as examine associated aspects. Then, by doubting the set of positive segments at the t-level and doubting the set of negative s-level segments, we describe the doubtful fuzzy dipole measure sub-algebra and the questionable fuzzy dipole measure ideal. Also examined are the connections between the ambiguous dipole measure sub-algebra and the ambiguous dipole perfect measure sub-algebra. Finally, we study homogenous and doubt images of putative fuzzy bipolar measure ideals in BCW and BCM-measure algebras. Moreover, we define the conditions under which a perfect fuzzy dipole measure model becomes a fuzzy closed dipole model.

2  Preliminaries

Definition 2.1. [26] A functional μ:T→R+ is called a σ-additive measure if whenever a set A∈T is a disjoint union of an at most countable sequence {Ak}k=1M (where M is either finite or M=∞) then u(A)=∑k=1Mu(Ak). If M=∞ then the above sum is understood as a string. If this property applies only to the finite values of M, then μ is a final additive measure.

Definition 2.2. [27] Let W be a universe of discourse, then a fuzzy set (FS) T is characterized by a membership function μT(w) that takes values in [0, 1].

Definition 2.3. [28]. Let ∅≠J⊆F, where F is BCK/BCI algebra. Then J is a sub algebra of F if ∀ζ,η∈J then ζ∗η∈J.

Definition 2.4. [28]. Let ∅≠J⊆F, where F is BCK/BCI algebra. Then J is an ideal of F if it achieves the following:

1)   0∈J

2)   ∀ζ,η∈F,ζ∗η∈J,η∈J⇒ζ∈J.

Definition 2.5. [29]. Let F≠∅. An M-polar fuzzy measure set is a map ψ:F→[0,1]M. The membership value of anyζinF is defined by:

ψ(ζ)=(P1∘ψ(ζ),P2∘ψ(ζ),…,PM∘ψ(ζ)) where Pk∘ψ(ζ):[0,1]M→[0,1] is defined as the k-th function of projection.

Definition 2.6. [30] A fuzzy ordered set (X,μR) is called a fuzzy well-ordered set if it is a totally fuzzy ordered set in which every non-empty subset has a fuzzy least element.

Definition 2.7. [30] (Zorn’s lemma) Let P be a partially ordered set. If every chain in P has an upper bound, then X has a maximal element.

Proposition 2.8. [30] If Zorn’s lemma holds, then fuzzy Zorn’s lemma holds.

3  BCW and BCM in Polar Fuzzy Measure Sub-Algebras

In this part, we give three concepts of BCWandBCM in fuzzy measure algebra and with a study of its most prominent characteristics.

Definition 3.1. Let ∅≠J⊆F where F is fuzzy measure algebra. Then J is a BCW− sub algebra of F if (ζ∗η)−1∈J,∀ζ,η∈J.

Example 3-1: A 3-polar fuzzy measure set ψ:F={0,1,j,2}→[0,1]3 by:

ψ(x)={(0.6,0.7,0.4),ifx=0(0.3,0.5,0.6),ifx=1(0.1,0.4,0.5),ifx=2(0.0,0.3,0.7),ifx=j

So ψ is a 3-polar fuzzy measure ideal of F.

For each 3-polar fuzzy measure set ψ on F with σˇ={σ1,σ2,σ3}∈[0,1]3, the set ψ[σˇ]={x∈F:ψ(x)≥σˇ} is BCM-sub algebra set of F because (J∗η)2∈ψ[σˇ], ∀J,η∈ψ[σˇ] for the ∗-operation we can see the following Cayley table:

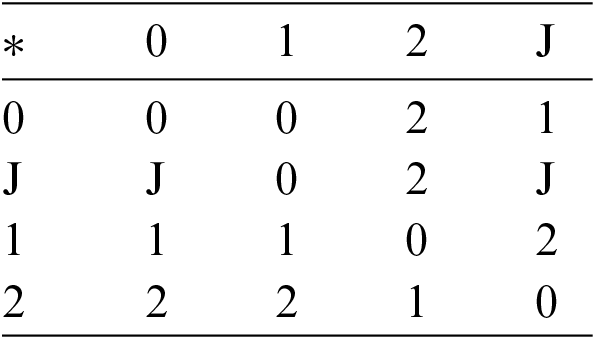
Let K={0, 1}, then K is BCM-sub algebra of F.

Definition 3.2. Let ∅≠J⊆F, where F is fuzzy measure algebra. Then J is a BCM− sub algebra of F if (ζ∗η)2∈J∀ζ,η∈J.

Definition 3.3. Let ∅≠J⊆F, where F is BCWandBCM fuzzy measure algebra. Then J is an ideal of F if it achieves the following:

1)   0,1∈F.

2)   ∀ζ,η∈F,(ζ∗η)2∈J,η∈J⇒ζ2∈J.

Definition 3.4. A fuzzy measure effect algebra is a system (F,M,0F,u,⊕) consisting of a set F,M is fuzzy measure on bolean algebra, special elements 0F and u are called the zero and the unit respectively, and a totally defined binary operation ⊕ on F, called the ortho sum if for all h,ℓ,ℷ∈F:

1)   Ifh⊕ℓ and (h⊕ℓ)2⊕ℷ are defined, then ℓ⊕ℷ and h⊕(ℓ⊕ℷ) are defined and h⊕(ℓ⊕ℷ)2=(h⊕ℓ)2⊕ℷ.

2)   Ifh⊕ℓ2 is defined, then (ℓ⊕ℷ)2=(ℓ⊕h)2, also ℓ2⊕h is fuzzy measure set.

3)   ∀h∈F, there is a unique ℓ∈F such that h⊕ℓ2 is fuzzy measure set and h⊕ℓ2=u.

4)   Ifh⊕u is fuzzy measure set defined, then h=0F.

Definition 3.5. Let Θˇ be M-polar fuzzy measure set of F. We say Θˇ is an M-polar fuzzy measure sub-algebra if: ∀μ,ν∈F,(Θˇ(μ∗ν))≥inf{Θˇ(μ),Θˇ(ν)}, where Θˇ(μ),Θˇ(ν) are fuzzy measure point of μ and ν, respectively. So ∀μ,ν∈F

pi∘Θˇ(μ∗ν)≥inf{pi∘Θˇ(μ),pi∘Θˇ(ν)}∀i=1,2,…,m.

Lemma 3.6. If Θˇ is a polar fuzzy measure sub-algebra of F, then Θˇ(0)≥Θˇ(μ),∀μ∈F.

Proof. Let Θˇ be a polar fuzzy measure sub-algebra of F. Then, we have Θˇ(μ∗ν)≥inf{Θˇ(μ),Θˇ(ν)},∀μ,ν∈F.Takeμ=ν, we have Θˇ(0)=Θˇ(μ∗μ)≥inf{Θˇ(μ),Θˇ(μ)}=Θˇ(μ)→Θˇ(0)≥Θˇ(μ),∀μ∈F.

Example 3.7. Let F={0,ι2,κ2} be BCW and BCM- fuzzy measure algebra. Define a mapping Θˇ:F→[0,1]3 by:

Θˇ(μ)={(0.4,0.4,0.8)ifμ=0.(0.1,0.2,0.4)ifμ=ι2.(0.2,0.3,0.3)ifμ=κ2.

Then Θˇ is 3-polar fuzzy measure sub-algebra of F.

Definition 3.8. A polar fuzzy measure set Θˇ of F is said to be an a polar fuzzy measure ideal (PFMI, for short) if satisfies:

∀μ,ν∈F,(pi∘Θ⌣(0)≥pi∘Θ⌣(μ)2≤sup{pi∘Θ⌣(μ∗ν)2,pi∘Θ⌣(μ)2})∀i=1,2,…,ζ.

Theorem 3.9. Suppose that Θˇ is an M-polar fuzzy measure set of F. Then Θˇ[σˇ] is BCM-sub algebra of F for any Θˇ[σˇ]≠∅, where σˇ={σ1,σ2,…,σm}∈[0,1]m if and only if Θˇ is an M-polar fuzzy measure sub-algebra of F.

Proof. Suppose that Θˇ is an polar fuzzy measure sub-algebra of F and assume σˇ∈[0,1]m with Θˇ[σˇ]≠∅. Let μ,ν∈Θˇ[σˇ]. Then Θˇ(μ)≥σ~ and Θˇ(ν)≥σ~. It follows that Θˇ(μ∗ν)≥inf{Θˇ(μ),Θˇ(ν)}≥σ~ and hence Θ⌣(((μ∗ν)2))=Θ⌣((μ∗ν)∗(μ∗ν))≥inf{Θ⌣((μ∗ν)),Θ⌣((μ∗ν))}≥σ~, thus (μ∗ν)2∈Θˇ[σˇ]. Therefore Θˇ[σˇ] is BCM-sub algebra of F.

Conversely, assume that Θˇ[σˇ] is BCM-sub algebra of F, i.e., Θˇ(μ∗ν)≥inf{Θˇ(μ),Θˇ(ν)},∀μ,ν∈F. Now, suppose that there exist μ,ν∈F s.t, Θˇ(μ∗ν)<inf{Θˇ(μ),Θˇ(ν)}. Thus there exist σˇ={σ1,σ2,…,σm}∈[0,1]m such that, Θˇ(μ∗ν)<σ~≤inf{Θˇ(μ),Θˇ(ν)}.However,inf{Θˇ(μ),Θˇ(ν)}≤Θˇ(μ)andinf{Θˇ(μ),Θˇ(ν)}≤Θˇ(ν). Hence μ,ν∈Θˇ[σˇ], but (μ∗ν)∉Θˇ[σˇ] and it is a contradiction. So Θˇ(μ∗ν)≥inf{Θˇ(μ),Θˇ(ν)},∀μ,ν∈F. Thus Θˇ is an polar fuzzy measure sub- algebra of F.

Proposition 3.10. A polar fuzzy measure sub-algebra Θˇ of F is a PFMI if such that Θˇ(μ∗ν)2≤Θˇ(ν)2 implies Θˇ(μ)=Θˇ(0),∀μ,ν∈F.

Example 3.11. Let F={0,ι2,3,6} be BCW and BCM-fuzzy measure algebra with Cayley table defined by a mapping Θˇ:F→[0,1]3:

Θˇ(μ)={(0.7,0.3,0.6)ifμ=0,3.(0.4,0.6,0.8)ifμ=ι2.(0.3,0.3,0.7)ifμ=6.

Then Θˇ is a (PFMI) of F.

Proposition 3.12. If Θˇ is an PFMI of F, then

∀μ,ν∈F,μ≥ν⇒Θˇ(μ)2≤Θˇ(ν)2.

Proof. Let μ,ν∈F be s.t, μ≥ν. Then μ∗ν=1 and so

Θˇ(μ)2≤sup{Θˇ(μ∗ν)2,Θˇ(ν)2}=sup{Θˇ(1),Θˇ(ν)2}=Θˇ(ν)2. Thus Θˇ(μ)2≤Θˇ(ν)2.

Theorem 3.13. Let ω∈F. If Θˇ is a PFMI of F, then Fω is an fuzzy measure ideal of F.

Proof. Let ω∈F. Let μ,ν∈F be s.t, (μ∗ν)2∈Fω and ν∈Fω. Then Θˇ(μ∗ν)2≤Θˇ(ω)2 and Θˇ(μ)2≤Θˇ(ω)2. Since Θˇ is an M-polar fuzzy measure ideal (M-PFMI, for short) of F, so Θˇ(μ)2≤sup{Θˇ(μ∗ν)2,Θˇ(ν)2}≥Θˇ(ω)2, ω2∈Fω. Hence, Fω is an fuzzy measure ideal of F.

Defi1q`nition 3.14. Let F be a BCW and BCM fuzzy measure algebra. Then a PFMI Θˇ of F is closed if it is a polar fuzzy measure sub-algebra of F.

Proposition 3.15. Every closed a PFMI Θˇ of a BCW-fuzzy measure algebra F satisfies:

∀μ∈F,Θˇ(1∗μ)2≤Θˇ(μ)2.

Proof. For any μ∈F, we have Θˇ(1∗μ)2≤sup{Θˇ(1),Θˇ(μ)2} ≤sup{Θˇ(1),Θˇ(μ)2}=Θˇ(μ). Thus, we get the result.

Proposition 3.16. Every closed M-PFMI Θˇ of a BCM-fuzzy measure algebra F such that:

∀μ∈FΘˇ(1∗μ)2≤Θˇ(μ)2.

Proof. For any μ∈F, we have Θˇ(1∗μ)2≥sup{Θˇ(0),Θˇ(μ)} ≥sup{Θˇ(x),Θˇ(μ)2}=Θˇ(μ)2. Therefore Θˇ(1∗μ)2≥Θˇ(μ)2.

4  M-Polar (α, β)-Fuzzy Measure Ideals

In this part, we suggest and discussion this concept M-polar (α,β)-BCM and BCI2 fuzzy measure ideals, where: α,β∈{∈,δ,∈∨δ,∈∧δ},α≠∈∧q.

Proposition 4-1. Let ℘ be an M-polar fuzzy measure M-PFM of F, the set ℘1≠∅,∀ι^∈(0.25,1]m is an ideal of F if and only if ℘ such that the assertions below: for all x,y∈F,

(1)   Inf{℘(0),0.2⌣5}≥℘(x).

(2)   Inf{℘(x),0.2⌣5}≥inf{℘(x∗y),℘(y)}.

Proof. Suppose that ℘1≠∅ is an ideal of F with sup{℘(0),0.2⌣5}<℘(ν), for some υ∈F. Then, ℘(ν)∈(0.25,1]m, so υ∈℘℘(ν), hence ℘(0)>℘(ν), thus 0∉℘℘(ν) and its a contradiction. So that (1) holds.

Now, suppose sup{℘(x),0.2⌣5}>inf{℘(x∗y),℘(y)}=ι^ for some x,y∈F. So, ι^∈(0.25,1]mm and y,x∗y∈℘1. Let, x∉℘1 since ℘(x)>ι^, a contradiction. Hence, (2) holds.

Conversely, assume that (1) and (2) hold. Let ι^∈(0.25,1]m be such that ℘1≠∅, for any x∈℘, then 0.2⌣5>ι^≤℘(x)≥sup{℘(x),0.2⌣5} So that, ℘(0)=sup{℘(x),0.2⌣5}≤ι^. Thus, 0∈℘ι^. Let x,y∈F be such that x∗y,y∈℘ι^ .

Therefore sup{℘(x),0.2⌣5}≤inf{℘(x∗y),℘(y)}≤ι^<0.2⌣5, hence, ℘(x)=sup{℘(x),0.2⌣5}≤ι^, that is, x∈℘ι^. Thus ℘ι^ is an ideal of F.

Definition 4.2. Let ℘ be an M-PFMI of F. We say ℘ is an M-polar (α,β)−BCK2− fuzzy measure ideal [M–P-(α,β)-BCK2− FMI, for short] of F if for all x,y∈F and ι^,κ^∈(0.5,1]m,

(1) If xι^α℘ then 0.5ι^β℘.

(2) If (x∗y)2ι^α℘ and y\, κ^α℘ then xsup{ι^,κ^}β℘.

Definition 4.3. Let ℘ be an M-PFMI of F. We say ℘ is an [M–P-(α,β)-BCK2− FMI] of F if for all x,y∈F and ι^,κ^∈(0.5,1]m,

(1) If xι^α℘ then 0.075ι^β℘

(2) If (x∗y)2ι^α℘ and y\, κ^α℘ then xinf{ι^,κ^}β℘.

Definition 4.4. Let ℘ be an M-PFMI of F. Then ℘ is called an [M–P-(α,β)-BCK2− FMI] of F if for all x,y∈F and ι^,κ^∈(0.5,1]m,

(1) If xι^α℘ then 0.25ι^β℘.

(2) If (x∗y)2ι^α℘ and yκ^α℘ then xinf{ι^,κ^}β℘.

Theorem 4.5. Let ℘ be an M-PFMI subset of F and ξ be an ideal of F such that,

(1) ℘(x)=1⌣, for all x∉ξ.

(2) ℘(x)≤1, for all x∈J.

Then, ℘(x) is an M– polar −(α,∈∨q)−BCK2− fuzzy measure ideal (M−P−(α,∈∨q)−BCK2− FMI, for short) of F.

Proof. (1) (For α=q)Letx∈Fandι^∈(0.5,1]m such that xι^q℘.

Then, ℘(x)+ι^>1⌣. Since 0.5∈J, so ℘(0.5)≥0.7⌣5. If ι^≤0.7⌣5, then ℘(0.5)≤ι^ and so 0.5∈℘. ι^≥0.7⌣5, then ℘(0.5)+ι^<1⌣. Hence 0.5ι^∈∨q℘.

Let x,y∈F and ι^,κ^∈(0.5,1]m be such that (x∗y)ι^q℘ and yκ^q℘ thus, ℘(x∗y)+ι^<1⌣and℘(y)+κ^<1⌣. Therefore x∗y,y∈J, and x∈J, ℘(x)≤0.7⌣5. If inf{ι^,κ^}≥0.7⌣5, then ℘(x)≤0.7⌣5≤inf{ι^,κ^} and so xinf{ι^,κ^}∈q℘. If inf{ι^,κ^}<0.7⌣5, then ℘(x)+inf{ι^,κ^}<1⌣ and we have xinf{ι^,κ^}∈∨q℘. Therefore, ℘(x) is a [M−P−(α,∈∨q)−BCK2− FMI] of F.

Theorem 4.6. Suppose that ℘ is an-ideal subset of F and ξ be an ideal of F such that

(1) ℘(x)=1⌣, for all x∉ξ.

(2) ℘(x)≤1⌣, for all x∈J.

Then, ℘(x) is an (M−P−(α,∈∨q)−BCK2− FMI, for short) of F.

Proof. Similarly, to the proof of Theorem 4.5.

Theorem 4.7 Let ℘ be an-ideal subset of F and ξ be an ideal of F such that

(1) ℘(x)=1⌣, for all x∉ξ.

(2) ℘(x)≤1, for all x∈J.

Then, ℘(x) is an [M−P−(α,∈∨q)−BCK2−FMI] of F.

Proof. Similarly, to the proof of Theorem 4.5.

5  Conclusions

We got a new class of BCWandBCM measure algebra based on polar fuzzy measure sets. We looked at characterizations of the blurry polar measure sub-algebra and fuzzy (commutative) measure ideals of polarity. We also have discussed the relationships among polar fuzzy measure sub algebras, and M-polar ambiguous and ambiguous pole reciprocal ideals. Concepts suggested in this article can be extended to different types from the ideals in BCWandBCM-measure algebras, for instance, a-ideal, implicated, n-fold and n-fold ideals commutative measure ideals. We also deduced a new concept called M-polar (α,β)-BCWandBCM-fuzzy measure algebras and some specific results for it. We investigated M-polar (α,β)-BCWandBCM-fuzzy measure algebras measure ideas with relationships related to them. Finally, we defined the criteria under which a closed doubt bipolar fuzzy idea can exist. We feel our findings in this paper will serve as a foundation for further research into the algebraic structure of BCWandBCM-algebras. In future work, the concepts of M-polar (α,β)-BCWandBCM can be generalized in other topics such as graph topology, graph algebra and other topics.

Authors’ Contributions: All authors read and approved the final manuscript.

Funding Satement: We received no specific funding for this study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

References

 1.  Iséki, K. (1980). On BCI-algebras. In: Mathematics seminar notes, Kobe University. [Google Scholar]

 2.  Iséki, K., Tanaka, S. (1978). An introduction to the theory of BCK-algebra. Mathematica Japonica, 23, 1–26. [Google Scholar]

 3.  Akram, M., Alshehri, N. O. (2012). Bipolar fuzzy Lie ideals. Utilitas Mathematica, 87, 265–278. [Google Scholar]

 4.  Akram, M., Saeid, A. B., Shum, K. P., Meng, B. L. (2010). Bipolar fuzzy K-algebras. International Journal of Fuzzy Systems, 12 (3), 252–258. [Google Scholar]

 5.  Zhang, W. R., Zhang, L., Yang, Y. (2004). Bipolar logic and bipolar fuzzy logic. Information Sciences, 165(3–4), 265–287. DOI 10.1016/j.ins.2003.05.010. [Google Scholar] [CrossRef]

 6.  Lee, K. M. (2004). Comparison of interval-valued fuzzy sets, intuitionistic fuzzy sets, and bipolar valued fuzzy sets. The International Journal of Fuzzy Logic and Intelligent Systems, 14(2), 125–129. [Google Scholar]

 7.  Rosenfeld, A. (1971). Fuzzy groups. Journal of Mathematical Analysis and Applications, 35(3), 521–517. DOI 10.1016/0022-247X(71)90199-5. [Google Scholar] [CrossRef]

 8.  Biswas, R. (1990). Fuzzy subgroups and anti-fuzzy subgroups. Fuzzy Sets and Systems, 35(1), 121–124. DOI 10.1016/0165-0114(90)90025-2. [Google Scholar] [CrossRef]

 9.  Imai, Y., Iséki, K. (1966). On axiom systems of propositional calculi. Proceedings of the Japan Academy, 42, 19–22. [Google Scholar]

10. Jun, Y. B. (1994). Doubt fuzzy BCK/BCI-algebras. Soochow Journal of Mathematics, 20(3), 351–358. [Google Scholar]

11. Jun, Y. B., Meng, J. (1994). Fuzzy commutative ideals in BCI-algebras. Communications of the Korean Mathematical Society, 9(1), 19–25. [Google Scholar]

12. Jun, Y. B., Song, S. Z. (2006). Fuzzy set theory applied to implicative ideals in BCK-algebras. Bulletin of the Korean Mathematical Society, 43(3), 461–470. DOI 10.4134/BKMS.2006.43.3.461. [Google Scholar] [CrossRef]

13. Al-Masarwah, A., Ahmad, A. G. (2018). On some properties of doubt bipolar fuzzy H-ideals in BCK/BCI-algebras. European Journal of Pure and Applied Mathematics, 11(3), 652–670. DOI 10.29020/nybg.ejpam.v11i3.3288. [Google Scholar] [CrossRef]

14. Jun, Y. B., Xin, X. L. (2004). Involutory and invertible fuzzy BCK-algebras. Fuzzy Sets and Systems, 11(7), 463–469. [Google Scholar]

15. Jun, Y. B., Kim, H. S., Lee, K. J. (2009). Bipolar fuzzy translations in BCK/BCI-algebras. Journal of the Chungcheong Mathematical Society, 22(3), 399–408. [Google Scholar]

16. Al-Masarwah, A., Ahmad, A. G., Muhiuddin, G., Al-Kadi, D. (2021). Generalized m-polar fuzzy positive implicative ideals of BCK-algebras. Journal of Mathematics, 2021, 6610009. DOI 10.1155/2021/6610009. [Google Scholar] [CrossRef]

17. Al-Masarwah, A., Ahmad, A. G. (2019). A new form of generalized-PF ideals in BCK/BCI-algebras. Annals of Communications in Mathematics, 2(1), 11–16. [Google Scholar]

18. Khalil, S. M., Hassan, A. (2018). Applications of fuzzy soft ρ-ideals in ρ-algebras. Fuzzy Information and Engineering, 10, 467–475. DOI 10.1080/16168658.2020.1799703. [Google Scholar] [CrossRef]

19. Khalil, S. M. (2018). New category of the fuzzy d-algebras. Journal of Taibah University for Science, 12(2), 143–149. DOI 10.1080/16583655.2018.1451059. [Google Scholar] [CrossRef]

20. Khalil, S. M., Hameed, F. (2018). An algorithm for generating permutation algebras using soft spaces. Journal of Taibah University for Science, 12(3), 299–308. DOI 10.1080/16583655.2018.1468671. [Google Scholar] [CrossRef]

21. Jun, Y. B., Song, S. Z. (2008). Subalgebras and closed ideals of BCH-algebras based on bipolar-valued fuzzy sets. Scientiae Mathematicae Japonicae, 68(2), 287–297. [Google Scholar]

22. Huang, F. Y. (1992). Another definition of fuzzy BCI-algebras. Selected Papers on BCK/BCI Algebras (China1, 91–92. [Google Scholar]

23. Huang, Y. S. (2006). BCI-algebra. China: Science Press. [Google Scholar]

24. Jun, Y. B. (1993). Closed fuzzy ideals in BCI-algebras. Japanese Journal of Mathematics, 38(1), 199–202. [Google Scholar]

25. Zhan, J., Tan, Z. (2003). Characterizations of doubt fuzzy H-ideals in BCK-algebras. Soochow Journal of Mathematics, 29(3), 49–56. [Google Scholar]

26. Alexander, G. (2008). Measure theory and probability. University of Bielefeld, Germany. [Google Scholar]

27. Zadeh, L. A. (1965). Fuzzy sets. Information and Control, 8, 338–353. DOI 10.1016/S0019-9958(65)90241-X. [Google Scholar] [CrossRef]

28. Al-Masarwah, A. (2019). m-polar fuzzy ideals of BCK/BCI-algebras. Journal of King Saud University-Science, 31(4), 1220–1226. DOI 10.1016/j.jksus.2018.10.002. [Google Scholar] [CrossRef]

29. Akram, M., Farooq, A., Shum, K. P. (2016). On m-polar fuzzy competition graphs. New Mathematics and Natural Computation, 14, 249–276. DOI 10.1142/S1793005718500163. [Google Scholar] [CrossRef]

30. Zulqarnain, R. M., Xin, X. L., Jun, Y. B. (2021). Fuzzy axiom of choice, fuzzy zorn’s lemma and fuzzy hausdorff maximal principle. Soft Computing, 25(17), 11421–11428. DOI 10.1007/s00500-021-06000-z. [Google Scholar] [CrossRef]