The Fractional Investigation of Some Nonlinear Partial Differential Equations by Using an Efficient Procedure

1  Introduction

The integral and derivative of fractional order are considered to be common topics of fractional calculus (FC) due to their numerous applications in applied sciences. This topic has gained much popularity among researchers because of its popularity and significance while modelling various procedures in nature. For example, these problems are increasingly being applied to equations in fluid flow, diffusion, polymer physics, electric network rheology, relaxation, reaction-diffusion, diffusive transport akin to diffusion, turbulence, anomalous diffusion, porous structures, and dynamical processes in complex systems, as well as a variety of other physical phenomena [1,2]. In the last few decades, the early theory and development regarding fractional derivatives and fractional differential equations (FPDEs) have been observed rapidly. The subject is further explained and extended by the authors, such as Abbas et al. [3], Hilfer [4], Kilbas et al. [5], and Legnani et al. [6]. The systematic understanding of FC like uniqueness and existence and theoretic issues related to FPDEs are the core themes of their work. Other concepts of FC can be read in the papers of Hernández et al. [7] where the recent advances in the theory of FPDEs are presented. Magin et al. [8] and Mainardi [9] looked into FC’s applications in interdisciplinary fields like image processing and control theory.

It is very rare to calculate the exact solution of non-linear FPDEs in the literature. Using linearization, successive, or perturbation methods, only approximate solutions can be obtained. Iterative Laplace transform method [10], Adomian decomposition method [11], Homotopy analysis method [12], operational matrix method [13], fractional differential transform method [14], Fourier transform technique [15], operational calculus method [16], Variational iteration method [17], Sumudu transform method [18], multistep generalised differential transform method [19], iterative reproducing kernel method [20], Homotopy perturbation method [21], and Numerical multistep method [22].

In many cases, analytical or exact solutions are very difficult to investigate. Therefore, mathematicians have tried to develop and use several numerical techniques with fractional derivative and integral operators [23–25]. In this connection, in 2017, Li et al. presented three efficient techniques, namely the finite difference method, the Galerkin finite element method, and the spectral method, for the solutions of some FPDEs [26]. Atangana et al. used the fundamental theorem of fractional calculus along with the Lagrange interpolation polynomial and investigated the solution of the Keller-Segel model [27] in 2018. In 2020, the shifted Chebyshev polynomials with some assumptions were used to find the solutions of some FPDEs with variable coefficients [28]. Similarly, in 2020, the finite difference method and operational matrix method were used to determine the solution of Riez-space FPDEs [29].

Without linearization, perturbation, and discretization, the residual power series method (RPSM) is an effective and uncomplicated technique for constructing a power series (PS) solution for FPDEs. Unlike the traditional PS method, the RPS method does not require a recursion relation or comparison of the coefficients of the related terms. A series of algebraic expressions are obtained to calculate the PS coefficients. The methodology’s main advantage is that it relies on simpler and more accurate derivation as compared to other techniques that are based on integration. This method is a different way of solving FPDEs in theory [30]. The Laplace residual power series method (LRPSM) [31] is a combination of the Laplace transform (LT) and the RPSM. In the procedure of LRPSM, the first Laplace transformation is used to simplify the targeted problem into new algebraic equations. The RPSM is then implemented to obtain the series solution. In the end, the inverse Laplace transform is applied to attain the required solution. The LRPSM required less calculation with less time and more accuracy.

In this article, the LRPSM is used to solve the time and space FPDEs. The aim of the study is to use LRPSM with space-time fractional derivatives of the form to obtain numerical solutions to nonlinear fractional partial differential equations.

Dtξu(ζ,t)+u(ζ,t)Dζρu(ζ,t)=g(ζ),0<ξ,ρ≤1,(1)

where u(ζ,t) is assumed to be a spatial function that vanishes accordingly for ζ<0 and t<0, and are parameters describing the order of the fractional derivatives of ζ and t, respectively. When dealing with fractional derivatives, Caputo sense [32] is applied.

The generalized LRPSM procedure is presented and then LRPSM algorithm is applied to solve few numerical problems. The results and the accuracy of the suggested technique is shown by tables and graphs. The graphical representation is done and the obtained solutions are vary closed to the actual solutions of each target problem. The fractional order LRPSM solutions provide the analysis of some useful dynamics of the given FPDE’s. The tables have shown that LRPSM has the higher degree accuracy. LRPSM is comparatively a very simple and direct procedure to evaluate the solutions of non-linear FPDEs and their systems. The proposed required fewer calculations to compute the non-linear terms in each problem.

The article layout as follows. The fundamental concepts regarding FC are described in Section 2, the basic methodology is discussed in Section 3, the effectiveness of LRPSM is confirmed by some test models in Section 4, results and discussion are in Section 5 and the conclusion is given in Section 6.

2  Basic Definitions

2.1 Definition

The Caputo’s derivative of f(t) is given as [33]

CDtξf(t)=1Γ(1−ζ)∫0t(t−s)−ζf′(s)ds,0<ζ≤1.

2.2 Definition

Let T>0, g∈H1(0,T), and ζ∈(0,1); then the ζth Caputo-Fabrizio derivative of f is given as

CFDζf(t)=12M(ζ)(2−ζ)1−ζ∫0tf′(s)exp[−m(t−s)]ds,(2)

where m=ζ1−ζ, and M(ζ) is a normalizing function depending on ζ, such that M(o)=M(1)=1.

In [34], Losada and Nieto provided an explicit formula for M(ζ) as M(ζ)=22−ζ. In this case, the Caputo-Fabrizio derivative Eq. (2) is reduced to

CFDζf(t)=11−ζ∫0tf′(s)exp[−m(t−s)]ds.(3)

2.3 Definition

Let f∈H1(a,b), a<b and ζ∈[0,1]. The Caputo Atangana-Baleanu fractional derivative [35,36] of f of order ζ is defined by

ABCDζf(t)=M(ζ)1−ζ∫atf′(s)Eζ[−ζ(t−s)ζ1−ζ]ds,(4)

where Eζ(t) is Mittag-Leffler function defined [4,7] Eζ(t)=∑n=0∞tnΓ(nζ+1) and M(ζ) is a normalizing function.

2.4 Theorem

(Fractional Taylor’s formula [37]) Let us assume that f(t) has a FPS representation at t=0 of the form

f(t)=∑n=0∞bntnξ,0<m−1<ξ≤m,0≤t<R.(5)

If f(t)∈C[0,R] and Dtnξf(t)∈C(0,R) for n=0,1,2,…, then the coefficients bn in the Eq. (1) can be written as

bn=(Dtnξf)(0)Γ(nζ+1),(6)

where Dtnξ=Dtξ.Dtξ⋯Dtξ(n−times).

2.5 Theorem

Suppose that f0 has a fractional power series expression of the form at t=t0

u(ζ,t)=∑θ=0∞fθ(ζ)(t−t0)θξ,x∈I,t0≤t<t0+R.

If Dtθξu(ζ,t),θ=0,1,… are continuous I×(t0,t0+R), then the coefficient fθ(ζ) can be defined as

fθ(ζ)=Dθξu(ζ,t0)Γ(1+θξ).

2.6 Definition

A PS representation of the form

∑τ=0∞pτ(τ−τ0)τξ=p0+pτ(τ−τ0)ξ+pτ(τ−τ0)2ξ+⋯,(7)

where 0≤n−1<ξ≤n, τ≤τ0 is known as fractional power series (FPS) about τ0, where Pn the coefficient of the series. If τ0=0 then FPS will be reduced to a fractional Maclaurin series at that point.

2.7 Definition

The extension form of PS is

∑n=0∞fθ(ζ)(t−t0)θξ,(8)

is called multi FPS about t=t0, where fn(ζ) are the coefficients of multi FPS.

2.8 Theorem

Consider that u(ζ,t) has a many FPS representation at t=t0 of the form

u(ζ,t)=∑j=0∞fj(ζ)(t−t0)jξ,ζ∈I,t0≤t<t0+R.

If Djξu(ζ,t), j=0,1,2,… are continuous on I×(t0,t0+R), then fj(ζ)=Djξu(ζ,t0)Γ(1+jξ).

3  The Methodology of LRPSM

Here, we will go through the procedure that LRPSM takes to solve time and space FPDEs.

Dtξu(ζ,t)+u(ζ,t)Dζρu(ζ,t)=g(ζ),0<ξ,ρ≤1,(9)

with initial condition (IC)

u(ζ,0)=f0(ζ),(10)

We apply the LT to Eq. (9), i.e.,

Lt[Dtξu(ζ,t)]=−Lt[Lt−1(U(ζ,s))DζρLt−1(U(ζ,s))−g(ζ)].(11)

By the fact that Lt[Dtξμ(ζ,t)]=sξLt[μ(ζ,t)]−sξ−1μ(ζ,0) and using the initial condition (IC) Eq. (10), we rewrite Eq. (11) as

U(ζ,s)=f0(ζ)s−1sξLt[Lt−1(U(ζ,s))DζρLt−1(U(ζ,s))−g(ζ)](12)

where U(ζ,s)=Lt[u(ζ,t)].

We write the Eq. (12) as the following expansion:

U(ζ,s)=∑n=0∞fn(ζ)snξ+1.(13)

The kth-truncated series of Eq. (13) takes the form

Uk(ζ,s)=∑n=0kfn(ζ)snξ+1=f0(ζ)s∑n=1kfn(ζ)snξ+1.(14)

As stated in [31], the definition of Laplace Residual (LR) function to Eq. (12) is

LtRes(ζ,s)=U(ζ,s)−f0(ζ)s+1sξLt[Lt−1(U(ζ,s))DζρLt−1(U(ζ,s))−g(ζ)],(15)

and the kth-LR function of Eq. (15) is

LtResU,k(ζ,s)=Uk(ζ,s)−f0(ζ)s+1sξLt[Lt−1(Uk(ζ,s))DζρLt−1(Uk(ζ,s))−g(ζ)],

here are some properties arise in the RPSM [31], to point out some facts:

•   LtResk(ζ,s)=0 and limk→∞=LtRes(ζ,s) for each s>0.

•   lims→∞sLtRes(ζ,s)=0⇒lims→∞sLtResk(ζ,s)=0.

•   limx→∞skξ+1LtRes(ζ,s)=lims→∞skξ+1LtResk(ζ,s)=0,0<ξ≤1,k=1,2,3,…

Therefore, to determine the coefficient functions fn(ζ), we solve recursively, the following system

lims→∞skξ+1LtResk(ζ,s)=0.0<ξ≤1.k=1,2,3,…

We apply the inverse LT to Uk(ζ,s), to obtain the kth-approximate solution uk(ζ,t).

4  Numerical Problems

4.1 Problem 1

Consider the time and space FPDE [38]

Dtξu(ζ,t)+u(ζ,t)Dζρu(ζ,t)=ζ,0<ξ,ρ≤1,(16)

with IC

u(ζ,0)=1,(17)

exact solution of Eq. (16) at ξ=ρ=1 is

u(ζ,t)=ζtanh(t)+sech(t).(18)

We apply the LT to Eq. (16) and using of Eq. (17), i.e.,

U(ζ,s)=1s−1sξLt[Lt−1(U(ζ,s))DζρLt−1(U(ζ,s))−ζ](19)

The kth-truncated series of Eq. (19) takes the form

Uk(ζ,s)=1s+∑n=1kfn(ζ)snξ+1.(20)

and the kth LR function of Eq. (19)

LtResU,k(ζ,s)=Uk(ζ,s)−f0(ζ)s+1sξLt[Lt−1(Uk(ζ,s))DζρLt−1(Uk(ζ,s))−ζ],(21)

Now, to determine fk(ζ), k=1,2,3,…, by putting the kth-truncated series Eq. (20) into the kth Laplace Residual function Eq. (21), multiply the resulting equation by skξ+1, and then recursively solve the relation lims→∞[skξ+1Resk(ζ,s)]=0, k=1,2,3,… for fk(ζ). The first few elements of the sequences fk(ζ)

f1(ζ)=ζ,f2(ζ)=−ζ1−ρΓ(2−ρ),f3(ζ)=−ζ2−ρΓ(2ξ+1)Γ(1+ξ)2Γ(2−ρ),⋮.(22)

Putting the values of fn(ζ), (n≥1) in Eq. (4), we have

U(ζ,s)=f0(ζ)s+f1(ζ)sξ+1+f2(ζ)s2ξ+1+f3(ζ)s3ξ+1+⋯,U(ζ,s)=1s+ζsξ+1−(ζ1−ρΓ(2−ρ))1s2ξ+1−(ζ2−ρΓ(2ξ+1)Γ(1+ξ)2Γ(2−ρ))1s3ξ+1+⋯.(23)

Applying inverse LT to Eq. (23), we get

u(ζ,t)=1+ζtξΓ(ξ+1)−(ζ1−ρΓ(2−ρ))t2ξΓ(2ξ+1)−(ζ2−ρΓ(2ξ+1)Γ(1+ξ)2Γ(2−ρ))t3ξΓ(3ξ+1)+⋯.(24)

putting ξ=ρ=1 in Eq. (24), we get the solution in closed form

u(ζ,t)=ζtanh(t)+sech(t).(25)

4.2 Problem 2

Consider the time and space FPDE [38]

Dtξu(ζ,t)+u(ζ,t)Dζρu(ζ,t)=1,0<ξ,ρ≤1,(26)

with initial condition

u(ζ,0)=−ζ,(27)

exact solution of Eq. (26) at ξ=ρ=1 is

u(ζ,t)=2ζ−2t+t22(t−1).(28)

We apply the LT to Eq. (26) and making use of Eq. (27) i.e.,

U(ζ,s)=−ζs−1sξLt[Lt−1(U(ζ,s))DζρLt−1(U(ζ,s))−1].(29)

The kth-truncated series of Eq. (29) takes the form

Uk(ζ,s)=−ζs+∑n=1kfn(ζ)snξ+1.(30)

and the kth LR function of Eq. (29)

LtResU,k(ζ,s)=Uk(ζ,s)+ζs+1sξLt[Lt−1(Uk(ζ,s))DζρLt−1(Uk(ζ,s))−1].(31)

Now, to determine fk(ζ), k=1,2,3,…, we substitute the kth-truncated series Eq. (30) into the kth Laplace Residual function Eq. (31), multiply the resulting equation by skξ+1, and then recursively solve the relation lims→∞[skξ+1Resk(ζ,s)]=0, k=1,2,3,… for fk(ζ). First few elements of the sequences fk(ζ)

f1(ζ)=(Γ(2−ρ)−ζ2−ρΓ(2−ρ)),f2(ζ)=−(ζ3−2ρΓ(3−ρ)Γ(2−ρ)+ζ3−2ρ−ζ1−ρΓ(2−ρ)Γ(2−ρ)2),f3(ζ)=−(ζ4−3∗ρΓ(4−2ρ)Γ(3−ρ)Γ(2−ρ)Γ(3−2ρ)Γ(4−3ρ)+ζ4−3ρΓ(4−2ρ)Γ(2−ρ)2Γ(4−3ρ)−(ζ2−2ρΓ(3−ρ)Γ(2−ρ)−ζ4−3ρΓ(3−2ρ)Γ(2−ρ)2)Γ(2ξ+1)Γ(ξ+1)2+ζ4−3ρΓ(2−ρ)2Γ(3−2ρ)+ζ4−3ρ−ζ2−2ρΓ(2−ρ)Γ(2−ρ)3),⋮.(32)

Putting the values of fn(ζ), (n≥1) in Eq. (30), we have

U(ζ,s)=f0(ζ)s+f1(ζ)sξ+1+f2(ζ)s2ξ+1+f3(ζ)s3ξ+1+⋯,

U(ζ,s)=−ζs+(Γ(2−ρ)−ζ2−ρΓ(2−ρ))1sξ+1−(ζ3−2ρΓ(3−ρ)Γ(2−ρ)+ζ3−2ρ−ζ1−ρΓ(2−ρ)Γ(2−ρ)2)1s2ξ+1−(ζ4−3ρΓ(4−2ρ)Γ(3−ρ)Γ(2−ρ)Γ(3−2ρ)Γ(4−3ρ)+ζ4−3ρΓ(4−2ρ)Γ(2−ρ)2Γ(4−3ρ)−(ζ2−2ρΓ(3−ρ)Γ(2−ρ)−ζ4−3ρΓ(3−2ρ)Γ(2−ρ)2)Γ(2ξ+1)Γ(ξ+1)2+ζ4−3ρΓ(2−ρ)2Γ(3−2ρ)+ζ4−3ρ−ζ2−2ρΓ(2−ρ)Γ(2−ρ)3)1s3ξ+1+⋯.(33)

Applying inverse LT to Eq. (33), we get

u(ζ,t)=−ζ+(Γ(2−ρ)−ζ2−ρΓ(2−ρ))tξΓ(ξ+1)−(ζ3−2ρΓ(3−ρ)Γ(2−ρ)+ζ3−2ρ−ζ1−ρΓ(2−ρ)Γ(2−ρ)2)t2ξΓ(2ξ+1)−(ζ4−3∗ρΓ(4−2ρ)Γ(3−ρ)Γ(2−ρ)Γ(3−2ρ)Γ(4−3ρ)+ζ4−3ρΓ(4−2ρ)Γ(2−ρ)2Γ(4−3ρ)−(ζ2−2ρΓ(3−ρ)Γ(2−ρ)−ζ4−3ρΓ(3−2ρ)Γ(2−ρ)2)Γ(2ξ+1)Γ(ξ+1)2+ζ4−3ρΓ(2−ρ)2Γ(3−2ρ)+ζ4−3ρ−ζ2−2ρΓ(2−ρ)Γ(2−ρ)3)t3ξΓ(3ξ+1)+⋯.(34)

Putting ξ=ρ=1 in Eq. (34), we get the solution in closed form

u(ζ,t)=−ζ+(1−ζ)t+(−2ζ+1)t22+(−6ζ+3)t36+⋯,=−(ζ−t+η22)(1+t+t2+⋯),=(2ζ−2t+η2)2(t−1).(35)

4.3 Problem 3

Consider the time and space FPDE [38]

Dtξu(ζ,t)+u(ζ,t)Dζρu(ζ,t)=0,0<ξ,ρ≤1,(36)

with IC

u(ζ,0)=ζ+1,(37)

exact solution of Eq. (36) at ξ=ρ=1 is

u(ζ,t)=1+ζ1+t.(38)

We apply the LT to Eq. (36) and making use of Eq. (37), i.e.,

U(ζ,s)=ζ+1s−1sξLt[Lt−1(U(ζ,s))DζρLt−1(U(ζ,s))](39)

The kth-truncated series of Eq. (39) takes the form

Uk(ζ,s)=ζ+1s+∑n=1kfn(ζ)snξ+1.(40)

and the kth Laplace Residual function of Eq. (39)

LtResU,k(ζ,s)=Uk(ζ,s)+ζ+1s+1sξLt[Lt−1(Uk(ζ,s))DζρLt−1(Uk(ζ,s))],(41)

Now, to determine fk(ζ), k=1,2,3,…, we substitute the kth-truncated series Eq. (40) into the kth LR function Eq. (41), multiply the resulting equation by skξ+1, and then solve recursively the relation lims→∞[skξ+1Resk(ζ,s)]=0, k=1,2,… for fk(ζ). The first few elements of the sequences fk(ζ) as follows:

f1(ζ)=−(ζ2−ρ+ζ1−ρΓ(2−ρ)),f2(ζ)=(Γ(3−ρ)ζ3−2ρ+Γ(3−ρ)ζ2−2ρΓ(2−ρ)Γ(3−2ρ)+ζ3−2ρ+ζ2−2ρΓ(2−ρ)2),⋮.(42)

Putting the values of fn(ζ), (n≥1) in Eq. (40), we have

U(ζ,s)=f0(ζ)s+f1(ζ)sξ+1+f2(ζ)s2ξ+1+⋯,U(ζ,s)=ζ+1s−(ζ2−ρ+ζ1−ρΓ(2−ρ))1sξ+1+(Γ(3−ρ)ζ3−2ρ+Γ(3−ρ)ζ2−2ρΓ(2−ρ)Γ(3−2ρ)+ζ3−2ρ+ζ2−2ρΓ(2−ρ)2)1s2ξ+1+⋯.(43)

Applying inverse LT, we get

u(ζ,t)=ζ+1−(ζ2−ρ+ζ1−ρΓ(2−ρ))tξΓ(ξ+1)+(Γ(3−ρ)ζ3−2ρ+Γ(3−ρ)ζ2−2ρΓ(2−ρ)Γ(3−2ρ)+ζ3−2ρ+ζ2−2ρΓ(2−ρ)2)t2ξΓ(2ξ+1)+⋯,(44)

putting ξ=ρ=1, the solution in closed form is

u(ζ,t)=1+ζ1+t.(45)

5  Results and Discussion

Figs. 1 and 2 present the 2D and 3D plots of the Exact and LRPSM solutions at different fractional order’s of Example 4.1. The exact and LRPSM solutions in integer order are in closed contact with each other. It is observed that the fractional solutions are convergent towards integer order solution, which confirmed the validity of the suggested method for fractional order problems. Tables 1, 2 and 4 discuss the absolute error associated with LRPSM solution at different time level and spaces of Examples 4.1, 4.2 and 4.3. It is noted that as the fractional-orders approach to integer order, the method’s accuracy is increased. This phenomenon supports the applicability of LRPSM to fractional-order solutions. The tables have confirmed the convergence of the fractional solutions towards integer order solutions. In Table 3, the LRPSM solutions are compared with ADM solutions. The solutions comparison has shown that LRPSM has greater accuracy as compared to ADM. Figs. 3 and 4 show the 2D and 3D plots of exact and LRPSM solutions at different fractional orders of Example 4.2. Also, Figs. 5 and 6 provide the 2D and 3D plots of the exact and LRPSM fractional solutions of Example 4.3, respectively. The overall discussion of the graphs and tables reveals that the LRPSM solutions are more accurate and effective. The overall comparison of LRPSM and exact solutions provided the sufficient and closed relation with each other. The convergence of fractional solutions towards the integer order solution is observed. Table 5 represents the list nomenclatures that have been used to abbreviate various kinds of important names in the paper.

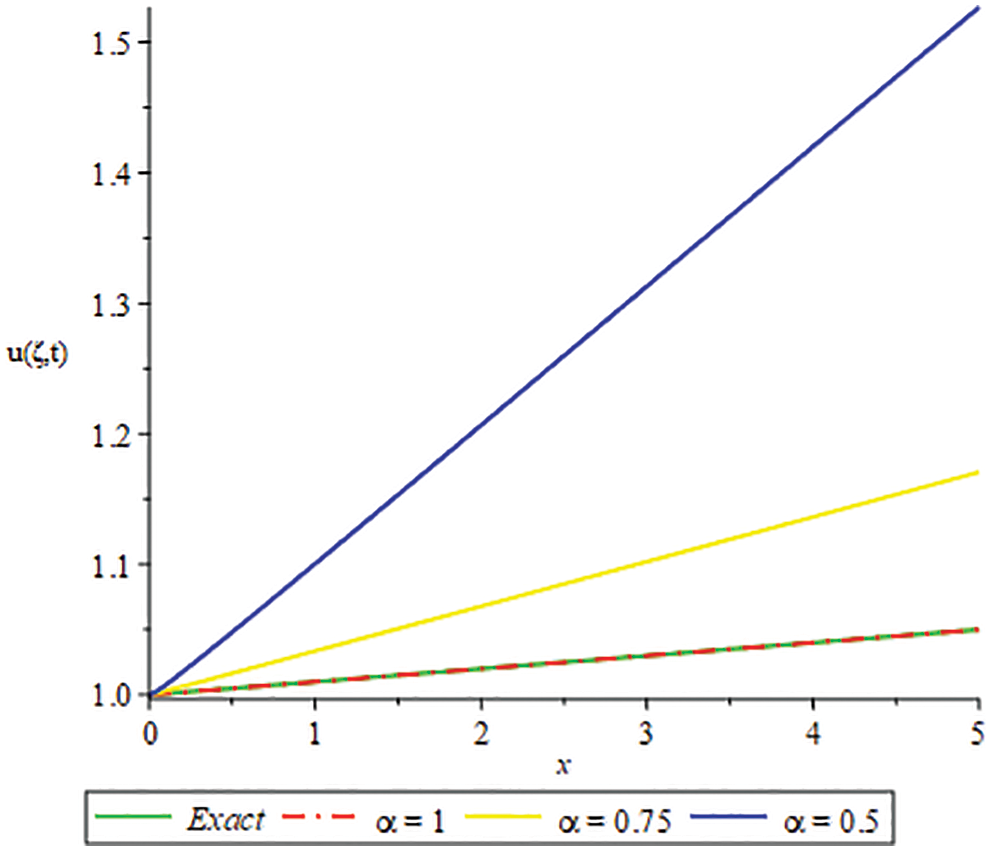
Figure 1: 2D plots for exact and LRPSM solution of Example 4.1 for different values of ξ

Figure 2: 3D plots exact and LRPSM solution of Example 4.1 for different values of ξ

Figure 3: 2D plots for exact and LRPSM solution of Example 4.2 for different values of ξ

Figure 4: 3D plots for exact and LRPSM solution of Example 4.2 for different values of ξ

Figure 5: 2D plots for exact and LRPSM solution of Example 4.3 for different values of ξ

Figure 6: 3D plots for exact and LRPSM solution of Example 4.3 for different values of ξ

6  Conclusion

The present article is related to the approximate analytical solutions of some nonlinear fractional partial differential equations using the Laplace residual power series method. The fractional derivatives in each targeted problem are represented by the Caputo operator. First, the proposed scheme is discussed for the general nonlinear problem and the few nonlinear problems related to nonlinear fractional partial differential equations are solved by using the proposed method. The obtained results are compared with the exact solution of each problem. The fractional order solutions are analyzed by using the suggested method successfully. It is observed that the present technique is the most suitable tool for the solutions of nonlinear fractional partial differential equations and possesses a higher degree of accuracy. In conclusion, this new hybrid technique is straightforward to solve the nonlinear fractional problems and can be used effectively in other branches of applied sciences.

Authors Contribution: Fairouz Tchier (Investigation, Draft Writing), Hassan Khan (Supervision), Shahbaz Khan (Methodology, Investigation) Poom Kumam (Funding, Draft Writing), Ioannis Dassios (Draft Writing).

Availability of Data and Material: Not applicable.

Funding Statement: Researchers Supporting Project No. (RSP-2021/401), King Saud University, Riyadh, Saudi Arabia.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

References

 1.  Khan, T., Zaman, G., El-Khatib, Y. (2021). Modeling the dynamics of novel coronavirus (COVID-19) via stochastic epidemic model. Results in Physics, 24, 104004. [Google Scholar]

 2.  Jain, S., El-Khatib, Y. (2021). Stochastic COVID-19 model with fractional global and classical piecewise derivative. Results in Physics, 30, 104788. [Google Scholar]

 3.  Abbas, S., Benchohra, M., N’Guerekata, G. M. (2015). Advanced fractional differential and integral equations. Hauppauge, New York, USA: Nova Publishers. [Google Scholar]

 4.  Hilfer, R. (2000). Applications of fractional calculus in physics. Singapore: River Edge, World ScientificPublishing Co. [Google Scholar]

 5.  Kilbas, A. A., Srivastava, H. M., Trujillo, J. J. (2006). Theory and applications of fractional differential equations, vol. 204. Amsterdam: Elsevier Science B.V. [Google Scholar]

 6.  Legnani, W., Moschandreou, T. E., Reyhanoglu, M. (2020). Nonlinear systems: Theoretical aspects and recent applications. London, UK: IntechOpen. [Google Scholar]

 7.  Hernández, E., O’Regan, D., Balachandran, K. (2010). On recent developments in the theory of abstract differential equations with fractional derivatives. Nonlinear Analysis: Theory, Methods & Applications, 73(10), 3462–3471. [Google Scholar]

 8.  Magin, R., Ortigueira, M. D., Podlubny, I., Trujillo, J. J. (2011). On the fractional signals and systems. Signal Process, 91, 350–371. [Google Scholar]

 9.  Mainardi, F. (2010). Fractional calculus and waves in linear viscoelasticity: An introduction to mathematical models. London, UK: Imperial College Press. [Google Scholar]

10. Li, X., Li, S. (2021). A fast element-free Galerkin method for the fractional diffusion-wave equation. Applied Mathematics Letters, 122, 107529. [Google Scholar]

11. Noeiaghdam, S., Sidorov, D., Wazwaz, A. M., Sidorov, N., Sizikov, V. (2021). The numerical validation of the adomian decomposition method for solving volterra integral equation with discontinuous kernels using the CESTAC method. Mathematics, 9(3), 260. [Google Scholar]

12. Saratha, S. R., Krishnan, G. S. S., Bagyalakshmi, M. (2021). Analysis of a fractional epidemic model by fractional generalised homotopy analysis method using modified Riemann-Liouville derivative. Applied Mathematical Modelling, 92, 525–545. [Google Scholar]

13. Saha Ray, S. (2021). A new approach by two-dimensional wavelets operational matrix method for solving variable-order fractional partial integro-differential equations. Numerical Methods for Partial Differential Equations, 37(1), 341–359. [Google Scholar]

14. Kamil Jassim, H., Vahidi, J. (2021). A new technique of reduce differential transform method to solve local fractional PDEs in mathematical physics. International Journal of Nonlinear Analysis and Applications, 12(1), 37–44. [Google Scholar]

15. Candoğan, K., Altuntas, E. G., Iğci, N. (2021). Authentication and quality assessment of meat products by Fourier-transform infrared (FTIR) spectroscopy. Food Engineering Reviews, 13(1), 66–91. [Google Scholar]

16. Fahad, H. M., Fernandez, A. (2021). Operational calculus for caputo fractional calculus with respect to functions and the associated fractional differential equations. Applied Mathematics and Computation, 409, 126400. [Google Scholar]

17. Gonzalez-Gaxiola, O., Biswas, A., Ekici, M., Khan, S. (2022). Highly dispersive optical solitons with quadratic-cubic law of refractive index by the variational iteration method. Journal of Optics, 51(1),29–36. [Google Scholar]

18. Ahmed, S. A., Elzaki, T. M., Elbadri, M., Mohamed, M. Z. (2021). Solution of partial differential equations by new double integral transform (Laplace-Sumudu transform). Ain Shams Engineering Journal, 12(4), 4045–4049. [Google Scholar]

19. Aljahdaly, N. H., El-Tantawy, S. A. (2021). On the multistage differential transformation method for analyzing damping duffing oscillator and its applications to plasma physics. Mathematics, 9(4), 432. [Google Scholar]

20. Momani, S., Djeddi, N., Al-Smadi, M., Al-Omari, S. (2021). Numerical investigation for Caputo-Fabrizio fractional Riccati and Bernoulli equations using iterative reproducing kernel method. Applied Numerical Mathematics, 170, 418–434. [Google Scholar]

21. He, J. H., El-Dib, Y. O. (2021). Homotopy perturbation method with three expansions. Journal of Mathematical Chemistry, 59(4), 1139–1150. DOI 10.1007/s10910-021-01237-3. [Google Scholar] [CrossRef]

22. Du, Q., Gu, Y., Yang, H., Zhou, C. (2021). The discovery of dynamics via linear multistep methods and deep learning: Error estimation. arXiv preprint arXiv:2103.11488. [Google Scholar]

23. Abro, K. A., Atangana, A., Gómez-Aguilar, J. F. (2022). A comparative analysis of plasma dilution based on fractional integro-differential equation: An application to biological science. International Journal of Modelling and Simulation, 1–10. DOI 10.1080/02286203.2021.2015818. [Google Scholar] [CrossRef]

24. Souayeh, B., Ali Abro, K., Alfannakh, H., Al Nuwairan, M., Yasin, A. (2022). Application of Fourier sine transform to carbon nanotubes suspended in ethylene glycol for the enhancement of heat transfer. Energies, 15(3), 1200. DOI 10.3390/en15031200. [Google Scholar] [CrossRef]

25. Abro, K. A., Souayeh, B., Malik, K., Atangana, A. (2022). Chaotic characteristics of thermal convection at smaller verse larger prandtl number through fractal and fractional differential operators from nanofluid. International Journal of Modelling and Simulation, 1–12. DOI 10.1080/02286203.2021.2018261. [Google Scholar] [CrossRef]

26. Li, C., Chen, A. (2018). Numerical methods for fractional partial differential equations. International Journal of Computer Mathematics, 95(6–7), 1048–1099. DOI 10.1080/00207160.2017.1343941. [Google Scholar] [CrossRef]

27. Atangana, A., Alqahtani, R. T. (2018). New numerical method and application to Keller-Segel model with fractional order derivative. Chaos, Solitons & Fractals, 116, 14–21. DOI 10.1016/j.chaos.2018.09.013. [Google Scholar] [CrossRef]

28. Kheybari, S. (2021). Numerical algorithm to Caputo type time-space fractional partial differential equations with variable coefficients. Mathematics and Computers in Simulation, 182, 66–85. DOI 10.1016/j.matcom.2020.10.018. [Google Scholar] [CrossRef]

29. Srivastava, N., Singh, A., Kumar, Y., Singh, V. K. (2021). Efficient numerical algorithms for Riesz-space fractional partial differential equations based on finite difference/operational matrix. Applied Numerical Mathematics, 161, 244–274. DOI 10.1016/j.apnum.2020.10.032. [Google Scholar] [CrossRef]

30. Alquran, M., Ali, M., Alsukhour, M., Jaradat, I.  (2020). Promoted residual power series technique with laplace transform to solve some time-fractional problems arising in physics. Results in Physics, 19, 103667. DOI 10.1016/j.rinp.2020.103667. [Google Scholar] [CrossRef]

31. Eriqat, T., El-Ajou, A., Moa’ath, N. O., Al-Zhour, Z., Momani, S. (2020). A new attractive analytic approach for solutions of linear and nonlinear neutral fractional pantograph equations. Chaos, Solitons and Fractals, 138, 109957. DOI 10.1016/j.chaos.2020.109957. [Google Scholar] [CrossRef]

32. Gorenflo, R., Mainardi, F. (1997). Fractional calculus. In: Fractals and fractional calculus in continuum mechanics, pp. 223–276. Vienna: Springer. [Google Scholar]

33. Maachado, J. A. T., Baleanu, D., Chen, W., Sabatier, J. (2014). New trends in fractional dynamics. Journal of Vibration and Control, 20(7). DOI 10.1177/1077546313507. [Google Scholar] [CrossRef]

34. Losada, J., Nieto, J. J. (2015). Properties of a new fractional derivative without singular. Progress in Fractional Differentiation and Applications, 1(2), 87–92. [Google Scholar]

35. Atangana, A., Baleanu, D. (2016). New fractional derivative with non-local and non-singular kernel. Thermal Science, 20, 757–763. [Google Scholar]

36. Dutta, H., Akdemir, A., Atangana, A. (2020). Fractional order analysis: Theory, methods and applications. Hoboken, USA: John Willey and Sons Ltd. [Google Scholar]

37. El-Ajou, A. (2020). Taylor’s expansion for fractional matrix functions: Theory and applications. Journal of Mathematics and Computer Science, 21(1), 1–17. DOI 10.22436/jmcs.021.01.01. [Google Scholar] [CrossRef]

38. Shingareva, I., Lizrraga-Celaya, C. (2011). Solving nonlinear partial differential equations with maple and mathematica. Springer Science & Business Media. [Google Scholar]

39. Javed, I., Ahmad, A., Hussain, M., Iqbal, S. (2017). Some solutions of fractional order partial differential equations using adomian decomposition method. arXiv preprint arXiv:1712.09207. [Google Scholar]