Notes on Curves at a Constant Distance from the Edge of Regression on a Curve in Galilean 3-Space

1  Introduction

Klein pronounced a different definition of geometry in his introductory speech at the University of Erlangen in 1872. He explained that geometry, given by a subgroup G of a set and its symmetries, is the examination of invariants under this group [1]. This concept was first presented in a lecture, and it resulted in the emergence of numerous geometries. Galilean geometry is one of these geometries whose motions are the Galilean transformations of classical kinematics. Yaglom explained the basics of Galilean geometry in 1979 [2]. Then particularly, the geometry of ruled surfaces in this space has been largely improved in Röschel’s thesis [3].

One of the important research areas in differential geometry is the theory of curves examined in various spaces. In particular, it has been examined in a lot of papers and remarkable results have been obtained in the 3-dimensional Galilean space [4–10].

The notion of the curves at a constant distance from the edge of regression has been introduced by Vogler. He has studied the curves traced on a torse at a constant distance from its edge of regression. The torse of a space curve in E3 is dual to its pseudo-rectifying torse [11]. Later, Hacısalihoğlu obtained a more general case of Vogler’s results [12].

This subject has been studied in Euclidean 3-space since the 1970s, and it is a method that generates a new curve from the curve through the Frenet frame of the curve. For the first time, we will discuss this issue in 3-dimensional Galilean space. While the curve is produced by using the unit vector which is defined by the Frenet frame apparatus of a curve in Euclidean 3-space, we will have produced the curve by considering two situations in the Galilean 3-space. This is because, in Galilean space, vectors are treated in two ways, isotropic and non-isotropic.

In this paper, we first recall the essential preliminaries on the Galilean 3-space. Then, we define curves in the Galilean 3-space and give the curvature properties of these curves. In the main part of our study, we define a curve noted by rλ at a constant distance from its edge of regression on a unit-speed admissible curve r in the Galilean 3-space. We give relations between the Frenet apparatus and the curvatures of r and rλ. Using these relations, we get some conclusions. Also, we investigate ruled surfaces generated via the curve rλ. In the last section, there are examples, two of which are ruled surfaces.

2  Preliminaries

Let us consider a curve α(t) in 3-dimensional Euclidean space with {T, N, B} as the Frenet frame at the point P=α(s) of α(t). d is described as a vector tightly fastened to Frenet trihedron {T, N, B} such that d=d1T+d2N+d3B, where d1,d2,d3 are constant numbers and d12+d22+d32=1. k is described as a line tightly fastened to Frenet trihedron {T, N, B} in the direction of d and passing through point P (see Fig. 1) [12]. Let Pv denote a point on the line k at a constant distance v from P. During the movement of the Frenet trihedron along the curve α(t), Cv(t) is geometric place of Pv(s) which is defined as a curve at a constant distance from the edge of regression of the curve α (see Fig. 1) [12].

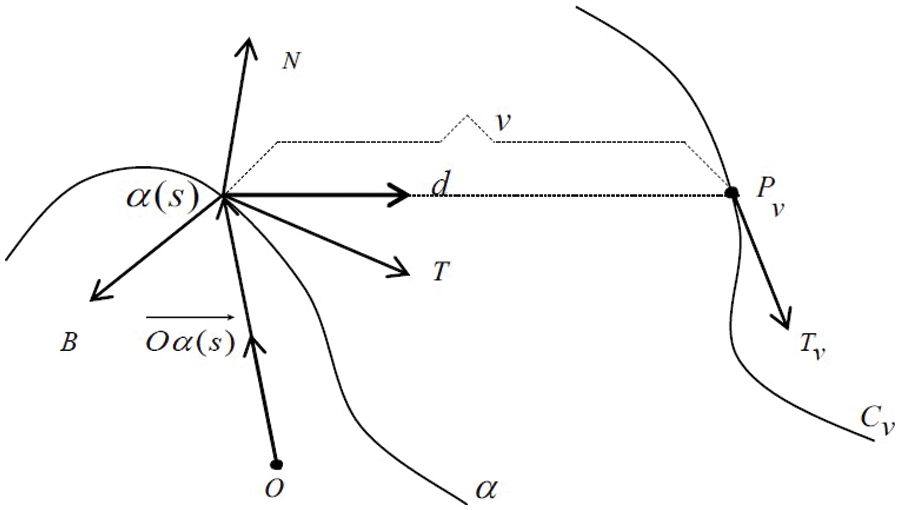
Figure 1: The curve α and the curve Cv

The Galilean space G3 is one of the Cayley-Klein geometries with projective signature (0, 0, +, +) as described in [6]. The absolute of the Galilean geometry is an ordered triple {w, f, I} where w is the ideal (absolute) plane, f is the (absolute) line in w and I is the fixed elliptic involution of points of f. For more detailed information about this space, see [2,3,9,13,14].

Now, let us consider the basic definitions and notions.

Let A→=(x,y,z) and B→=(x1,y1,z1) be two vectors in the Galilean 3-space G3. The Galilean scalar product of two vectors is defined by

⟨A→,B→⟩G=A→.B→={xx1,ifx≠0orx1≠0yy1+zz1,ifx=0andx1=0

If A→.B→=0, these vectors are called perpendicular in the sense of Galilean in G3 [2].

Let A→=(x,y,z) be a vector in the Galilean 3-space. The norm of the vector A→ is defined by [2]

||A→||G={|x|,x≠0y2+z2,x=0

The Galilean vector product of two vectors in G3 is

A→×GB→=|0e2e3xyzx1y1z1|,

where A→=(x,y,z) and B→=(x1,y1,z1)∈G3 [3,15].

A vector A→=(x,y,z)∈G3 is said to be isotropic if x = 0. On the other hand, the vector is defined as non-isotropic vector if x≠0 [15].

Definition 2.1. An angle θ between two unit non-isotropic vectors A→=(1,y,z) and B→=(1,y1,z1) in G3 is described in [16] as

θ=(y1−y)2+(z1−z)2.(1)

If the vectors A→=(1,y,z) and B→=(0,y1,z1) in G3 are taken, an angle θ between the vectors is described as

θ=yy1+zz1y12+z12.(2)

If the vectors A→=(0,y,z) and B→=(0,y1,z1) in G3 are isotropic, the cosine of the angle between two vectors is described as

cos⁡θ=yy1+zz1y2+z2y12+z12.(3)

2.1 Curves in Galilean 3-Space

Let α be a curve given by α:I→G3,α(t)=(x(t),y(t),z(t)) where x(t),y(t),z(t)∈G3. In this case if x′(t)≠0, α(t) is said to be a regular curve.

Let α:I→G3 be a regular curve in G3. Arc length of the curve α is ds=|x′(t)dt|=|dx|. Hence, we obtain s = x. Let α:I→G3 be a curve α(x)=(x,y(x),z(x)) then we say that the curve is parameterized by arc length [4].

In this case, the functions y,z:I→R are said to be coordinate functions of the curve. Here, differentiating α(x)=(x,y(x),z(x)) with respect to x and using the norm definition, we obtain

||α′(x)||G=1.(4)

Then, α(x) is a unit speed curve. Let α:I→G3,α(x)=(x,y(x),z(x)) be a regular unit speed curve in G3. Differentiating α(x), we have

α′(x)=(1,y′(x),z′(x)).(5)

The using Eq. (4), then the tangent vector of α is defined as

T(x)=(1,y′(x),z′(x)).(6)

If we take the derivation of Eq. (5), we get

α′′(x)=(0,y′′(x),z′′(x)).(7)

And from Eqs. (5) and (7), we write α′(x).α′′(x)=0. Here, the normal vector of the curve α is the vector in the direction. Then, the unit normal vector is defined as

N(x)=α′′(x)||α′′(x)||G.(8)

Using Eqs. (7) and (8), we write

N(x)=1y′′2(x)+z′′2(x)(0,y′′(x),z′′(x)).(9)

As a consequence, the unit binormal vector B(x) of α is

B(x)=1y′′2(x)+z′′2(x)(0,−z′′(x),y′′(x)),(10)

and then the frame {T(x),N(x),B(x)} chosen in this way is called the Frenet-Serret frame for unit speed curves in the Galilean 3-space [5].

Proposition 2.1. The Frenet formulae of a unit speed curve α(x) in G3 is given by

(T′(x)N′(x)B′(x))=(0κ(x)000τ(x)0−τ(x)0)(T(x)N(x)B(x)),(11)

where

κ(x)=y′′2(x)+z′′2(x)(12)

is the curvature of α and

τ(x)=det(α′(x),α′′(x),α′′′(x))κ2(x)(13)

is the torsion of α [17].

3  Curve at a Constant Distance from the Edge of Regression on a Curve in Galilean 3-Space

Definition 3.1. Suppose that r is a curve in Galilean 3-space and {T, N, B} is the Frenet frame at the point P = r(s) of r. Let Pλ be a point at a constant distance λ from P. During the movement of the Frenet trihedron along the curve r, rλ=r+λd is geometric place of Pλ, where

d={d1T,if d is non-isotropicd2N+d3B, ifdis isotropic

such that d12+d22+d32=1, d1,d2,d3∈R and d2=1, |d|=1. In this case, rλ is called curve at a constant distance from the edge of regression of r.

Now, let us construct the Frenet frame of rλ generated by both the non-isotropic vector d and isotropic vector d and examine its curvature properties.

Case 3.1. d is non-isotropic. In this case, d12=1. Let us d1=1,d2=d3=0. Then, we obtain

rλ(s)=r(s)+λT(s),(14)

where d(s) = T(s).

Theorem 3.1. If r(s) is a curve with arc length parameter s, then the arc length parameter of the curve rλ is also s.

Proof. By differentiating Eq. (14)

ddsrλ(s)=r′(s)+λT′(s).(15)

If we take the norm of two sides of Eq. (15), we have

||ddsrλ(s)||G=||r′(s)+λT′(s)||G=1

which completes the proof.

Theorem 3.2. Let (r(s),rλ(s)) be given the curves pair with arc length s in G3. If the Frenet vectors of r and rλ are {T, N, B} and {Tλ,Nλ,Bλ}, the curvatures are κ, τ and κλ, τλ, respectively, then the following relations hold:

Tλ=T+λκN,(16)

Nλ=1κλ[(κ+λκ′)N+λκτB],(17)

Bλ=1κλ[−λκτN+(κ+λκ′)B],(18)

κλ=κ2+2λκκ′+λ2(κ′2+κ2τ2)(19)

and

τλ=κλ2τ+λκ2τ′+λ2(κ′2τ+κκ′τ′−κκ′′τ)κλ2.(20)

Proof. By differentiating Eq. (14) and using Eq. (11), we have

rλ′=T+λκN(21)

which gives us Eq. (16). If we take derivation of Eq. (21) according to s, we get

rλ′′=(κ+λκ′)N+λκτB.(22)

Using Eq. (22) in Eq. (12), then we get Eq. (19). From Eq. (9), we easily obtain the Eq. (17) and from Eq. (10), we get Eq. (18). Considering Eq. (13), we obtain Eq. (20).

In these calculations we used rλ′′′=(κ′+λκ′′−λκτ2)N+(κτ+2λκ′τ+λκτ′)B

Corollary 3.1. Let (r(s),rλ(s)) be the curves pair given with arc length s in G3. If the curve r is a circular helix, rλ is also a circular helix.

Proof. We know that if the curvatures κ and τ of r are constants, τκ is also constant and then r is a circular helix. If we take κ and τ as constants in Eqs. (19) and (20), we get κλ=κ1+λ2τ2 and τλ=τ.

In this case, we have τλκλ=τκ1+λ2τ2. τλκλ is fixed since λ,κ and τ are constants. Then, rλ is also a circular helix.

Case 3.2. d is isotropic. In this case, d22+d32=1 and d=d2N+d3B. Hence, we have

rλ=r(s)+λ2N+λ3B,(23)

where λ2=λd2 and λ3=λd3.

Theorem 3.3. If r(s) is a curve with arc length parameter s, then the arc length parameter of the curve rλ is also s.

Proof. By differentiating Eq. (23), we have

ddsrλ(s)=r′(s)+λ2N′(s)+λ3B′(s).(24)

If we take the norm of two sides of Eq. (24), we have ||ddsrλ(s)||G=||r′(s)+λ2N′(s)+λ3B′(s)||G=1 which completes the proof.

Theorem 3.4. Let (r(s),rλ(s)) be the curves pair given with arc length s in G3. If the Frenet vectors of r and rλ are {T, N, B} and {Tλ,Nλ,Bλ}, the curvatures are κ, τ and κλ, τλ, respectively, then the following relations hold:

Tλ=T−λ3τN+λ2τB,(25)

Nλ=1κλ[(κ−λ2τ2−λ3τ′)N+(λ2τ′−λ3τ2)B],(26)

Bλ=1κλ[(−λ2τ′+λ3τ2)N+(κ−λ2τ2−λ3τ′)B],(27)

κλ=κ2+(λ22+λ32)(τ4+τ′2)−2κ(λ2τ2+λ3τ′),(28)

and

τλ=κλ2τ+(λ22+λ32)(2ττ′2−τ2τ′′)+λ2(κτ′′−κ′τ′)+λ3(κ′τ2−2κττ′)κλ2.(29)

Proof. By differentiating Eq. (23) and using Eq. (11), we obtain rλ′=T−λ3τN+λ2τB which gives Eq. (25). If we take second derivation of rλ according to s and use Eq. (11) again, we get rλ′′=(κ−λ2τ2−λ3τ′)N+(λ2τ′−λ3τ2)B. In the light of this last equation, if we take into account Eq. (12) we have Eq. (28). From Eqs. (8) and (26) is obtained and Eq. (27) is found as a consequence of Eq. (10). Considering (13), the torsion of rλ is found as in Eq. (29).

In these calculations, we use rλ′′′=(κ′−λ3τ′′−3λ2ττ′+λ3τ3)N+(κτ−λ2τ3−3λ3ττ′+λ2τ′′)B.

Corollary 3.2. Let (r(s),rλ(s)) be the curve pair given with arc length s in G3. If the curve r is circular helix, rλ is also a circular helix.

Proof. If the curvatures of r are constants, τκ is constant and r is a circular helix. Considering κ and τ as constants in Eqs. (28) and (29), the curvatures of rλ are κλ=κ2+(λ22+λ32)τ4−2κλ2τ2 and τλ=τ, respectively.

In this case, we have τλκλ=τκ2+(λ22+λ32)τ4−2κλ2τ2. Here, τλκλ is fixed since λ2,λ3,κ and τ are constants. Then, rλ is also a circular helix.

4  Ruled Surfaces Generated by the Curve rλ

The ruled surfaces in G3 are three types. Definitions of the ruled surfaces of type A, B, C and current studies can be viewed in [6,15,17–20]. Our goal is to define the ruled surfaces using rλ as the base curve and rλ′ as the director curve, and to see if they can be developed. Here, we take into account ruled surfaces of type A.

4.1 Ruled Surface of Type A Generated by rλ(s)=r(s)+λT(s)

A ruled surface of type A in G3 by using non-isotropic vector D can be written as

XA(s,v)=rλ(s)+vD(s),(30)

where rλ is defined as in Eq. (14) and the vector D is tangent of rλ. Besides, the curve rλ defined a directrix that does not lie in Euclidean plane and non-isotropic vector D(s)=Tλ is generator. The associated orthonormal triple of XA(s,v) is given by

t(s)=Tλ(s),n(s)=Nλ(s),b(s)=Bλ(s),(31)

where Frenet trihedron {Tλ,Nλ,Bλ} is the Frenet frame of the unit speed curve rλ(s) in Galilean 3-space. From Eq. (31), we see that two orthonormal triple coincide.

Additionally, the parameter of distribution PXA of XA(s,v) is

PXA=−det(rλ′,D,D′)||D′||G2.(32)

We know that if PXA=0, XA(s,v) is developable. Then, we can express the following theorem:

Theorem 4.1. Suppose that (r,rλ) is a unit speed curves pair in Galilean 3-space with rλ=r+λT, where {T, N, B} and {Tλ,Nλ,Bλ} are the Frenet frame of r and rλ, respectively. D is a non-isotropic vector tightly fastened to Frenet trihedron {Tλ,Nλ,Bλ} of rλ at the origin and XA(s,v) is the ruled surface of type A generated by D and rλ. Then, XA(s,v) is a developable surface.

Proof. By taking derivative of rλ with respect to s and by using Theorem 3.2, we obtain

rλ′=Tλ=T+λκN.(33)

If we take D=Tλ, we get PXA=0 from Eq. (32). Thus, XA(s,v) is developable.

Now, we consider that v is a constant in the ruled surface XA(s,v). Then, XA(s,v)=rλ(s)+vD(s) is the equation of parametric curve rλv for the points Kv on the ruled surface. In this case, we have

Tλv=Tλ+vκλNλ,(34)

where Tλv is tangent vector at a point Kv of rλv for v −constant. If κλ is a non-zero constant, we deduce from Eq. (34) that rλ is a Bertrand curve.

Finally considering Eq. (1), the angle θ between non-isotropic vectors Tλv and D, we calculate as θ=vκλ.

4.2 Ruled Surface of Type A Generated by rλ=r(s)+λ2N+λ3B

Similarly to Section 4.1, a ruled surface of type A in G3 by using D=Tλ can be written as

XA(s,v)=rλ(s)+vD(s),(35)

where the curve rλ(s)=r(s)+λ2N+λ3B is directrix and D(s)=Tλ=T−λ3τN+λ2τB is generator. In this case, the associated orthonormal triple of XA(s,v) is found as Eq. (31).

Thus, the following theorem can be written:

Theorem 4.2. Suppose that (r,rλ) is a unit speed curves pair in Galilean 3-space with rλ=r+λ2N+λ3B, where {T, N, B} and {Tλ,Nλ,Bλ} are Frenet frame of r and rλ, respectively. D is a non-isotropic vector tightly fastened to Frenet trihedron {Tλ,Nλ,Bλ} of rλ at the origin and XA(s,v) is the ruled surface of type A generated by D and rλ. Then, XA(s,v) is a developable surface.

Proof. By taking derivative of rλ with respect to s and by using Theorem 3.4, we have

rλ′=Tλ=T−λ3τN+λ2τB.(36)

We take as D=Tλ, so by a straightforward computation, the parameter of distribution for XA(s,v) is calculated as follows:

PXA=0.(37)

Hence, XA(s,v) is developable.

5  Applications

Example 5.1. Consider the curve given by the parametrization

ϕ(σ)=(σ,−σcos⁡(σ)+2sin⁡(σ),−σsin⁡(σ)−2cos⁡(σ)).(38)

The Frenet frame fields of the curve of ϕ(σ) are [Fig. 2]

T(σ)=(1,σsin⁡(σ)+cos⁡(σ),−σcos⁡(σ)+sin⁡(σ)),N(σ)=(0,cos⁡(σ),sin⁡(σ)),B(σ)=(0,−sin⁡(σ),cos⁡(σ)).

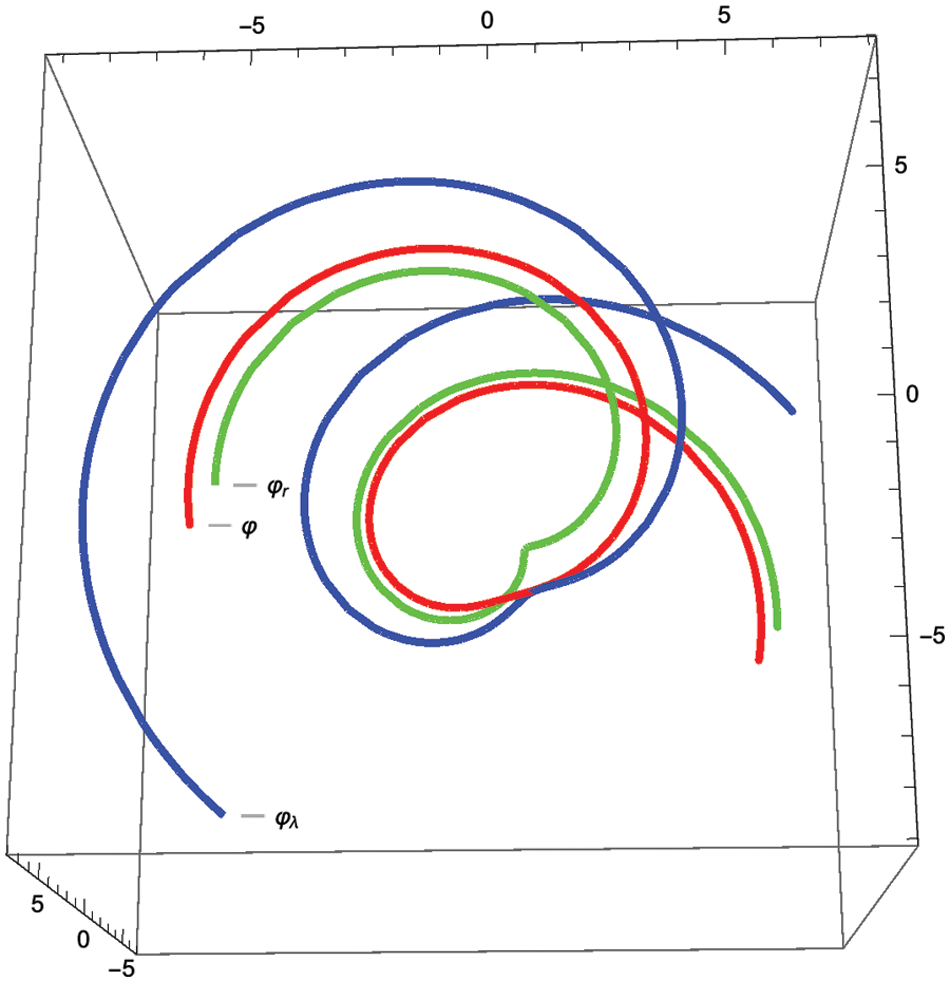
Figure 2: The red curve is ϕ(σ), the blue curve is ϕλ (σ) and the green curve is ϕr(σ)

Considering Eq. (14), the curve ϕλ(σ) generated by non-isotropic vector d in G3 is

ϕλ(σ)=ϕ(σ)+λT,

ϕλ(σ)=(1+σ,(1−σ)cos⁡(σ)+(2+σ)sin⁡(σ),(1−σ)sin⁡(σ)−(2+σ)cos⁡(σ))

for λ=1 (see Fig. 2). If d is isotropic vector, from Eq. (23) we have

ϕr(σ)=(σ,−σcos⁡(σ)+(4−32)sin⁡(σ)+12cos⁡(σ),−σsin⁡(σ)+(−4+32)cos⁡(σ)+12sin⁡(σ))

for r=1,d2=12,d3=32 (see Fig. 2).

Example 5.2. Let us consider the curve given by Eq. (38) in Example 5.1. The ruled surface XA(σ,v) obtained by using Eq. (38) in light Eq. (30) is

XA(σ,v)=(1+v+σ,(1−σ+v(1+λκ))cos⁡(σ)+(2+σ(1+v))sin⁡(σ),(1−σ+v(1+λκ))sin⁡(σ)−(2+σ(1−v))cos⁡(σ)).(39)

In Eq. (39), the curve ϕλ(σ)=(1+σ,(1−σ)cos⁡(σ)+(2+σ)sin⁡(σ),(1−σ)sin⁡(σ)−(2+σ)cos⁡(σ)) is directrix and the non-isotropic vector

D(σ)=Tλ(s)=(1,σsin⁡(σ)+(1+λκ)cos⁡(σ),−σcos⁡(σ)+(1+λκ)sin⁡(σ))

is generator. For κ=σ and λ=1, Eq. (39) is shown in the Fig. 3.

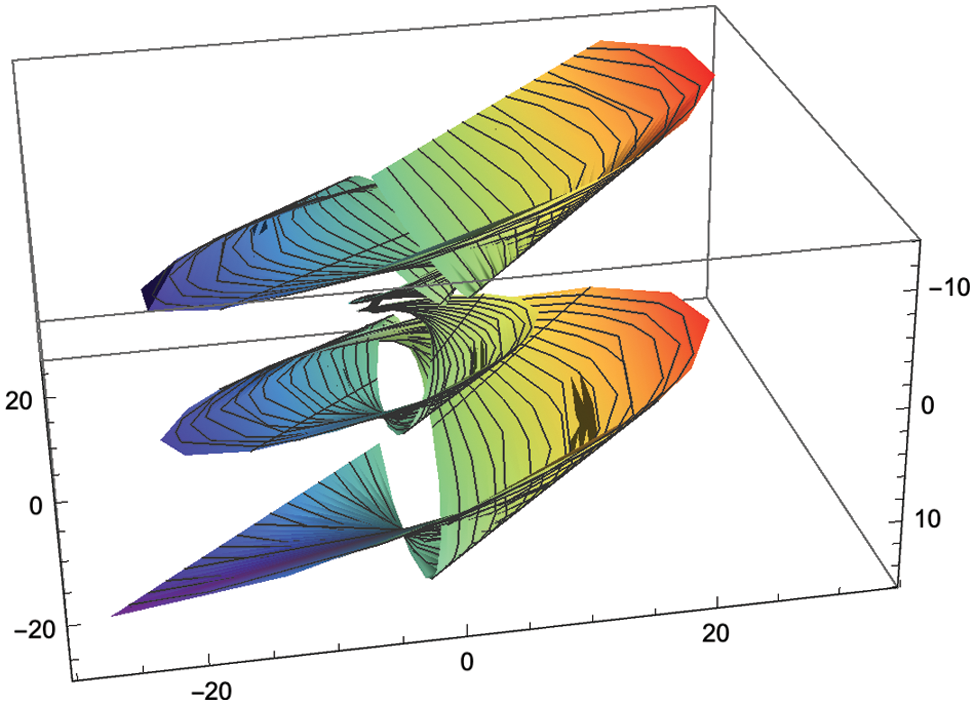
Figure 3: The surface XA(σ, v), for −π ≤ σ ≤ π, −1 ≤ v ≤ 1

Example 5.3. Let us consider the curve given by Eq. (38) in Example 5.1. The ruled surface XA(σ,v) obtained by using Eq. (38) in light Eq. (35) is

XA(σ,v)=(σ+v,(−σ+12+v(1−λ3τ))cos⁡(σ)+(4−32+v(σ−λ2τ))sin⁡(σ),(−σ+12+v(1−λ3τ)sin⁡(σ)+(−4+32+v(−σ+λ2τ))cos⁡(σ))).(40)

In Eq. (40), the curve

ϕr(σ)=(σ,−σcos⁡(σ)+(4−32)sin⁡(σ)+12cos⁡(σ),−σsin⁡(σ)+(−4+32)cos⁡(σ)+12sin⁡(σ))

is directrix and the non-isotropic vector

Tλ(s)=(1,(σ−λ2τ)sin⁡(σ)+(1−λ3τ)cos⁡(σ),(1−λ3τ)sin⁡(σ)+(−σ+λ2τ)cos⁡(σ))

is generator. For τ=1 and λ2=λ3=1, Eq. (40) is shown in the Fig. 4.

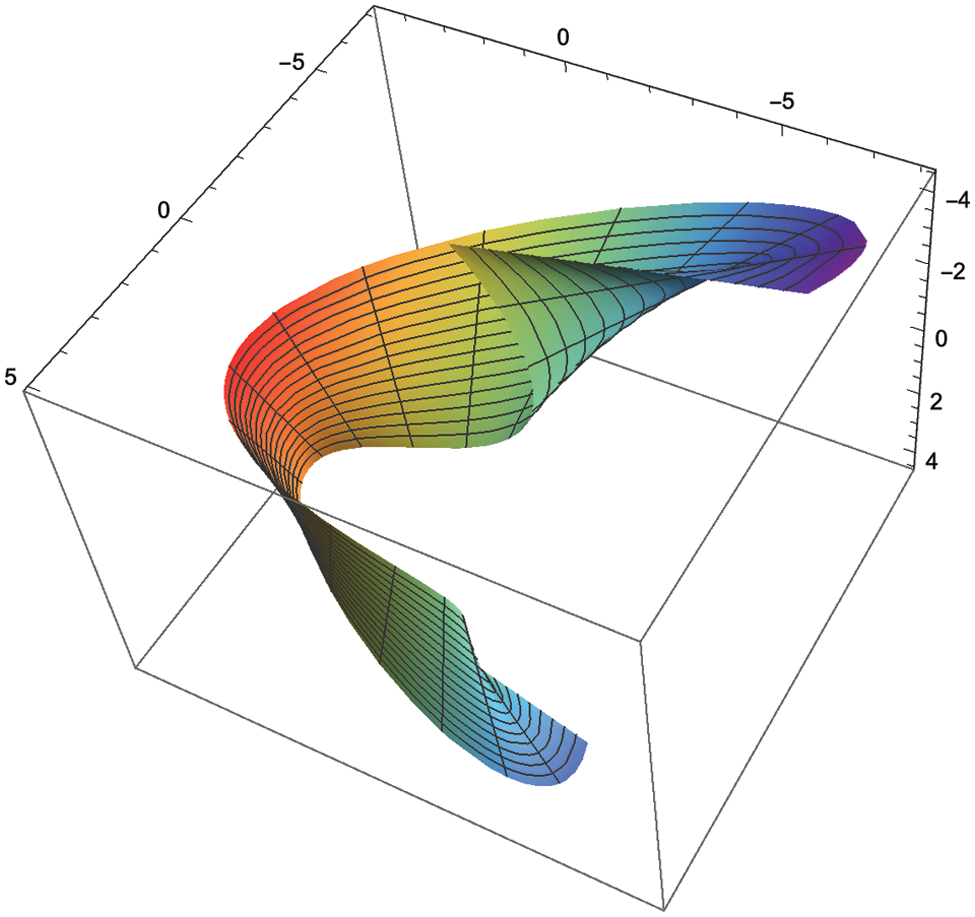
Figure 4: The surface XA(σ, v), for −π ≤ σ ≤ π, −1 ≤ v ≤ 1

6  Conclusion

In this study, we present a method that generates a new curve from the curve using the Frenet frame of a curve that is parameterized by arc length in G3. We show that it is possible in two ways to achieve this state in Galilean 3-space. We also calculate the Frenet frame and curvatures of the generated curve in terms of the Frenet frame and curvatures of the first curve. In this case, we reveal that if the first curve is helix, the generated curve is also the helix curve. In Section 4, for Case 3.1 and Case 3.2, ruled surfaces generated by the constructed curve and its tangents are discussed. It has been shown that the composed ruled surfaces are developable.

Acknowledgement: The authors wish to express their appreciation to the reviewers for their helpful suggestions which greatly improved the presentation of this paper.

Funding Statement: The authors received no specific funding for this study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

References

 1.  Klein, F. (1893). Vergleichende betrachtungen uber neuere geometrische forschungen. Mathematische Annalent, 43(1), 63–100. [Google Scholar]

 2.  Yaglom, I. (1979). A simple non-euclidean geometry and physical basis. New York, USA: Springer. [Google Scholar]

 3.  Röschel, O. M. (1984). Die geometrie des galileischen raumes (Ph.D. Thesis). Habilitationsschrift, Leoben. [Google Scholar]

 4.  Divjak, B. (1998). Curves in pseudo-galilean geometry. Annales Universitatis Scientiarum Budapestinensis de Rolando Eotvos Nominatae, 41, 117–128. [Google Scholar]

 5.  Pavkovic, B. J., Kamenarovic, I. (1987). The equiform differential geometry of curves in the galilean space G3. Glasnik Matematicki, 22(42), 449–457. [Google Scholar]

 6.  Divjak, B., Sipus, Z. M. (2003). Special curves on ruled surfaces in galilean and pseudo-galilean spaces. Acta Mathematica Hungarica, 98(3), 203–215. [Google Scholar]

 7.  Öğrenmiş, A. O., Ergut, M., Bektas, M. (2007). On the helices in the galilean space G3. Iranian Journal of Science and Technology, Transaction A, 31(2), 177–181. [Google Scholar]

 8.  Nešović, E., Öztürk, U., Öztürk, E., Koç, B. (2018). On T-slant, N-slant and B-slant helices in galilean space G3. Journal of Dynamical Systems and Geometric Theories, 16(2), 187–199. [Google Scholar]

 9.  Öğrenmiş, A. O., Öztekin, H., Ergüt, M. (2009). Bertrand curves in galilean space and their characterizations. Kragujevac Journal of Mathematics, 32(32), 139–147. [Google Scholar]

10. Yüzbaşı, Z. K., Yoon, D. W. (2020). On geometry of isophote curves in galilean space. AIMS Mathematics, 6(1), 66–76. [Google Scholar]

11. Vogler, H. (1963). Über die pseudorektifizierenden torsen von raumkurven. Springer in Komm, 171, 173–178. [Google Scholar]

12. Hacısalihoğlu, H. H. (1968). On the motion of the frenet thrihedron of a space curve, 17, 33–55. Communications Faculty of Sciences University of Ankara Series A1 Mathematics and Statistics. http://hdl.handle.net/20.500.12575/60677. [Google Scholar]

13. Dede, M., Ekici, C., Çöken, A. C. (2013). On the parallel surfaces in galilean space. Hacettepe Journal of Mathematics and Statistics, 42(6), 605–615. [Google Scholar]

14. Sipus, Z. M., Divjak, B. (2011). Translation surfaces in the galilean space. Glasnik Matematicki, 46(2), 455–469. [Google Scholar]

15. Sipus, Z. M. (2008). Ruled weingarten surfaces in galilean space. Periodica Mathematica Hungarica, 56(2), 213–225. [Google Scholar]

16. Artykbaev, A. (1988). Total angle about the vertex of a cone in galilean space. Mathematical Notes of the Academy of Sciences of the USSR, 43(5), 379–382. [Google Scholar]

17. Kamenarovic, I. (1991). Existence theorems for ruled surfaces in the galilean space G3. Rad Hrvatske Akademije Znanosti i Umjetnosti, Matematicke Znanosti, 456(10), 183–196. [Google Scholar]

18. Divjak, B., Sipus, Z. M. (2004). Transversal surfaces of ruled surfaces in pseudo galilean space. Sitzungsberichte und Anzeiger Abteilung II, 213, 23–32. [Google Scholar]

19. Dede, M., Ekici, C. (2016). On parallel ruled surfaces in galilean space. Kragujevac Journal of Mathematics, 40(1), 47–59. [Google Scholar]

20. Gür Mazlum, S., Şenyurt, S., Grilli, L. (2022). The dual expression of parallel equidistant ruled surfaces in Euclidean 3-space. Symmetry, 14(5), 1062. [Google Scholar]