Average Secrecy Capacity of the Reconfigurable Intelligent Surface-Assisted Integrated Satellite Unmanned Aerial Vehicle Relay Networks

1  Introduction

The future requirements of wireless communication networks have so many characters, such as wider coverage, higher energy utilization, et al. Wider coverage has become an important requirement for the wireless networks [1]. Based on this consideration, satellite communication (SatCom) comes to our insight for its ubiquitous coverage and seamless services for several users [2]. Thus, by both using the superiorities of the SatCom and terrestrial communication networks (TCNs), integrated satellite terrestrial networks (ISTNs) arise, which can not only use the advantage of the SatCom but also utilize TCNs. They are considered as the future structure of the next generation wireless communication system [3–5]. ISTNs have been considered as the important part in the practical systems, such as Digital Video Broadcasting (DVB) networks and the Space-Ground Integrated Information Network Engineering of China [6–9].

1.1 Related Works

As mentioned previously, the ISTN has been regarded as a hot research topic these years. In [10], the authors researched the outage probability (OP) for a representative ISTN along with several terrestrial relays by applying the threshold-assisted selection algorithm. The authors in [11] researched the OP for a representative uplink ISTN with the opportunistic terrestrial selection algorithm. In [12], the authors studied the OP for ISTNs with the proposed maximal-maximum terrestrial selection scheme. The authors in [13] studied the symbol error rate for an ISTN. As known, the SatCom has a wider coverage, which results into the appearance of the secrecy problem. By utilizing the different method, physical layer security (PLS) mainly researches the divergence between the legitimate user’s channel and the eavesdropper’s channel [14,15]. The authors in [15] summarized all the secrecy problems in the SatCom and gave some promising secrecy researching directions. In [16], the authors researched the security problem for a cognitive ISTN by applying beamforming (BF) scheme. The authors in [17] proposed a threshold-based legitimate users scheduling algorithm for an ISTN with several legitimate users and several eavesdroppers. In [18], the authors obtained the detailed investigations for the secrecy outage probability (SOP) of the ISTNs along with a two-way unmanned-aerial-vehicle (UAV). In [19], the authors studied the SOP for an ISTN with non-orthogonal multiple access (NOMA) technique by also considering the cognitive technology. In [20], a cooperative relay selection and legitimate user selection algorithm was proposed and the average secrecy capacity (ASC) was investigated in a considered ISTN. The authors in [21] proposed a BF algorithm to optimize the secrecy performance of an ISTN. In [22], the authors proposed an alternating optimization scheme to address the SEE problem by decomposing the original nonconvex problem into subproblems.

1.2 Motivations

Another important demand for the 6G network and next wireless communication network is higher energy efficiency, which has been an important factor for the urban networks [23]. On this foundation, reconfigurable intelligent surface (RIS) technology comes to our sight. The RIS is the man-made surface which can reflect the signal by setting the suitable phase with the special electronic material [24]. It will need nearly no power to reflect the legitimate signal, which is now the famous topic in recent five years [25,26]. The authors in [27] researched the OP of the UAV-assisted communication networks. In [28], the authors minimized the transmission power for the representative UAV-based RIS-assisted hetnets. The authors in [29] studied the effect of RIS and UAV relay on the ISTNs. In [30], the authors researched the impacts of hardware impairments and RIS on the considered ISTNs in the presence of a UAV relay. Besides, the insightful investigation for the OP was studied. In [31], the authors investigated the joint beamforming problems for a representative ISTN. In [32], the authors studied the impact of NOMA scheme on a representative ISTN in the presence of cognitive technology.

By summarizing all and trying the authors’ best efforts, the study for the effect of RIS-based ISTN with a UAV on the ASC remains unreported, which motivates our work.

1.3 Our Contributions

Accordingly, this paper first considers the UAV relay and an eavesdropper. Specifically, the RIS is mounted on a tall building to augment the transmission. Then, we research the ASC for the considered network. The detailed works of this paper are given in what follows as

•   By taking the satellite, the UAV, the legitimate user and an eavesdropper into consideration, the considered secrecy network appears. Furthermore, in order to enhance the secrecy transmission and improve the energy utilization, the RIS is stalled in the high building to help the UAV. In addition, the decode-and-forward (DF) forwarding method is used at the UAV to assist the satellite’ transmission. For some practical reasons, the direct transmission link does not exist in the considered network, namely, the satellite can not communicate with the legitimate user directly.

•   Relied on the considered networks, detailed investigations for the ASC are obtained. The detailed investigations provide efficient methods to evaluate the effects of key parameters, i.e., the channel and system parameters on the ASC. Especially, these derivations can derive the impacts of RIS’ parameters.

•   To gain further investigations of the ASC on the considered networks, the asymptotic investigations for the ASC are gotten, which give deep insights on the considered secrecy networks.

The structure of this paper is as follows. Through Section 2, the detailed illustration of regarded secrecy system model is provided. In Section 3, the deep investigations for the ASC are provided along with the closed-form expressions. In Section 4, the asymptotic analysis is further obtained. In Section 5, some representative Monte Carlo simulations are given to see the rightness of the analytical investigations. The summary of this work is given in Section 6.

Notations |⋅| depicts the absolute value of a complex scalar. E[⋅] represents the expectation function, 𝒞𝒩(a,B) depicts the complex Gaussian distribution, which consists of a random vector a and covariance matrix B. Fy(⋅) and fy(⋅) represent the cumulative distribution function (CDF) and probability density function (PDF) the of random variable y, respectively. The abbreviations are given in Table 1.

2  System Model

As shown in Fig. 1, through this secrecy RIS-assisted ISTN, it includes a satellite S, a legitimate user D and a DF UAV1 relay R. Besides, a RIS is equipped in a high structure to forward the signal. In this considered network, one antenna is considered for the whole network nodes2. As the former presentation, the satellite and UAV both own a wider beam coverage, thus an eavesdropper exists in the considered model and wants to steal the information signal from the UAV. Owing to the shadowing and so many problems, not any direct transmitting link is available in this paper, namely, D cannot receive the information from S and cannot receive the signal from R directly3.

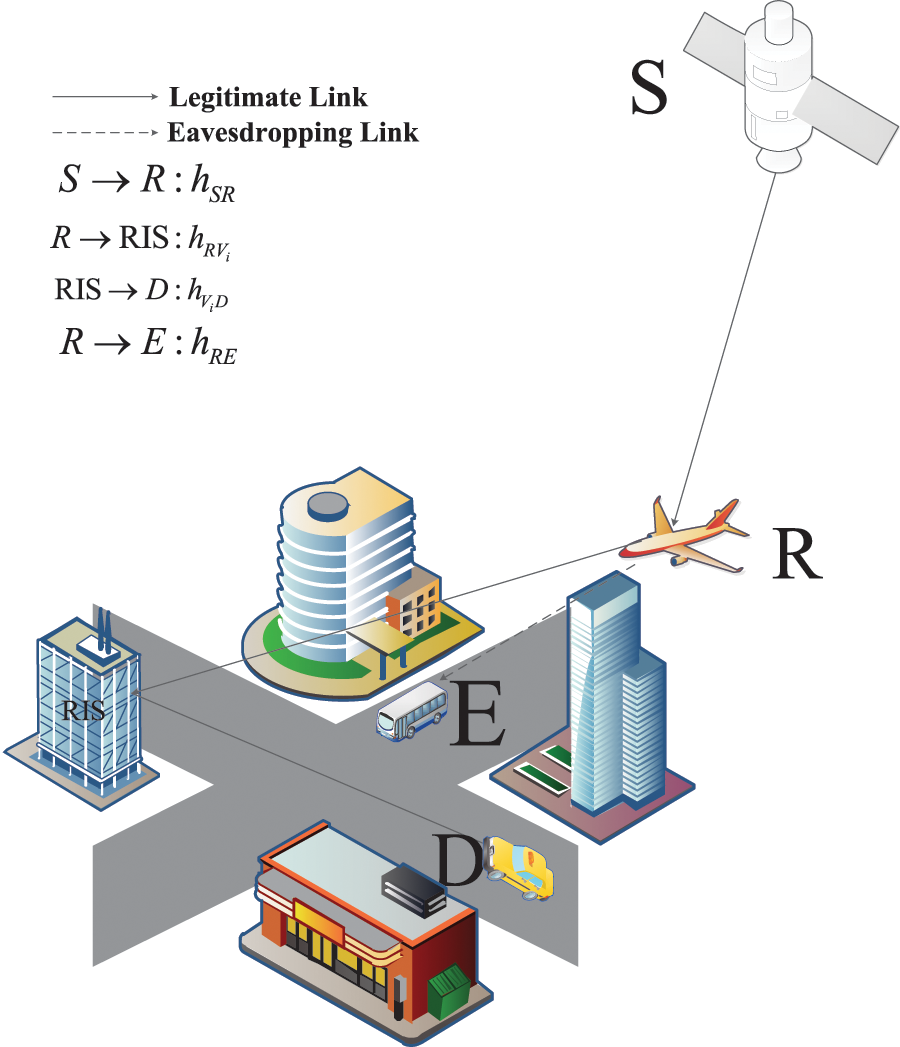
Figure 1: The system model illustration

Two time slots will last for the whole link. In the first one, S forwards the symbol s(t) with E[|s(t)|2]=1 to R, thus the obtained signal at R can be regarded as

ySR(t)=PShSRs(t)+nR(t),(1)

where PS depicts the transmitting energy of S, hSR represents the channel fading between the S and R which suffers from shadowed-Rician (SR), n(t) depicts the additive white Gaussian noise (AWGN) at R which has the model as nR(t)∼𝒞𝒩(0,δR2).

For the second one, R intends to transmit the legitimate information signal to D. Unfortunately, due to some reasons, such as obstacles, R cannot transmit the singal with D directly. So, RIS is utilized to assist the legitimate transmitting. Then, the final signal at D is represented with the expression as

yRD(t)=PR[∑i=1NhRXiejθihXiD]s(t)+nD(t),(2)

where PR depicts the transmitting power from R, N is the number of the reflecting factor, θi depicts the phase shift of the i-th factor for the RIS. hRXi and hXiD represent the channel function with presentation as hRXi=βie−ϕi/LRXi and hXiD=τie−ψi/LXiD, respectively. βi and τi denote the random variable which undergoes Rayleigh fading with a π/2 mean and a (4−π)/4 variance. LRXi=10log⁡10(lRXiφ)+B and LXiD=10log⁡10(lXiDφ)+B represent the path loss, B denotes the a target value which has a relationship with the transmitting frequency and some other issues. lXiD and lRXi denote the distance of the RIS-D and R-RIS links, respectively, φ represents the path loss value. nD(t) denotes the AWGN at D shadowed as nD(t)∼𝒞𝒩(0,δD2) [30].

By utilizing [33], the best performance signal for the RIS → D link can be obtained by setting θi=ϕi+ψi, which can be shown as

yRD(t)=PR(∑i=1Nβiτi⏟χ/LRXiLXiD)s(t)+nD(t).(3)

As mentioned before, a secrecy problem exists in the considered networks. Thus, the information overheard by the Eve is shown as

yRE(t)=PRhREs(t)+nE(t),(4)

where hRE represents the channel shadowing between R and E with modeling as Rayleigh fading, nE(t) represents the AWGN at E obeyed as nE(t)∼𝒞𝒩(0,δE2).

From (1), (3) and (4), the obtained signal-to-noise ratios (SNRs) for the different links are respectively given by

γSR=PS|hSR|2δR2,(5)

γRD=PRχ2LRXiLXiDδD2,(6)

γE=PR|hRE|2δE2.(7)

For the reason that DF protocol is applied for the UAV, then the legitimate SNR of the secrecy system is obtained as

γB=min(γSR,γRD).(8)

Relied on [14], the secrecy capacity has the following definition as

CS=⌊CB−CE⌋+,(9)

where ⌊x⌋+=Δ⁡max{x,0}, CB=log2(1+γB) and CE=log2(1+γE).

3  Average Secrecy Capacity

From [17], the ASC is regarded as

C¯S=∫0∞∫0∞CSfγB(x)fγE(z)dxdz=∫0∞∫0∞[log2(1+x)−log2(1+z)]fγB(x)fγE(z)dxdz=1ln⁡2∫0∞FγE(z)1+z[1−FγB(z)]dz.(10)

Before getting the final derivations of ASC, the first important one is to gain the CDF and PDF for γSR, γRD and γE, respectively.

3.1 Preliminaries

3.1.1 The Satellite Transmission Model

Through this paper, the geosynchronous earth orbit (GEO)4 satellite is assumed. In addition, the satellite is considered to own many transmission beams. Moreover, time division multiple access (TDMA)5 algorithm is inserted which leads to result that just one UAV is used in each time slot.

Then, hSR is expressed as

hSR=CSRfSR,(11)

where fSR depicts the SR fading of the satellite shaodwing, and CSR depicts the impact of antenna pattern and the free space loss (FSL), which can be represented as

CSR=λGSRGR4πd2+d02,(12)

where λ represents the frequency carrier’s wavelength, d is the length from terrestrial relay to the satellite beam’s center with d=d0tan⁡(θ¯k). θ¯k is regarded as the 3 dB angle. d0≈35786km is the antenna gain for terrestrial relay. Furthermore, GR depicts the beam gain of the considered satellite.

From [17], GR can be approximated as

GR(dB)≃{G¯max,for 0∘<ϑ<1∘32−25log⁡ϑ,for 1∘<ϑ<48∘−10,for 48∘<ϑ≤180∘,(13)

where G¯max represents the maximum beam gain, and ϑ denotes the off-boresight’s angle. Considering GSR as the gain of the antenna, by assuming θk being the angle, which is shown as [2,19]

GSR≃Gmax(K1(uk)2uk+36K3(uk)uk3),(14)

where Gmax depicts the best beam gain, uk=2.07123sin⁡θk/sin⁡θ¯k, K3 and K1 are the order 3 and 1 for the 1st-kind bessel function, respectively. To derive the best performance, θk→0 is applied which leads to GSR≈Gmax. After which consideration, we get hSR=CSRmaxfSR.

For fSR, a popular SR model was mentioned in [34] that suits for land mobile satellite (LMS) networks [17]. By using [2], fV could be re-given as fSR=f¯SR+f~SR, where f~SR has the assumption as independent identically distributed (i.i.d) Rayleigh shadowing, f¯SR represents the effect of line-of-sight (LoS) issue which undergoes i.i.d Nakagami-m fading.

By utilizing [34], the PDF and CDF of γSR=γ¯SR|CSRmaxfSR| are respectively obtained as

fγSR(x)=∑k1=0mSR−1αSR(1−mSR)k1(−δSR)k1xk1(k1!)2γ¯SRk1+1exp⁡(ΔSRx),(15)

FγSR(x)=1−∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1(−δSR)k1xtk1!t!γ¯SRk1+1ΔSRk1−t+1exp⁡(ΔSRx).(16)

where γ¯SR depicts the average SNR between S and R, ΔSR=βSR−δSRγ¯SR, αSR=(2bSRmSR2bSRmSR+ΩSR)mSR/2bSR, βSR=1/2bSR, δSR=ΩSR(2bSRmSR+ΩSR)2bSR, where mSR≥0 denotes the fading factor, 2bSR represents the multipath’s power. In addition, ΩSR denotes the LoS link’s power. As a common consideration, mSR is assumed to be an integer [18]. (⋅)k1 represents the Pochhammer symbol [35].

3.1.2 The RIS Channel Model

Then, by utilizing [30], the PDF and CDF for γRD are respectively presented as

fγRD(x)≃e−(x−γ¯RDs)22δ2γ¯RD22πδ2γ¯RD2,(17)

FγRD(x)=12πδ2γ¯RD2[Φ(A,B,C,x)−Φ(A,B,C,0)],(18)

where

Φ(A,B,C,x)=12π/Aexp⁡(B2−ACA)erf(Ax+B/A),(19)

and s=Nπ/4 and δ2=N(1−π2/16), A=12δ2γ¯RD2, B=−s2δ2γ¯RD and C=s22δ2, and erf(x) denotes the error function shown in [35]. γ¯RD depicts the average SNR from R to D.

From [35], erf(x) can be obtained as

erf(x)=2π∑k=1∞x2k−1(−1)k+1(k−1)!(2k−1).(20)

3.1.3 The Terrestrial Channel Model

By utilizing the similar method, from [10], the PDF for γE has the expression as

FγE(x)=1−exp⁡(−x/γ¯E).(21)

3.2 The ASC

Recalling (10), the first thing that needs to be done is to get the CDF of γE and the CDF of γB. The CDF of γE has been derived in (21), by utilizing the DF protocol and [33], the CDF for γB can be derived as

FγB(x)=1−Θ1−HΘ1+Θ2,(22)

with

H=12πAδ2γ¯RD2exp⁡(B2−ACA)∑k=1∞(−1)k+1B2k−1Ak−1/2(k−1)!(2k−1),(23)

Θ1=∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1(−δSR)k1xtk1!t!γ¯SRk1+1ΔSRk1−t+1exp⁡(ΔSRx),(24)

and

Θ2=∑k1=0mSR−1∑t=0k1∑k=1∞∑p2k−1(2k−1p)B2k−1−p(−1)k+1xt+pαSR(1−mSR)k1(−δSR)k1exp⁡(B2−ACA)Ak−1/2−p(k−1)!(2k−1)2πaδ2γ¯RD2k1!t!γ¯SRk1+1ΔSRk1−t+1exp⁡(ΔSRx).(25)

By submitting (22) and (21) into (10), (10) can be re-written as

CS=1ln⁡2∫0∞1−exp⁡(−z/γ¯E)1+z(Θ1+HΘ1−Θ2)dz=1ln⁡2∫0∞(1+H)Θ1−Θ21+zdz−1ln⁡2∫0∞exp⁡(−z/γ¯E)(1+H)Θ1−exp⁡(−z/γ¯E)Θ21+zdz=1ln⁡2∫0∞(1+H)Θ11+zdz−1ln⁡2∫0∞Θ21+zdz−1ln⁡2∫0∞exp⁡(−z/γ¯E)(1+H)Θ11+zdz+1ln⁡2∫0∞exp⁡(−z/γ¯E)Θ21+zdz=J1−J2−J3+J4,(26)

where

J1=1+Hln⁡2∫0∞Θ11+zdz=∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1(−δSR)k1(1+H)ln⁡2k1!t!γ¯SRk1+1ΔSRk1−t+1Y(t,ΔSR,1),J2=1ln⁡2∫0∞Θ21+zdz=∑k1=0mSR−1∑t=0k1∑k=1∞∑p2k−1(2k−1p)B2k−1−p(−1)k+1αSR(1−mSR)k1(−δSR)k1Y(t+p,ΔSR,1)ln⁡2Ak−1/2−p(k−1)!(2k−1)2πaδ2γ¯RD2k1!t!γ¯SRk1+1ΔSRk1−t+1exp⁡(AC−B2A),J3=1+Hln⁡2∫0∞Θ1e(−zγ¯E)1+zdz=∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1e(zγ¯E)(1+H)ln⁡2k1!t!γ¯SRk1+1ΔSRk1−t+1(−δSR)−k1Y(t,ΔSR+1/γ¯E,1),J4=1ln⁡2∫0∞Θ2e(−zγ¯E)1+zdz=∑k1=0mSR−1∑t=0k1∑k=1∞∑p2k−1(2k−1p)B2k−1−p(−1)k+1αSR(1−mSR)k1(−δSR)k1exp⁡(B2−ACA)ln⁡2Ak−1/2−p(k−1)!(2k−1)2πaδ2γ¯RD2k1!t!γ¯SRk1+1ΔSRk1−t+1e(−zγ¯E)×Y(t+p,ΔSR+1/γ¯E,1),(27)

where

Y(n,u,b)=(−1)n−1bnebuEi(−bu)+∑p=1n(p−1)!(−b)n−pu−p.(28)

Then, the detailed expression for the ASC of the regarded secrecy system is given by

C¯S=∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1(−δSR)k1(1+H)ln⁡2k1!t!γ¯SRk1+1ΔSRk1−t+1Y(t,ΔSR,1)−∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1e(zγ¯E)(1+H)ln⁡2k1!t!γ¯SRk1+1ΔSRk1−t+1(−δSR)−k1Y(t,ΔSR+1/γ¯E,1)−∑k1=0mSR−1∑t=0k1∑k=1∞∑p2k−1(2k−1p)B2k−1−p(−1)k+1αSR(1−mSR)k1(−δSR)k1Y(t+p,ΔSR,1)ln⁡2Ak−1/2−p(k−1)!(2k−1)2πaδ2γ¯RD2k1!t!γ¯SRk1+1ΔSRk1−t+1exp⁡(AC−B2A)+∑k1=0mSR−1∑t=0k1∑k=1∞∑p2k−1(2k−1p)B2k−1−p(−1)k+1αSR(1−mSR)k1(−δSR)k1exp⁡(B2−ACA)ln⁡2Ak−1/2−p(k−1)!(2k−1)2πaδ2γ¯RD2k1!t!γ¯SRk1+1ΔSRk1−t+1e(−zγ¯E)×Y(t+p,ΔSR+1/γ¯E,1).(29)

3.3 Asymptotic ASC

In the following, the asymptotic analysis for the ASC will be given. When SNR becomes lager enough, namely, γ¯SR and γ¯RD become infinite, the CDF of γSR and γRD are respectively derived as

FSR(x)=αSRγ¯SRx+o(x),(30)

FγRD(x)=e−s22σ2x/2πσ2γ¯RD2+o(x),(31)

where o(x) represents the higher order of x.

Thus, by utilizing (8), (30) and (31), the CDF of γB will be given as

FγB(x)≃αSRγ¯SRx+e−s22σ2x2πσ2λ¯RD2+o(x).(32)

By replacing (22) with (32), then submitting (32) and (21) into (10), the asymptotic investigations for ASC can be derived. Unfortunately, we find it that we can not get the final expression by utilizing this method. So, referring to (28), from the fact that when γ¯ becomes larger enough, exp⁡(xγ¯)≈1+xγ¯ and Aγ¯+B≈B, namely, when u→0, (28) can be approximated as

Y∞(n,u,b)=Y(n,u,b)⏟u→0=(−1)n−1bn(1+bu)Ei(−bu)+∑p=1n(p−1)!(−b)n−pu−p.(33)

Then, by utilizing (29) and (33), the asymptotic expression will be given by

C¯S∞=∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1(−δSR)k1(1+H)ln⁡2k1!t!γ¯SRk1+1ΔSRk1−t+1Y∞(t,ΔSR,1)−∑k1=0mSR−1∑t=0k1αSR(1−mSR)k1e(zγ¯E)(1+H)ln⁡2k1!t!γ¯SRk1+1ΔSRk1−t+1(−δSR)−k1Y(t,1/γ¯E,1)−∑k1=0mSR−1∑t=0k1∑k=1∞∑p2k−1(2k−1p)B2k−1−p(−1)k+1αSR(1−mSR)k1(−δSR)k1Y∞(t+p,ΔSR,1)ln⁡2Ak−1/2−p(k−1)!(2k−1)2πaδ2γ¯RD2k1!t!γ¯SRk1+1ΔSRk1−t+1exp⁡(AC−B2A)+∑k1=0mSR−1∑t=0k1∑k=1∞∑p2k−1(2k−1p)B2k−1−p(−1)k+1αSR(1−mSR)k1(−δSR)k1exp⁡(B2−ACA)ln⁡2Ak−1/2−p(k−1)!(2k−1)2πaδ2γ¯RD2k1!t!γ¯SRk1+1ΔSRk1−t+1e(−zγ¯E)×Y(t+p,1/γ¯E,1).(34)

4  Numerical Results

In this section, some typical Monte Carlo (MC) simulations are presented to prove the efficiency of the investigation results. The effects of the key parameters are evaluated. With loss of no generality, in Figs. 2–5, γ¯SR=γ¯RD=γ¯, δR2=δD2=δE2=1. Besides, LRXi=LXiD=L=20 and B = 1. The channel parameters and system parameters are, respectively, given in Tables 2 and 3. Besides, in this paper, the infinite series function is utilized. For example, when N = 2, 10 terms are acceptable for deriving the proper results. However, when N increases to 5, 20 terms will be required for the simulations. Moreover, in this paper, we used the Matlab for the simulations.

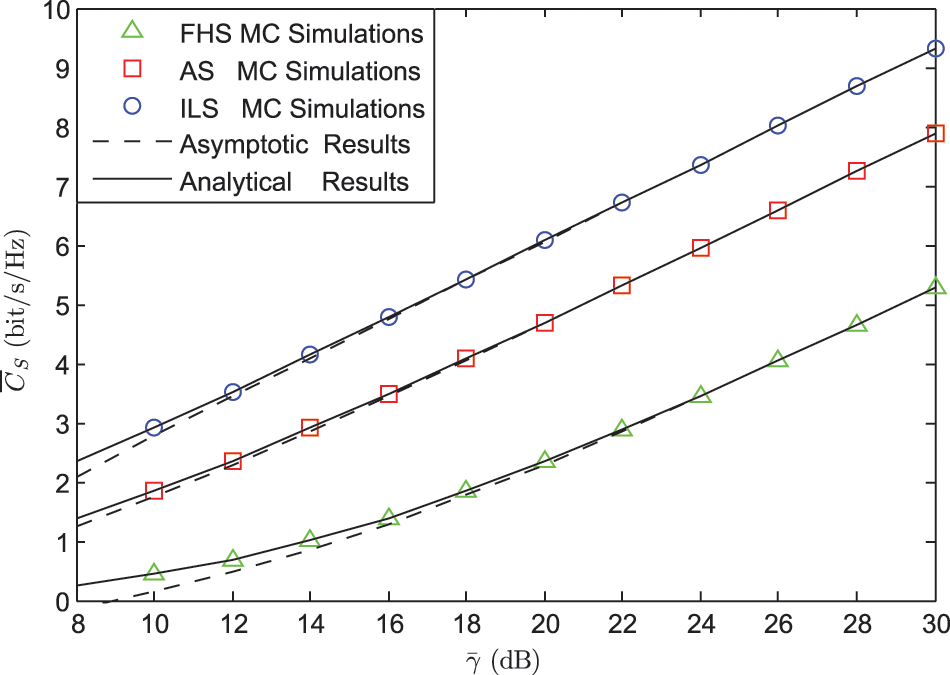
Figure 2: The ASC vs. different γ¯ for three channel fading and γ¯E = 0 dB with N=10

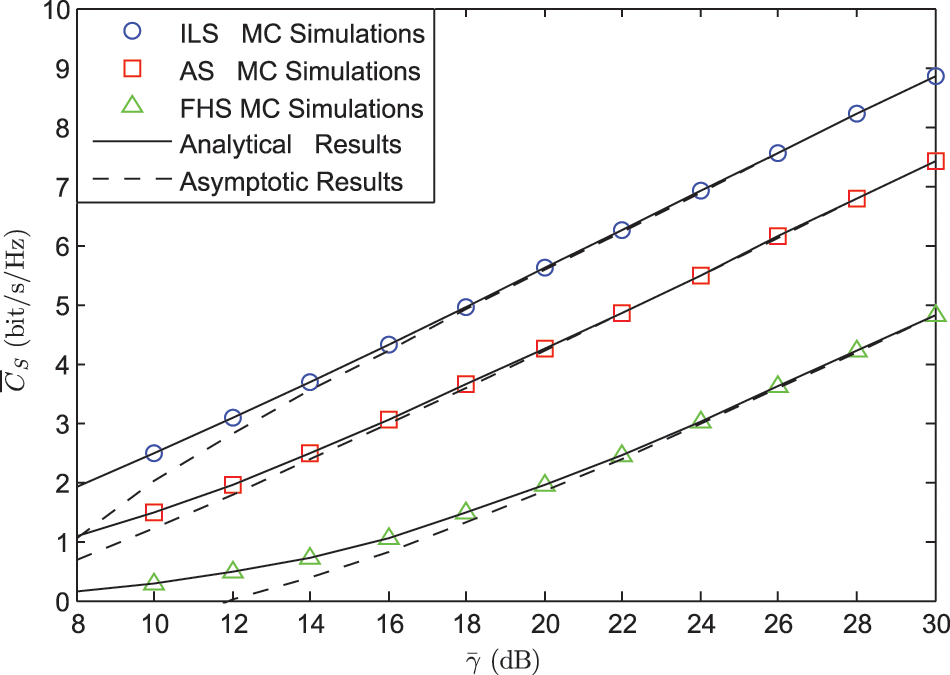
Figure 3: The ASC vs. different γ¯ for three channel fading and γ¯E = 3 dB with N=10

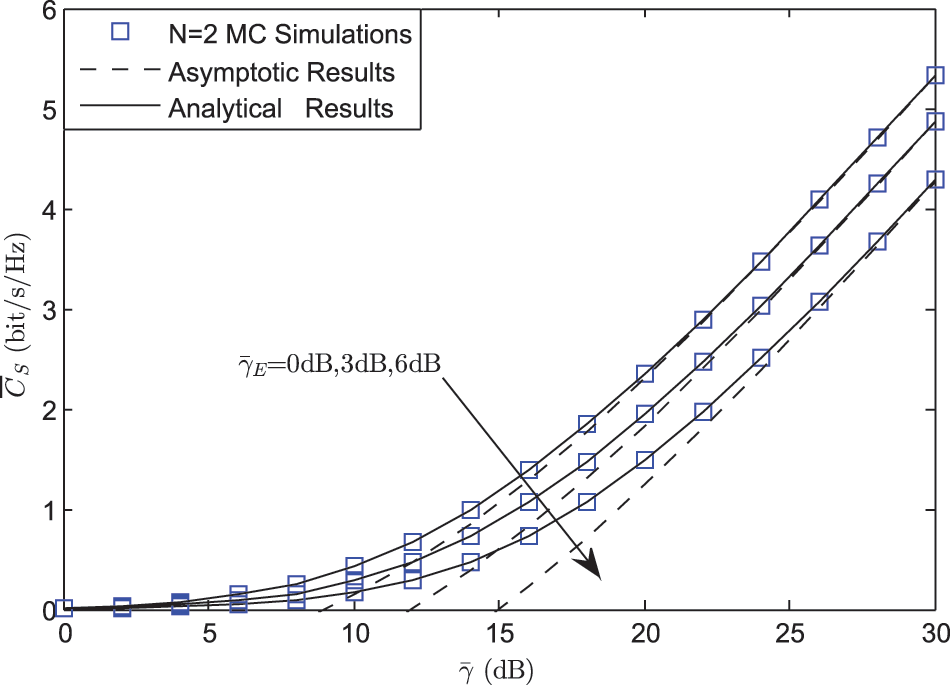
Figure 4: The ASC vs. several γ¯, several γ¯E and N = 2 for FHS scenario

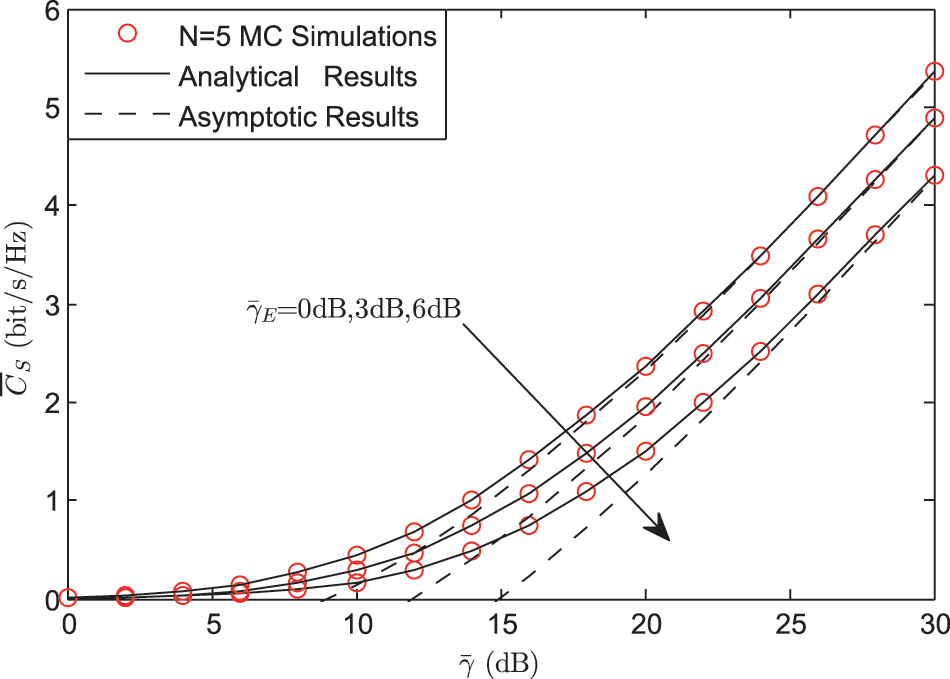
Figure 5: The ASC vs. several γ¯, several γ¯E and N = 5 for FHS scenario

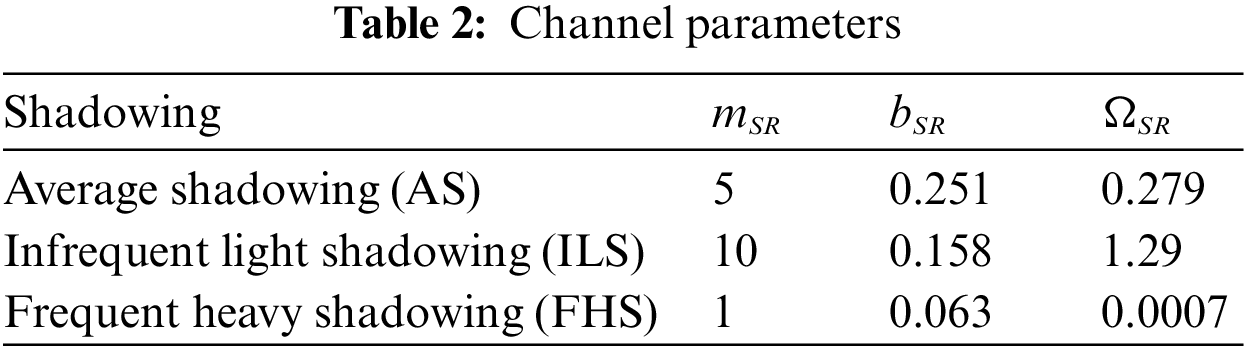
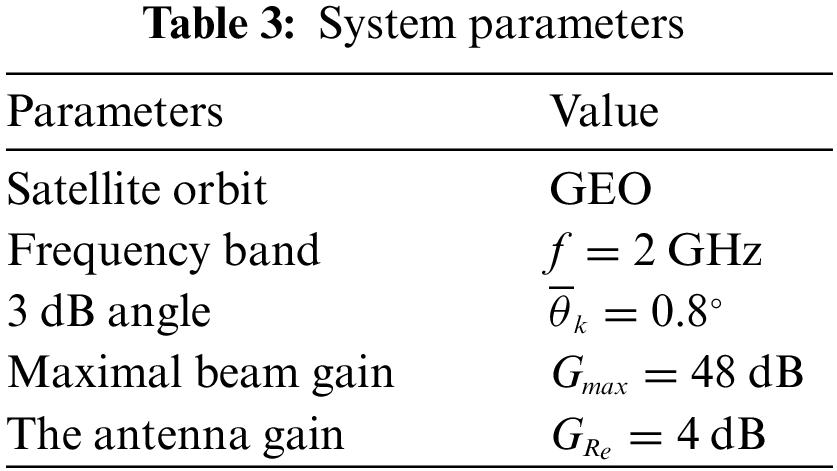
As proved in [34] and [36], a satellite channel model is expected to be general and applicable for a wide range of elevation angles, under which the satellite can be observed. In this regard, the most common approach to evaluate the impact of the elevation angle on channel statistical parameters is based on the transformation from empirical expression. According to [34], the maximum elevation angle is around 80, while the minimum elevation angle is considered to be around 20. In order to tackle the geographical terrain affects. Hence, this is particularly useful when applying a set of data parameters with moderate variation to a model with specific shadowing and infrequent light shadowing conditions (i.e., frequent heavy shadowing, average shadowing and infrequent light shadowing). Please note that for specific shadowing conditions, different parameters have been employed to cover a range of elevation angles in many existing works [17,19].

Fig. 2 plots the ASC vs. several γ¯ for three channel fading and γ¯E = 0 dB with N = 10. From Fig. 2, it can be got that the simulations are tight across the theoretical analysis, which implies the efficiency of the theoretical ones. Moreover, the approximate analysis matches with the simulations well in high SNR regime. Furthermore, we can find that the channel fading has a great impact on the ASC, i.e., the heavy channel fading brings the a lower ASC. In addition, we find that at high SNRs, the gap between the three curves are fixed, which will guide the engineering design.

Fig. 3 plots the ASC vs. several γ¯ for three channel fading and γ¯E = 3 dB with N = 10. From Fig. 3, it can be got that the simulations are tight across the theoretical analysis, which implies the efficiency of the theoretical ones. Moreover, the approximate analysis match with the simulations well in high SNR regime. Furthermore, we can find that the channel fading has a great impact on the ASC, namely the light channel fading brings the a larger ASC. When Fig. 2 is compared with Fig. 3, we can also find that when the power of the eavesdropper becomes larger, the ASC will have a lower value.

Fig. 4 illustrates the ASC vs. several γ¯, several γ¯E and N = 2 for FHS scenario. From Fig. 4, we also can see that the simulation results are the same with the theoretical results, which imply the correctness of our theoretical analysis. In addition, from this figure, we can find that when γ¯E becomes larger, the ASC will be smaller, for the reason that when the power of the eavesdroppers is smaller, the eve capacity will be degraded. At the same time, it can be observed that the curves are parallel at high SNRs, which is very interesting.

Fig. 5 examines the ASC vs. γ¯, several γ¯E and N = 5 for the FHS scenario. From Fig. 5, we can still find that the MC results are tight across the theoretical results, which also verify the rightness of the theoretical results. Besides, when compared with Fig. 4, it can be obtained that when N becomes larger, the ASC will be enhanced. However, we find that N has little impact on the ASC, for the reason that R→D link is not the decision link. The satellite transmission link is the decision link. Thus, there is a small gap for ASC between different N. In addition, Fig. 5 shows that the curves are still parallel in a high SNR regime, which is also very interesting.

5  Conclusions

In the section, the summary of this work was given. In this work, we researched the ASC for the RIS-based integrated satellite UAV relay networks. To enlarge the coverage area, the satellite was utilized. In order to enhance the transmission, the UAV was utilized to help the satellite’s transmission. Moreover, to save the energy, the RIS was utilized in a high building to enhance the transmission. Thus, the considered secrecy model was proper and acceptable. In particular, the final expressions were obtained for the ASC, and from the derived results, we could get the impacts of key parameters on the ASC. Especially for the asymptotic results, we could observe the following effects: the light channel fading, a lower γ¯E and a larger N would result in a larger ASC. Finally, several simulation results valuated the rightness of both the theoretical and asymptotic analyses.

Acknowledgement: The authors wish to express their appreciation to the reviewers for their helpful suggestions which greatly improved the presentation of this paper. The authors are grateful for the support by National Natural Science Foundation of China.

Funding Statement: This work was supported by the National Natural Science Foundation of China under Grants 62001517 and 61971474, in part supported by the Beijing Nova Program under Grant Z201100006820121.

Author Contributions: The authors confirm contribution to the paper as follows: study conception and design: Ping Li, Kefeng Guo and Feng Zhou; date collection: Ping Li, Kefeng Guo, and Xueling Wang; analysis and interpretation of results: Ping Li, Kefeng Guo, Feng Zhou, Xueling Wang and Yuzhen Huang; draft manuscript preparation: Ping Li, Kefeng Guo, and Feng Zhou. All authors reviewed the results and approved the final version of the manuscript.

Availability of Data and Materials: The raw/processed data required to reproduce the above findings cannot be shared at this time as the data also forms part of an ongoing study.

Conflicts of Interest: The authors declare that they have no conflicts of interest to report regarding the present study.

1Although in this paper, we just use only one UAV, the obtained results can also suit for the case with multiple UAVs.

2It is mentioned that, in this paper, each node owns only one antenna, while the remaining results are also suitable to the case that transmission nodes having multiple antennas when beamforming (BF) is utilized at the multiple antenna node.

3Owing to obstacles, fogs, rain attenuation, in this paper direct transmission link between the source and destination is not available, which will be considered in our future works.

4Although in this paper, we take the GEO satellite for an example, the obtained investigations can be also used to the scenario with medium Earth orbit (MEO) and low Earth orbit (LEO) satellites.

5TDMA scheme is used in the satellite to keep only one satellite beam and one UAV is used in each data transmission time slot. TDMA scheme is both adopted for the satellite downlink and uplink data transmission.

References

1. Ma, G., Dand, S., Alouini, M. S., Shihada, B. (2022). Smart buildings enabled by 6G communications. IEEE Internet of Things Magazine, 5(2), 33–52. [Google Scholar]

2. Guo, K., Lin, M., Zhang, B., Zhu, W. P., Wang, J. B. et al. (2019). On the performance of LMS communication with hardware impairments and interference. IEEE Transactions on Communications, 67(2), 1490–1505. [Google Scholar]

3. Peng, D., He, D., Li, Y., Wang, Z. (2022). Integrating terrestrial and satellite multibeam systems toward 6G: Techniques and challenges for interference mitigation. IEEE Wireless Communications, 29(1), 24–31. [Google Scholar]

4. Msadaa, I. C., Zairi, S., Dhraief, A. (2022). Non-terrestrial networks in a nutshell. IEEE Internet of Things Journal, 5(2), 168–174. [Google Scholar]

5. Giambene, G., Kota, S., Pillai, P. (2018). Satellite-5G integration: A network perspective. IEEE Network, 32(5), 25–31. [Google Scholar]

6. Lin, M., Huang, Q., Cola, T. D., Wang, J. B., Wang, J. et al. (2020). Integrated 5G-satellite networks: A perspective on physical layer relibility and security. IEEE Wireless Communications, 27(6), 152–159. [Google Scholar]

7. Li, X., Shi, W. (2022). Hybrid satellite-UAV-terrestrial maritime networks: Network selection for users on a vessel optimized with transmit power and UAV position. China Communications, 19(9), 37–46. [Google Scholar]

8. Wu, W., Zhou, F., Wang, B., Wu, Q., Dong, C. et al. (2022). Unmanned aerial vehicle swarm-enabled edge computing: Potentials, promising technologies, and challenges. IEEE Wireless Communications, 29(4),78–85. [Google Scholar]

9. Wu, W., Yang, F., Zhou, F., Wu, Q., Hu, R. Q. (2022). Intelligent resource allocation for IRS-enhanced OFDM communication systems: A hybrid deep reinforcement learning approach. IEEE Transactions on Wireless Communications, 22(6), 4028–4042. [Google Scholar]

10. Guo, K., Lin, M., Zhang, B., Wang, J. B., Wu, Y. et al. (2020). Performance analysis of hybrid satellite-terrestrial cooperative networks with relay selection. IEEE Transactions on Vehicular Technology, 69(8), 9053–9067. [Google Scholar]

11. Guo, K., An, K., Zhang, B., Huang, Y., Guo, D. et al. (2019). On the performance of the uplink satellite multi-terrestrial relay networks with hardware impairments and interference. IEEE Systems Journal, 13(3), 2297–2308. [Google Scholar]

12. Upadhyay, P. K., Sharma, P. K. (2016). Max-max user-relay selection scheme in multiuser and multirelay hybrid satellite-terrestrial relay systems. IEEE Communications Letters, 20(2), 268–271. [Google Scholar]

13. An, K., Lin, M., Ouyang, J., Huang, Y., Zheng, G. (2014). Symbol error analysis of hybrid satellite-terresrial cooperative networks with cochannel interference. IEEE Communications Letters, 18(11), 1947–1950. [Google Scholar]

14. Li, X., Zheng, Y., Khan, W. U., Zeng, M., Li, D. et al. (2021). Physical layer security of cognitive ambient backscatter communications for green Internet-of-Things. IEEE Transactions on Green Communications and Networking, 5(2), 1066–1076. [Google Scholar]

15. Li, B., Fei, Z., Zhou, C., Zhang, Y. (2020). Physical layer security in space information networks: A survey. IEEE Internet of Things Journal, 69(5), 33–52. [Google Scholar]

16. An, K., Lin, M., Ouyang, J., Zhu, W. P. (2016). Secure transmission in cognitive satellite terrestrial networks. IEEE Journal on Selected Areas in Communications, 34(11), 3025–3037. [Google Scholar]

17. Guo, K., An, K., Zhang, B., Huang, Y., Tang, X. et al. (2020). Physical layer security for multiuser satellite communication systems with threshold-based scheduling scheme. IEEE Transactions on Vehicular Technology, 69(5), 5129–5141. [Google Scholar]

18. Guo, K., Li, X., Alazab, M., Jhaveri, R. H., An, K. (2022). Integrated satellite multiple two-way relay networks: Secrecy performance under multiple eves and vehicles with non-ideal hardware. IEEE Transactions on Intelligent Vehicles, 8(2), 1307–1318. [Google Scholar]

19. Guo, K., Dong, C., An, L. (2022). NOMA-based cognitive satellite terrestrial relay network: Secrecy performance under channel estimation errors and hardware impairments. IEEE Internet of Things Journal, 9(18), 17334–17347. [Google Scholar]

20. Guo, K., An, K., Zhang, B., Guo, D. (2018). Physical layer security for hybrid satellite terrestrial relay networks with joint relay selection and user scheduling. IEEE Access, 6, 55815–55827. [Google Scholar]

21. Lin, Z., Lin, M., Champagne, B., Zhu, W. P., Dhahir, N. A. (2021). Secrecy-energy efficient hybrid beamforming for satellite-terrestrial integrated networks. IEEE Transactions on Communications, 69(3), 6345–6360. [Google Scholar]

22. Lin, Z., An, K., Niu, H., Hu, Y., Chatzinotas, S. et al. (2022). SLNR-based secure energy efficient beamforming in multibeam satellite systems. IEEE Transactions on Aerospace and Electronic Systems, 59(2), 2085–2088. [Google Scholar]

23. Chen, Z. N., Qing, X., Tang, X., Liu, W. E. I., Xu, R. et al. (2022). Phased array metantennas for satellite communications. IEEE Communications Magazine, 60(1), 46–50. [Google Scholar]

24. Huang, C., Zappone, A., Alexandropoulos, G. C., Debbah, M., Yuen, C. (2019). Reconfigurable intelligent surfaces for energy efficiency in wireless communication. IEEE Transactions on Wireless Communications, 18(8), 4157–4170. [Google Scholar]

25. Di, B., Zhang, H., Song, L., Li, Y., Han, Z. et al. (2020). Hybrid beamforming for reconfigurable intelligent surface based multi-user communications: Achievable rates with limited discrete phase shifts. IEEE Journal on Selected Areas in Communications, 38(8), 1809–1822. [Google Scholar]

26. Su, Y., Pang, X., Chen, S., Jiang, X., Zhao, N. et al. (2022). Spectrum and energy efficiency optimization in IRS-assisted UAV networks. IEEE Transactions on Communications, 70(10), 6489–6502. [Google Scholar]

27. Yang, L., Meng, F., Zhang, J., Hasna, M. O., Renzo, M. D. (2020). On the performance of RIS-assisted dual-hop UAV communication systems. IEEE Transactions on Vehicular Technology, 69(9), 10385–10390. [Google Scholar]

28. Khalili, A., Malarvizhi, S. (2022). Resource management for transmit power minimization in UAV-assisted RIS HetNets supported by dual connectivity. IEEE Transactions Wireless Communications, 21(3), 1806–1822. [Google Scholar]

29. Nguyen, T. N., Le, H. D., Pham, A. T. (2022). On the design of RIS-UAV relay-assisted hybrid FSO/RF satellite-aerial-ground integrated network. IEEE Transactions on Aerospace Electronic Systems, Early Access, 3, 1–15. [Google Scholar]

30. Guo, K., An, K. (2022). On the performance of RIS-assisted integrated satellite-UAV-terrestrial networks with hardware impairments and interference. IEEE Wireless Communications Letters, 11(1), 131–135. [Google Scholar]

31. Lin, Z., Niu, H., An, K., Wang, Y., Zheng, G. et al. (2022). Refracting RIS-aided hybrid satellite-terrestrial relay networks: Joint beamforming design and optimization. IEEE Transactions on Aerospace and Electronic Systems, 58(4), 3717–3724. [Google Scholar]

32. Guo, K., Liu, R., Dong, C., An, K., Huang, Y. et al. (2023). Ergodic capacity of NOMA-based overlay cognitive integrated satellite-UAV-terrestrial networks. Chinese Journal of Electronics, 32(2), 273–282. [Google Scholar]

33. Zhou, F., Li, X., Alazab, M., Jhaveri, R. H., Guo, K. (2022). Secrecy performance for RIS-based integrated satellite vehicle networks with a UAV relay and MRC eavesdropping. IEEE Transactions on Intelligent Vehicles, 8(2), 1676–1685. [Google Scholar]

34. Abdi, A., Lau, W. C., M., S., Kaveh, M. (2003). A new simple model for land mobile satellite channels: First-and second-order statistics. IEEE Transactions on Wireless Communications, 2(3), 519–528. [Google Scholar]

35. Gradshteyn, I. S., Ryzhik, I. M. (2007). Table of integrals, series and products. Boston, USA: Academic Press. [Google Scholar]

36. Arti, M. K., Suresh, K. J. (2016). OSTBC transmission in shadowed-Rician land mobile satellite links. IEEE Transactions on Vehicular Technology, 65(7), 5771–5777. [Google Scholar]