Open Access

ARTICLE

An Interpolation Method for Karhunen–Loève Expansion of Random Field Discretization

Zi Han^1,*, Zhentian Huang²

1 Department of Engineering Mechanics, Hohai University, Nanjing, 211100, China
2 College of Civil and Transportation Engineering, Shenzhen University, Guangdong Provincial Key Laboratory of Deep Earth Sciences and Geothermal Energy Exploitation and Utilization, Institute of Deep Earth Sciences and Green Energy, Shenzhen, China

* Corresponding Author: Zi Han. Email:

Computer Modeling in Engineering & Sciences 2024, 138(1), 245-272. https://doi.org/10.32604/cmes.2023.029708

Received 04 March 2023; Accepted 26 April 2023; Issue published 22 September 2023

Abstract

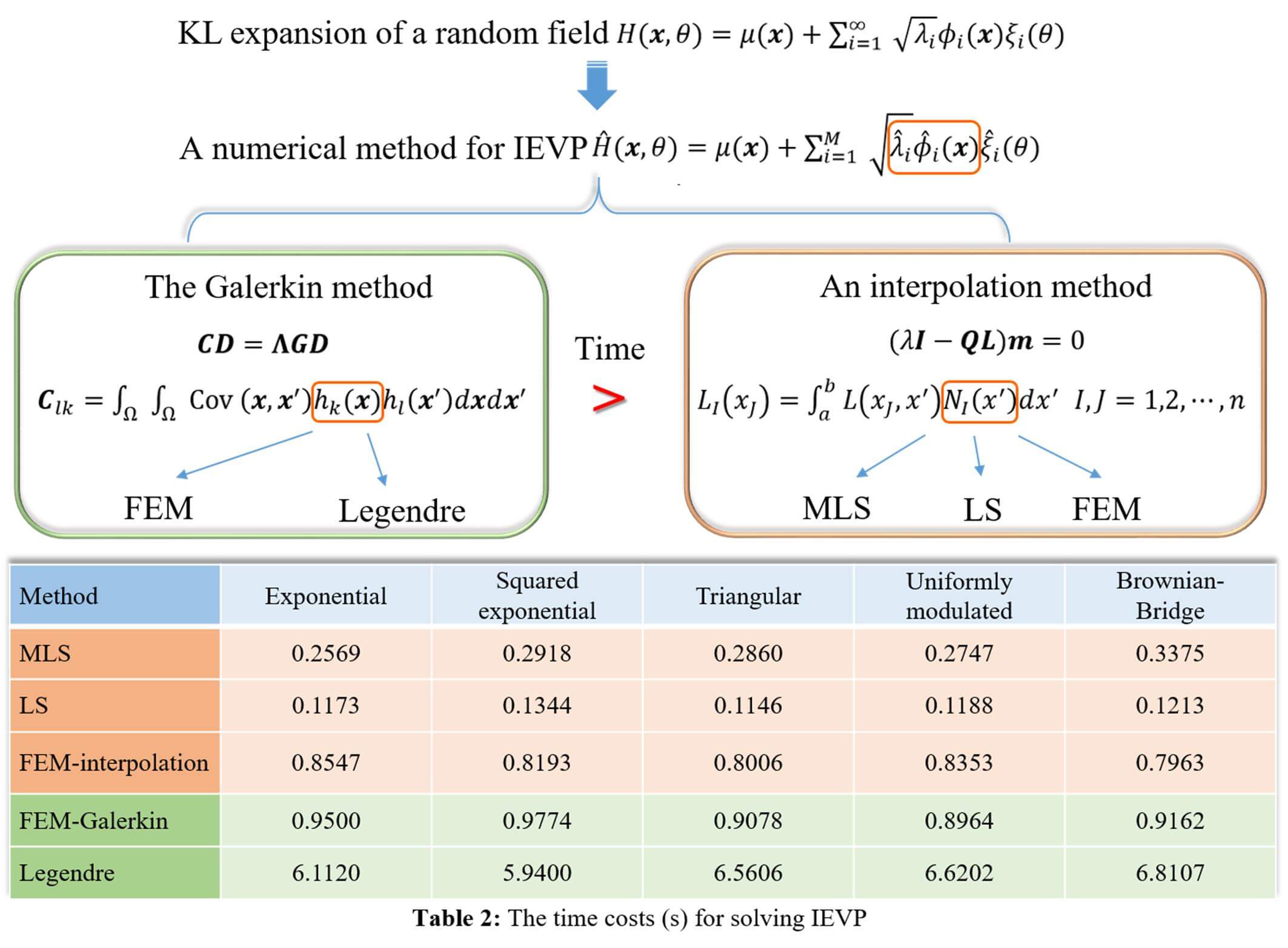
In the context of global mean square error concerning the number of random variables in the representation, the Karhunen–Loève (KL) expansion is the optimal series expansion method for random field discretization. The computational efficiency and accuracy of the KL expansion are contingent upon the accurate resolution of the Fredholm integral eigenvalue problem (IEVP). The paper proposes an interpolation method based on different interpolation basis functions such as moving least squares (MLS), least squares (LS), and finite element method (FEM) to solve the IEVP. Compared with the Galerkin method based on finite element or Legendre polynomials, the main advantage of the interpolation method is that, in the calculation of eigenvalues and eigenfunctions in one-dimensional random fields, the integral matrix containing covariance function only requires a single integral, which is less than a two-folded integral by the Galerkin method. The effectiveness and computational efficiency of the proposed interpolation method are verified through various one-dimensional examples. Furthermore, based on the KL expansion and polynomial chaos expansion, the stochastic analysis of two-dimensional regular and irregular domains is conducted, and the basis function of the extended finite element method (XFEM) is introduced as the interpolation basis function in two-dimensional irregular domains to solve the IEVP.

---

Graphic Abstract

---

Keywords

---