Open Access

ARTICLE

Geometric Morphometrics Applied to Cartography

Frédéric Roulier^*

UMR CNRS 7058 Écologie et Dynamique des Systèmes Anthropisés’ (EDYSAN), Université de Picardie Jules Verne, Amiens, France

* Corresponding Author: Frédéric Roulier. Email:

Revue Internationale de Géomatique 2023, 32, 17-37. https://doi.org/10.32604/RIG.2023.045458

Received 27 August 2023; Accepted 19 October 2023; Issue published 12 December 2023

Abstract

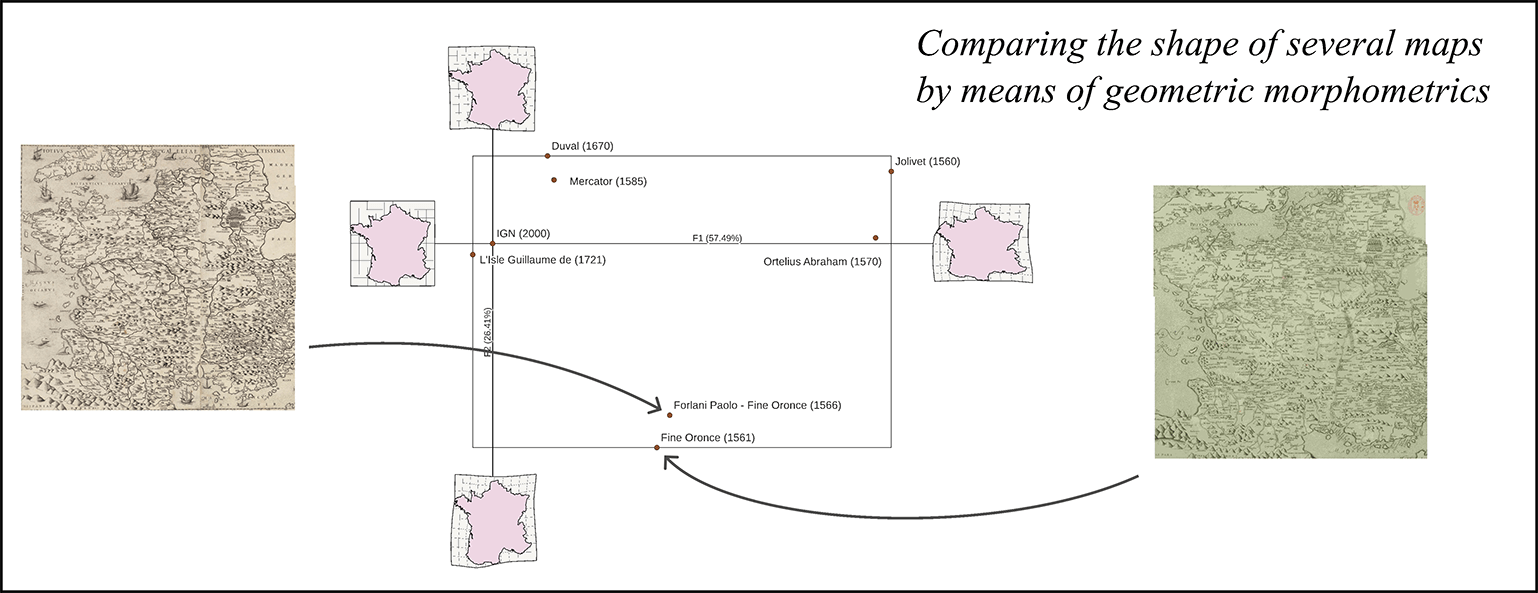
The morphological differences between two geographical maps can be highlighted by a polycentric distance cartogram resulting from a bidimensional regression. Beyond the communicational interest of the transformations thus produced, the method makes it possible to reveal the differences in structure and therefore constitutes a real research tool. However, bidimensional regression can only compare the shape of two maps. Since the 1990s, geometric morphometrics has revolutionized the morphological analysis of natural structures (and others). It has since been applied in many fields of research but not in cartography. This article describes the theoretical and methodological bases of a method combining bidimensional regression with a geometric morphometrics approach to compare the shape of several geographical maps. Geometric morphometrics and bidimensional regression indeed share common approaches of the statistical shape analysis like homologous landmarks and interpolation grids. However, there is no software in geometric morphometrics capable of directly reading geographical data, which would facilitate the work of cartographers accustomed to GIS software. That is why we present MapMorphy, a tool specifically developed for this task. An example on ancient maps illustrates the method.

---

Graphic Abstract

---

Keywords

---